What is the measure of a right angle?
45 degrees
60 degrees
90 degrees
120 degrees

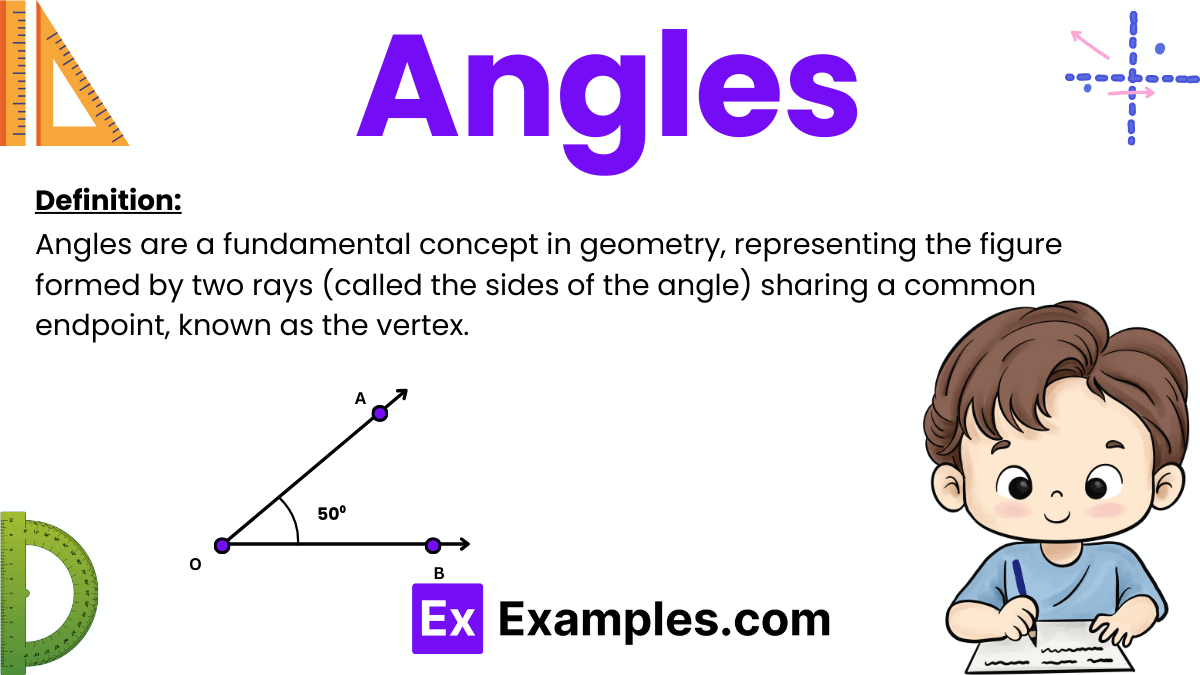
Angles are a fundamental concept in geometry, representing the figure formed by two rays (called the sides of the angle) sharing a common endpoint, known as the vertex. They are typically measured in degrees or radians and describe the amount of turn between each arm. Angles are used to describe the shape, size, and direction of corners found throughout daily life and various fields such as mathematics, engineering, and architecture.
An angle primarily consists of two essential parts:
Arms: These are the two rays that extend from a common point and form the angle. The arms can be of any length and direction but share the vertex as their starting point.

Vertex: This is the specific point at which the two arms meet. It acts as the pivotal point from which the angle is measured.
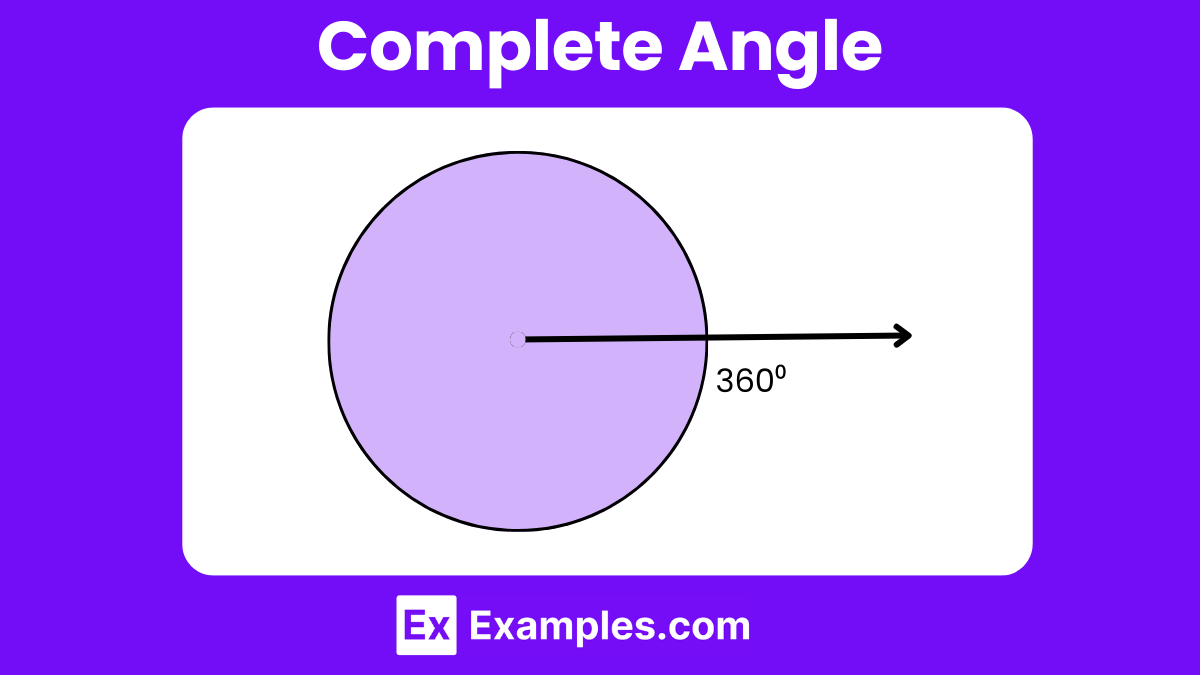
An angle is measured in degree, with a full rotation around a point forming a complete angle of 360°.
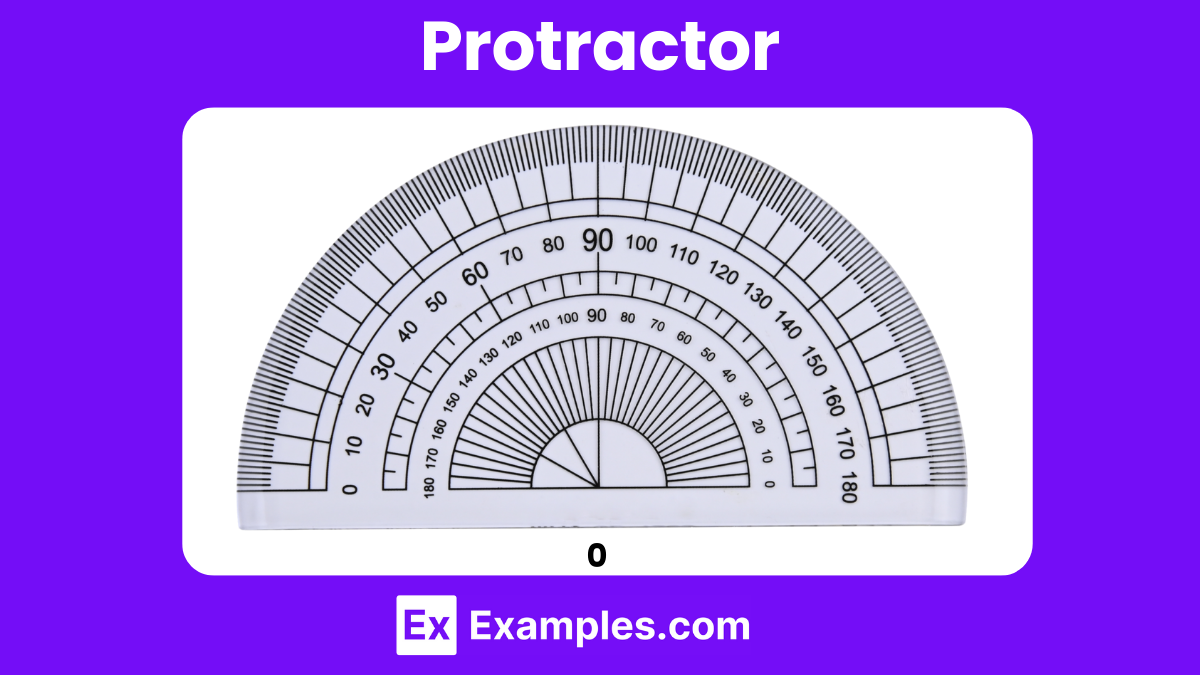
The most effective tool for measuring an angle is a protractor, which is typically a semi-circular, translucent instrument. It facilitates precise measurement of angles in degrees. A protractor is marked with degrees from 0° to 180° on both the outer and inner scales. The outer scale measures degrees clockwise, while the inner scale measures them counterclockwise. This dual scale system allows for easy and accurate reading of both acute and obtuse angles, making the protractor indispensable in geometry and various practical applications.
Angles are categorized based on their measure, which defines their unique characteristics. Let’s explore each type of angle and its properties:
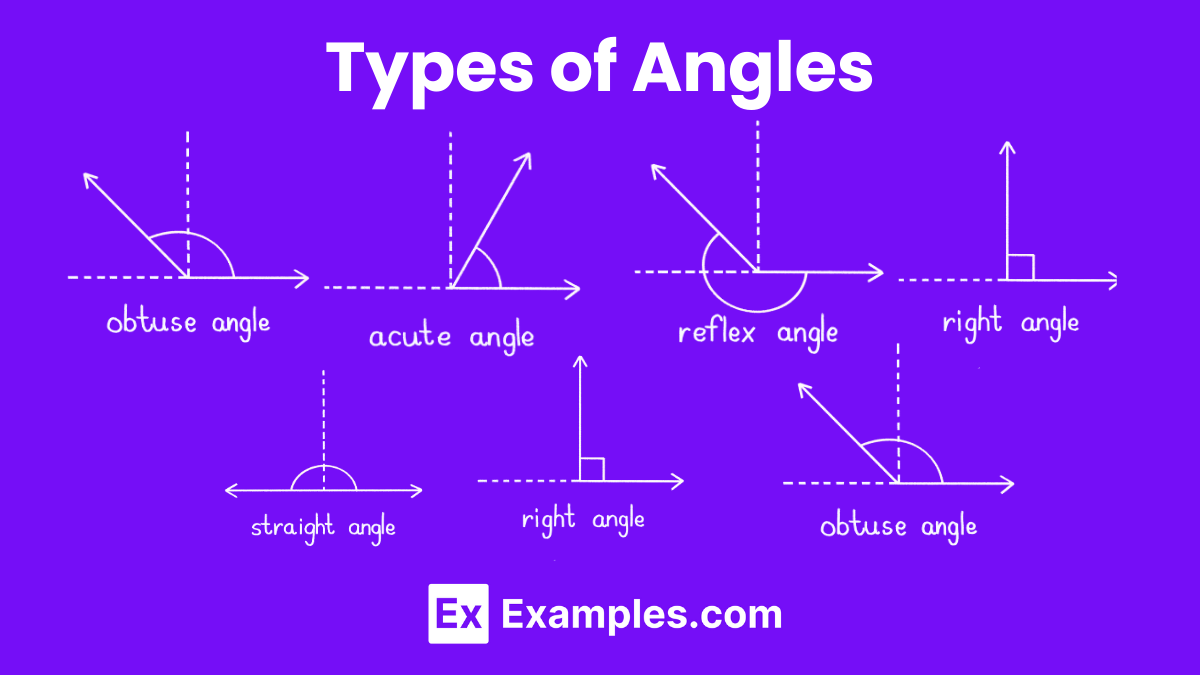
An acute angle measures greater than 0° but less than 90°. These angles are sharp and are often seen in various geometric figures.
A right angle measures exactly 90°, resembling the shape of the letter L. This type of angle is prevalent in everyday objects and represents a quarter turn.
An obtuse angle is one that measures more than 90° but less than 180°. These angles appear wider and are less common than acute angles.
A straight angle measures exactly 180°, forming a straight line. This angle represents a half turn, consisting of two right angles back-to-back.
A reflex angle measures more than 180° but less than 360°. These angles are larger than a straight angle and span more than half a turn.
A complete angle measures exactly 360°, representing a full rotation around a point.
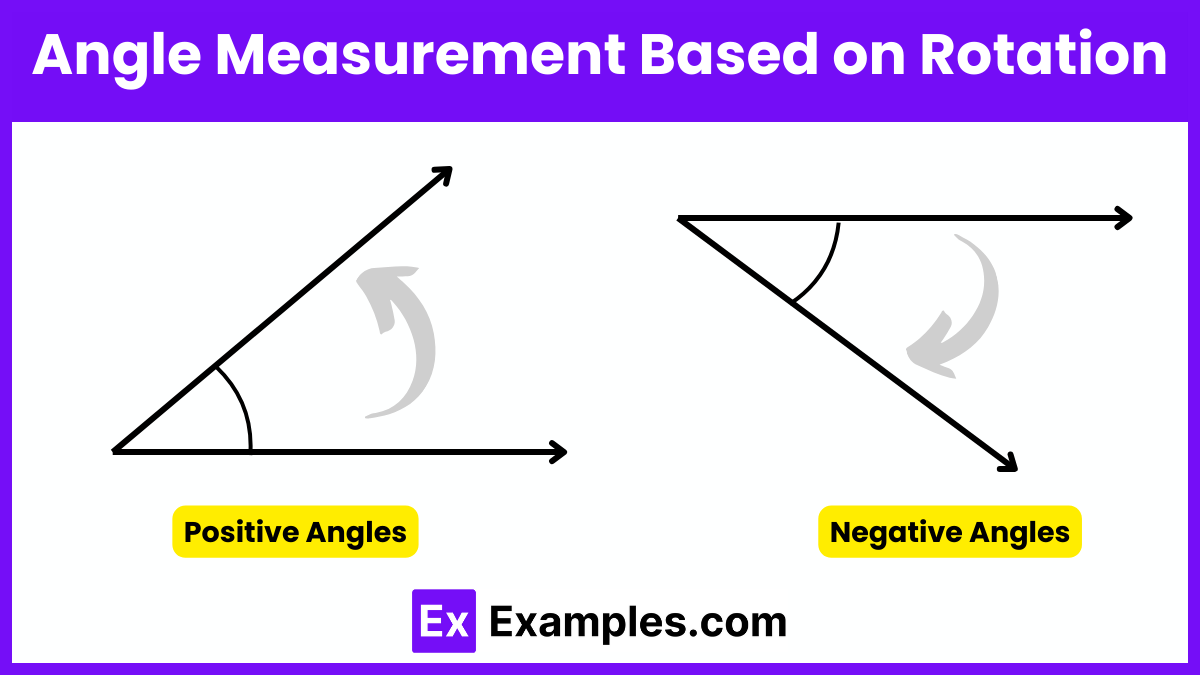
Angles can also be classified based on the direction of their rotation:
Positive angles are measured in a counterclockwise direction from the base. This is the standard direction for measuring angles in mathematics.
Negative angles are measured in a clockwise direction from the base. These angles represent a reverse rotation compared to the typical angle measurement.
We use protractors to accurately measure angles. Consider the angle ∠AOB depicted in the figure below. At first glance, ∠AOB appears to be an acute angle, indicating that its measurement is greater than 0° and less than 90°. Let’s explore how to measure this angle using a protractor effectively.
Let us try to measure the given ∠AOB.
Step 1: Position the protractor so that the ray OB aligns with the baseline of the protractor. Begin by observing the inner scale, starting from the 0° mark located at the bottom right of the protractor. This alignment ensures accurate measurement of the angle.
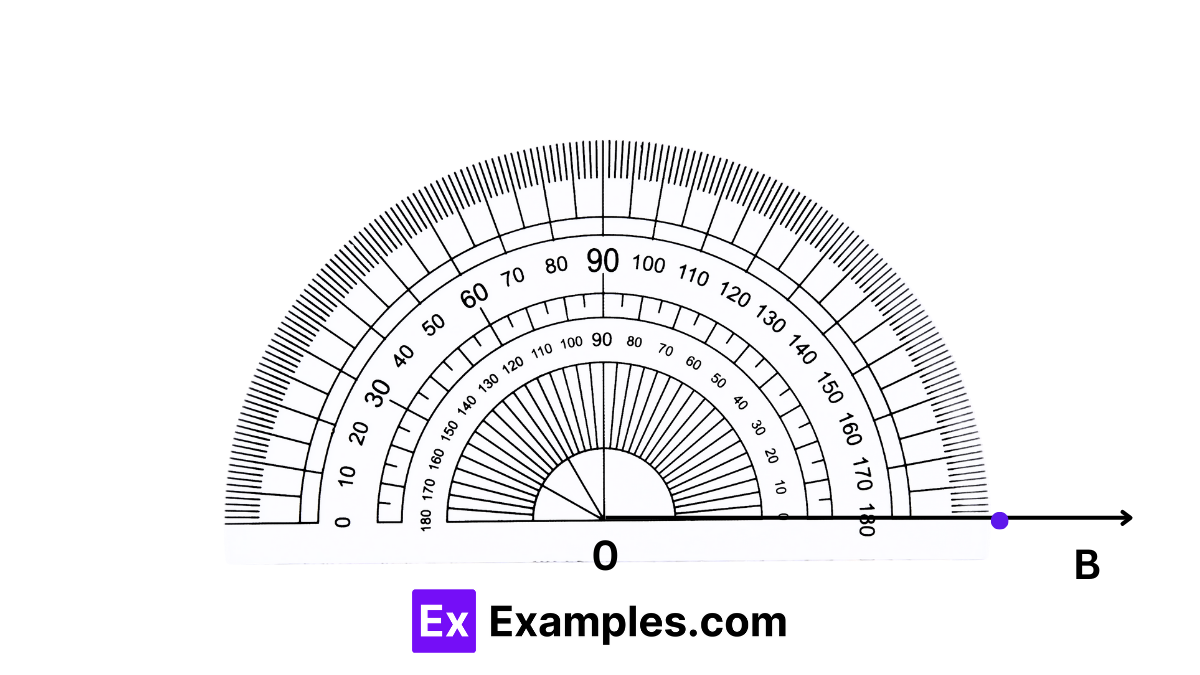
Step 2: Identify the number on the protractor that aligns with the second ray to determine the angle’s measurement. Use the protractor’s inner scale for this measurement. Consequently, the angle ∠AOB measures 37°.

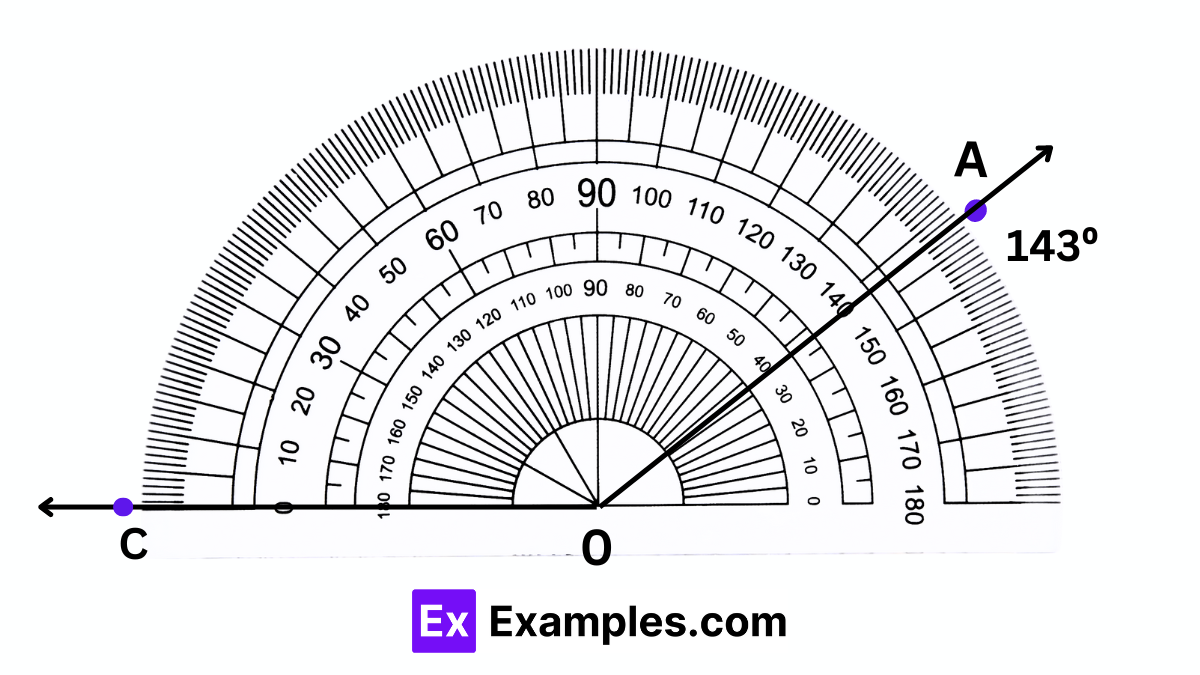
Now, let us try to measure the given ∠AOC.
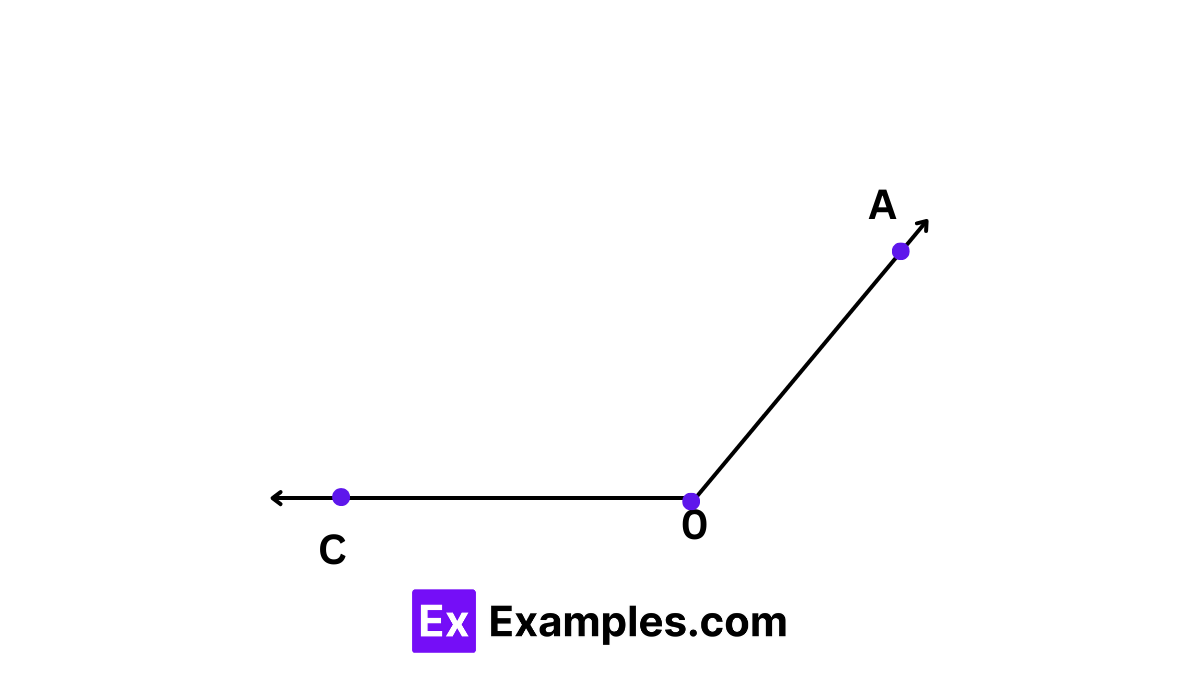
Step 1: To measure the angle, use the outer scale of the protractor, starting from the 0° mark located at the bottom left.
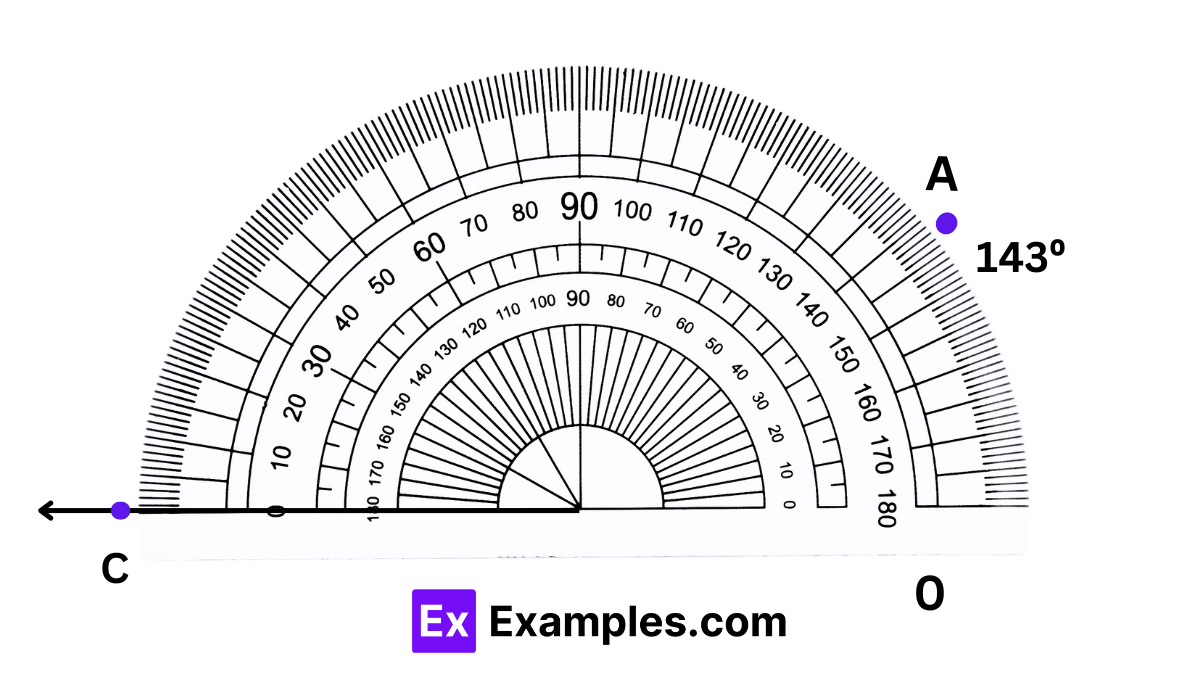
Step 2: The number on the outer scale of the protractor that aligns with ray OA indicates the measurement of ∠AOC. Therefore, ∠AOC measures 143°.
Constructing angles accurately is essential in various fields such as drafting, architecture, and even school projects. Here’s a step-by-step guide on how to draw a 50° angle using a protractor:
Step 1: First, draw a straight line, labeling it as ray OB. Position the protractor so that its baseline aligns perfectly with ray OB, ensuring the center point of the protractor is at the endpoint of the ray, typically marked as O.
Step 2: Using the inner scale of the protractor, mark a point A above the marking on the protractor that corresponds to 50°.
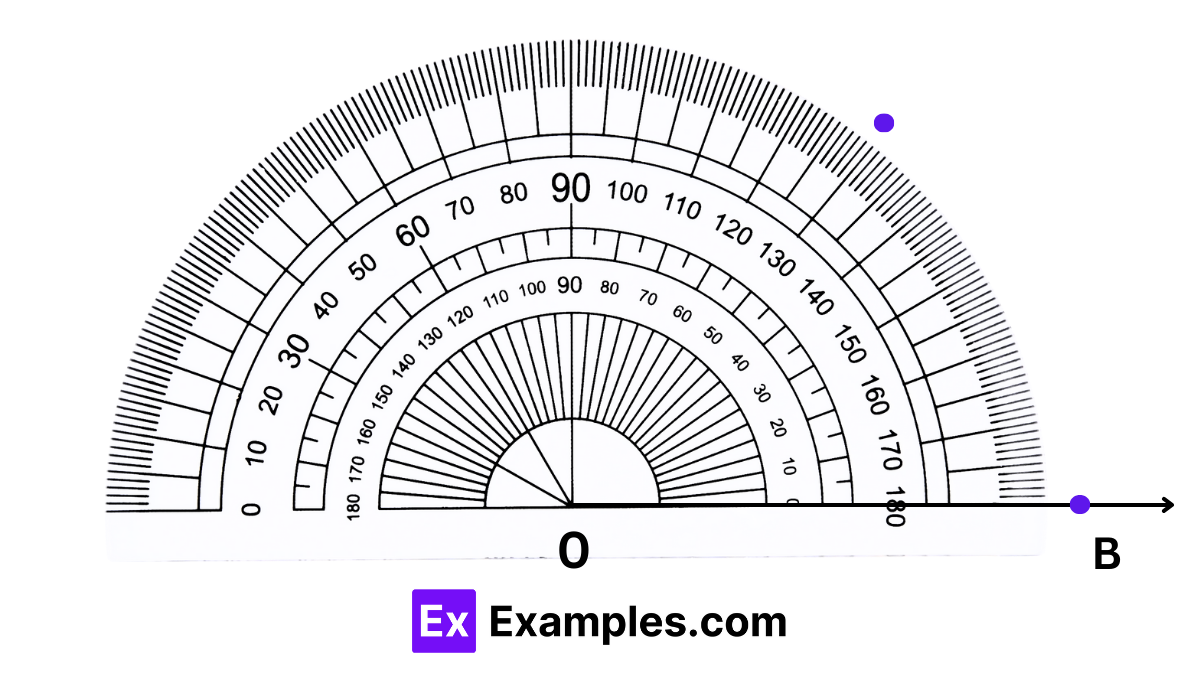
Step 3: After removing the protractor, draw a ray starting at point O and extending through point A. This creates the desired angle, ∠AOB, which measures 50°.
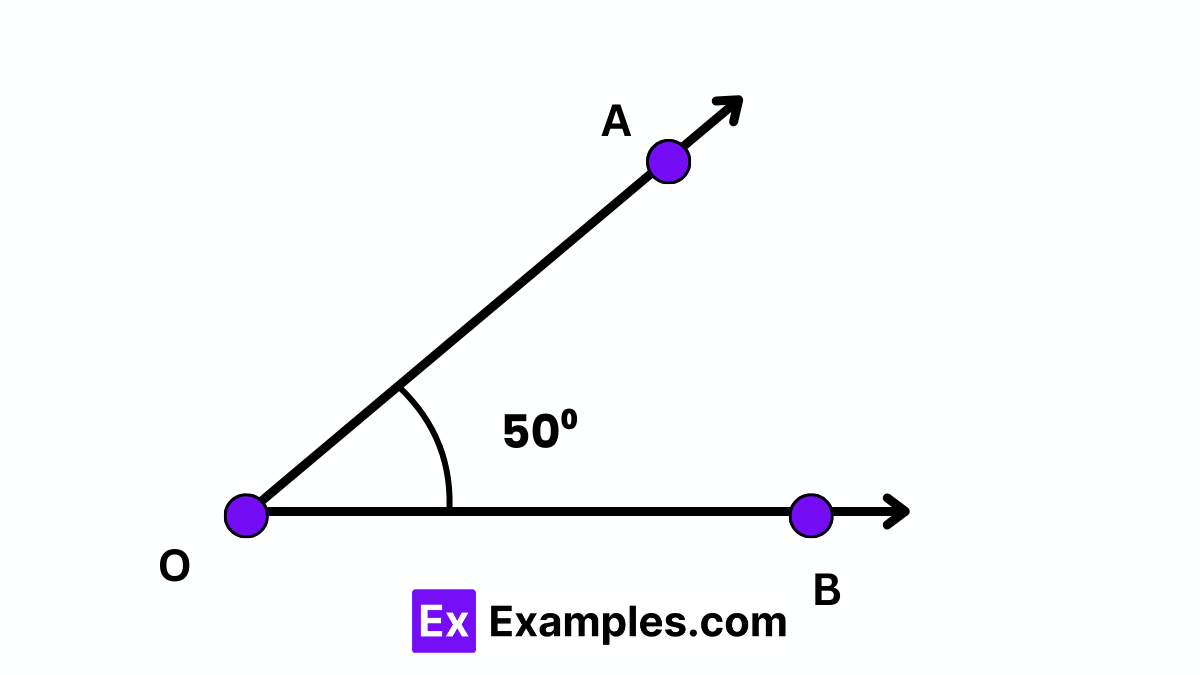
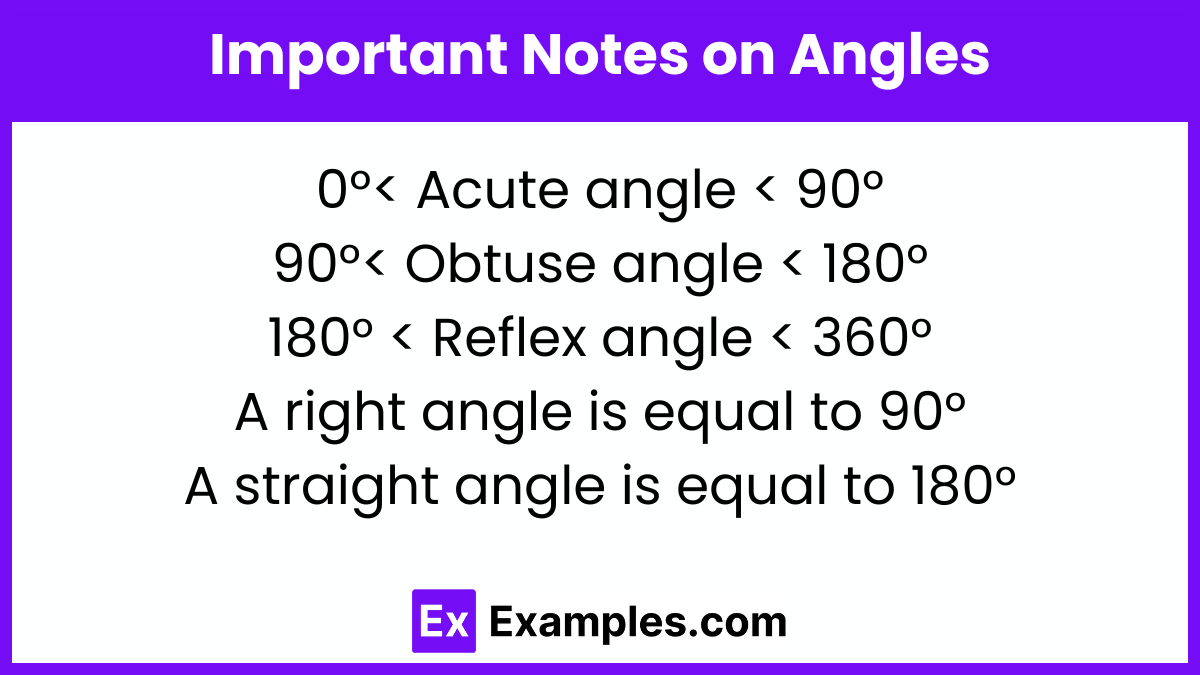
Here are some crucial points to understand about angles, which are essential for grasping their properties and applications in geometry:
Draw the following angles and identify them as acute, obtuse, right, straight, reflex, or complete:
Using a protractor, measure and draw the following angles:
Calculate the missing angle in each scenario:
Identify the type of angle formed in the following real-life situations:
Create a diagram that includes the following:
Question: Identify the type of each angle:
Solution:
Question: A triangle has two angles measuring 45° and 70°. What is the measure of the third angle?
Solution: The sum of the angles in a triangle is always 180°. Therefore: Third Angle=180°−(45°+70°)=180°−115°=65°Third Angle=180°−(45°+70°)=180°−115°=65° The third angle measures 65°.
Question: If one angle measures 65°, find its complementary and supplementary angles.
Solution:
Question: Two intersecting lines form four angles. If one of the angles is 110°, what are the measures of the other three angles?
Solution:
An angle is a geometric figure formed by two rays sharing a common endpoint called the vertex. It measures the rotation required to align one ray with the other.
The angle refers to the space between two intersecting lines or surfaces at or close to the point where they meet, usually measured in degrees.
The seven types of angles are acute, right, obtuse, straight, reflex, complete, and perigon angles, each defined by its unique range of measurements.
In slang, “angle” refers to a calculated approach or perspective, often used to imply someone has a specific motive or is looking for a particular advantage in a situation.
“Angel” refers to a spiritual being believed to act as a messenger of God, typically depicted with wings. “Angle” is a mathematical term describing the space between two intersecting lines or surfaces.
For kids, an angle can be described as a shape made when two lines meet at a point. You can think of it as the corner of a piece of paper or a book.
Explain angles to a child by demonstrating how two sticks or pencils can form a space or opening when they meet at one end. The size of the opening helps us understand how big or small the angle is.
Every angle is identified based on its measurement: acute (less than 90°), right (exactly 90°), obtuse (between 90° and 180°), straight (180°), reflex (between 180° and 360°), and complete (360°).
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the measure of a right angle?
45 degrees
60 degrees
90 degrees
120 degrees
What is the sum of the interior angles of a triangle?
90 degrees
180 degrees
270 degrees
360 degrees
If one angle of a triangle is 50 degrees and another is 60 degrees, what is the measure of the third angle?
60 degrees
70 degrees
80 degrees
90 degrees
What is the measure of each angle in an equilateral triangle?
45 degrees
60 degrees
75 degrees
90 degrees
What type of angle measures more than 90 degrees but less than 180 degrees?
Acute angle
Right angle
Obtuse angle
Reflex angle
What is the measure of each angle in a square?
90 degrees
60 degrees
45 degrees
120 degrees
What is the measure of a straight angle?
90 degrees
180 degrees
270 degrees
360 degrees
What is the sum of the exterior angles of a polygon?
180 degrees
360 degrees
540 degrees
720 degrees
What is the measure of an angle supplementary to a 110-degree angle?
70 degrees
80 degrees
90 degrees
100 degrees
What type of angle measures exactly 180 degrees?
Acute angle
Right angle
Straight angle
Obtuse angle
Before you leave, take our quick quiz to enhance your learning!

