Area of Rectangle

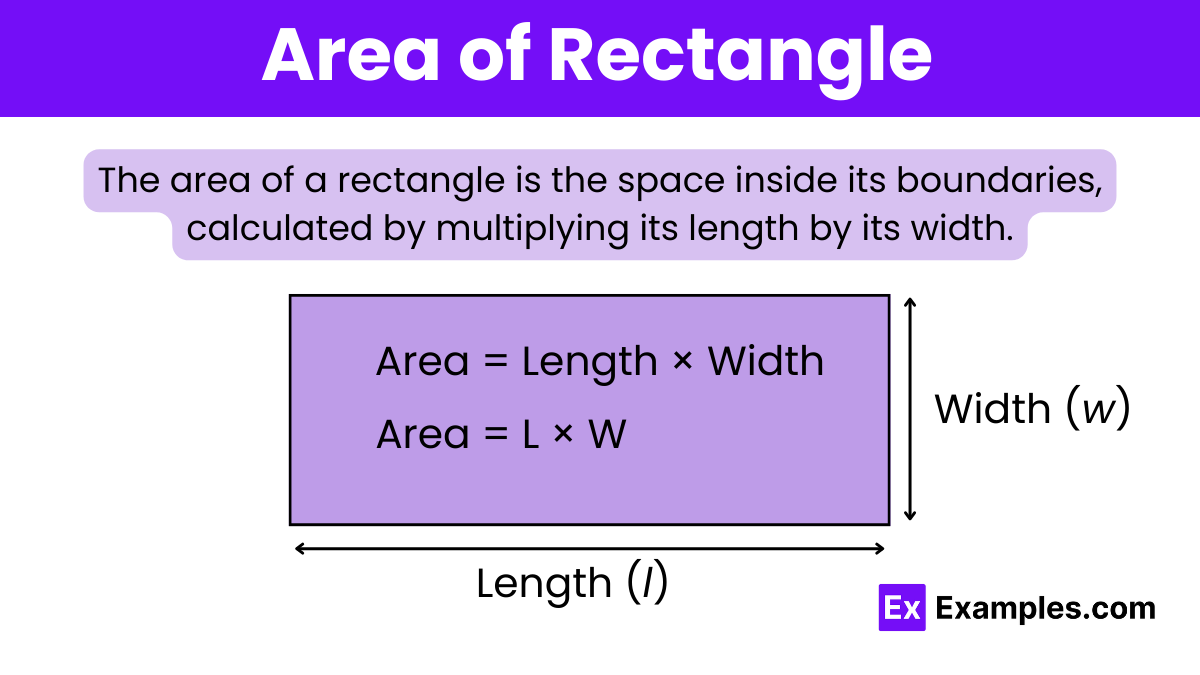
The area of a rectangle is the space inside its four sides. It tells us how much surface the rectangle covers. You can figure out this area with a special formula, depending on the rectangle’s length and width. In this lesson, we’ll learn how to calculate the area of a rectangle using different methods based on the measurements we have.

What is Area of Rectangle?

The area of a rectangle is the measure of the space enclosed within its four sides. It tells you how much surface area the rectangle occupies. To calculate the area, you multiply the length of the rectangle by its width. The result is expressed in square units, such as square meters, square feet, or square inches, depending on the units used for the length and width. This calculation helps determine the size of flat surfaces, like tabletops or floors.

Area of Rectangle Formula

The formula to calculate the area of a rectangle is straightforward: multiply the length of the rectangle by its width. This is expressed mathematically as:

Area = Length × Width

This formula provides the area in square units, based on the units used for length and width, whether they are in meters, feet, or another measure. This calculation is essential for determining how much space a rectangle covers, useful in various practical scenarios like flooring a room or covering a wall with paint.

How to Find Area of Rectangle?

1. Measure the Length: Determine the length of the rectangle, which is one of the longer sides.
2. Measure the Width: Determine the width, which is one of the shorter sides perpendicular to the length.
3. Use the Formula: Multiply the length by the width to find the area. The formula is:
   • Area = Length × Width
4. Calculate: Perform the multiplication to get the area. Ensure you use the same units for both length and width to get the area in square units (like square meters, square feet, etc.).
5. Result: The result from the multiplication gives you the area of the rectangle, telling you how much surface the rectangle covers.

Example:

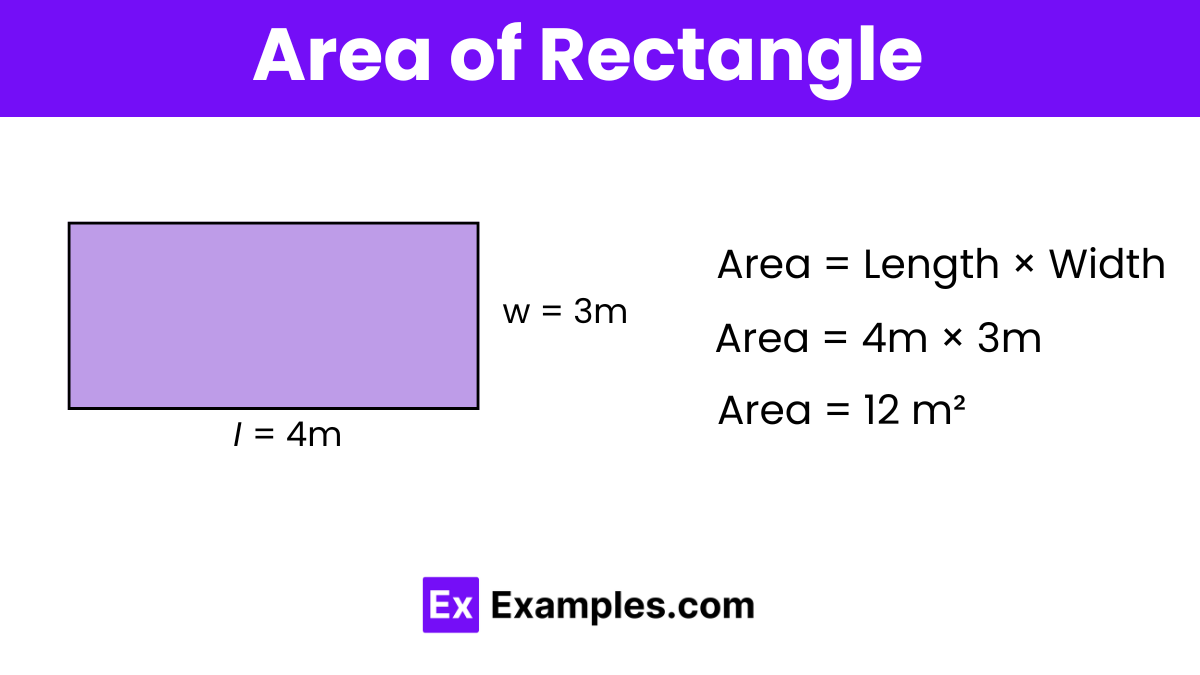
Suppose a rectangle has a length of 8 meters and a width of 3 meters.

Calculate the area:

• Length = 4 meters
• Width = 3 meters

Using the formula: Area = 4 meters × 3 meters = 12 square meters

So, the area of this rectangle is 24 square meters. This tells you how much space the rectangle covers.

Area of a Rectangle using Diagonal

To calculate the area of a rectangle using the diagonal, you need to know both the length of the diagonal and either the length or the width of the rectangle. This method involves a bit of geometry, specifically the Pythagorean theorem, because the diagonal divides the rectangle into two right triangles.

Method 1: Find the Missing Side First

Example: Calculate the area of a rectangle with a width of 5 cm and a diagonal of 13 cm.

Solution:

1. Use the Pythagorean theorem to find the missing length. The formula to find the missing side (length) is: length = √(diagonal²−width²)
2. Plug in the values: length= √(13²−5²) = √(169−25) = √144 = 12 cm
3. Now calculate the area: Area=length × width=12 cm×5 cm=60 cm²

Method 2: Use a Direct Formula

Example: Calculate the area of a rectangle where the length is 8 units, and the diagonal is 17 units.

Solution:

1. Apply the direct formula for the area using the length, diagonal, and missing width: Area = Length × √(Diagonal²−Length²)
2. Substitute the known values: Area=8 × √(17²−8²)= 8 × √(289−64) = 8 × √225 = 8×15
3. Calculate the final area: Area=120 square units

Both methods will help you calculate the area of a rectangle when the diagonal and one side length are known, using basic geometry principles and simple arithmetic.

Area of Rectangle using Perimeter

To calculate the area of a rectangle when you know the perimeter and one side, you first find the unknown side and then use that to calculate the area. Let’s go through an example to make this clear.

Example: Calculate the area of a rectangle where the perimeter is 24 units and the length is 7 units.

Solution:

1. Start with the Perimeter Formula:
   • The formula for the perimeter of a rectangle is P = 2 (l+w), where l is length and w is width.
2. Substitute the given values into the perimeter formula:
   • 24 = 2 (7+w)
3. Simplify to find the width:
   • Divide both sides by 2 to isolate the terms with w: 12 = 7+w
   • Subtract 7 from both sides to solve for w: =5 units
4. Calculate the Area:
   • Now that you have both the length and width, use the area formula A= l×w: A = 7×5 = 35 square units

FAQs

How do you solve the area of a rectangle problem?

Multiply the length by the width of the rectangle. Use the formula: Area = Length × Width to determine the rectangle’s area.

What is the area and perimeter of a rectangle?

Area is calculated by multiplying length by width. Perimeter is found by adding twice the length and twice the width: Perimeter = 2(Length + Width).

How do you find the area of a box?

Assuming the box is rectangular, calculate the area of one side using the formula for a rectangle’s area, then multiply by the number of sides.

What is the formula of volume of rectangle?

Technically, for a three-dimensional shape (rectangular prism), the volume is Length × Width × Height.

How do you find the area and volume of a rectangle?

Area: Multiply length by width. Volume (for a 3D rectangle, or prism): Multiply length by width by height. Use these formulas respectively for each calculation.