In the polynomial \(3x^2 + 5x - 7\), what is the coefficient of \(x\)?
3
-7
5
7
of 10
Coefficients
Coefficients
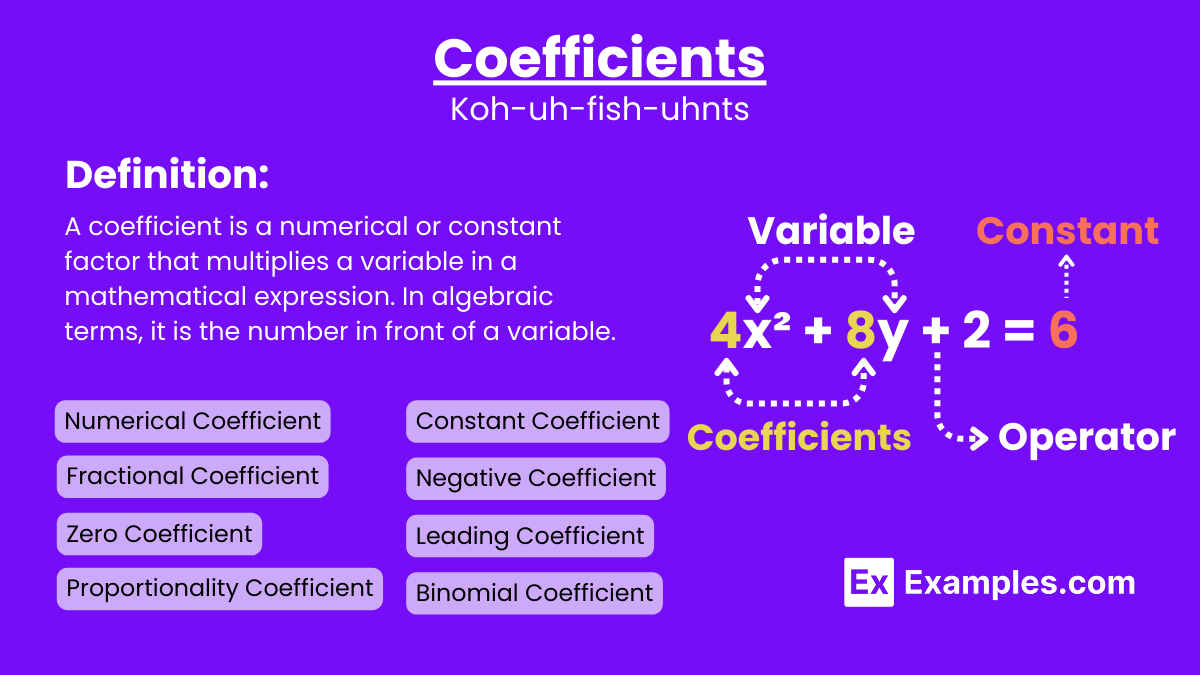
Coefficients are numerical values placed in front of variables in mathematical expressions to indicate multiplication. For example, in 3𝒙, 3 is the coefficient of the variable 𝒙. Coefficients quantify the contribution of the variable to the expression, playing a crucial role in algebraic equations, polynomials expressions, and various mathematical calculations.
What is a Coefficient?
Coefficients are fundamental in algebra, as they quantify the contribution of variables in equations and functions, allowing for the manipulation and solving of mathematical problems. They are used extensively in various fields, including physics, engineering, and economics, to model relationships and predict outcomes.
Coefficient of a Variable
A coefficient is a numerical factor that multiplies a variable in an algebraic expression. For example, in the term 5x, the coefficient is 5, meaning 5 times x. If the term is −3y, the coefficient is −3, and in 1/2 z, the coefficient is 1/2. When a variable stands alone, like x, the coefficient is implicitly 1. Coefficients help in simplifying expressions, such as combining 2x+3x to get 5x, and in solving equations, like solving 2x=10 by dividing both sides by the coefficient 2, resulting in x=5.
How to Find a Coefficient?
- Identify the Term:
- Locate the part of the algebraic expression that contains the variable you are interested in.
- Find the Number in Front:
- Look at the number directly in front of the variable. This number is the coefficient.
- Check the Sign:
- Note if there is a plus (+) or minus (-) sign before the number. This sign is part of the coefficient. If no number is present, the coefficient is 1, and if there is just a minus sign, the coefficient is -1.
Examples:
- Simple Coefficient:
- Term: 5x
- Coefficient: 5
- Negative Coefficient:
- Term: −3y
- Coefficient: -3
- Implicit Coefficient:
- Term: x
- Coefficient: 1
Numerical Coefficient
A numerical coefficient is the constant numerical factor that multiplies the variables in an algebraic term. It quantifies the contribution of the variable(s) in the term. For example, in the expression 4𝒙y, the numerical coefficient is 4, indicating that 𝒙y is multiplied by 4. In −3a²b, the numerical coefficient is -3. Identifying numerical coefficients is essential for simplifying, solving, and understanding algebraic expressions and equations.
Leading Coefficient
The leading coefficient is the numerical coefficient of the term with the highest degree in a polynomial. It is the coefficient of the term with the greatest exponent when the polynomial is written in standard form (terms in descending order of their exponents). The leading coefficient plays a crucial role in determining the polynomial’s behavior, especially its end behavior.
Examples:
In the polynomial 3𝒙⁴+2𝒙³−5𝒙+7, the leading coefficient is 3, as 3𝒙⁴ is the term with the highest degree.
For the polynomial −7𝒙⁵+4𝒙³+𝒙−2, the leading coefficient is -7, as −7𝒙⁵ is the term with the highest degree.
Different Types of Coefficients in Maths
1. Numerical Coefficient:
- The number that multiplies a variable in a term.
- Example: In 5x, 5 is the numerical coefficient.
2. Constant Coefficient:
- A fixed number that doesn’t change and isn’t multiplied by a variable.
- Example: In x+7, 7 is a constant term, not a coefficient.
3. Fractional Coefficient:
- A coefficient that is a fraction.
- Example: In 1/2y, 1/2 is the fractional coefficient.
4. Negative Coefficient:
- A coefficient with a negative sign.
- Example: In −3z, −3 is the negative coefficient.
5. Zero Coefficient:
- A coefficient that is zero, which makes the entire term zero.
- Example: In 0x, 0 is the zero coefficient.
6. Leading Coefficient:
- The coefficient of the term with the highest power of the variable in a polynomial.
- Example: In 4x³+3x²−2, 4 is the leading coefficient.
7. Proportionality Coefficient:
- A constant ratio between two directly proportional quantities.
- Example: In y=kx, k is the proportionality coefficient.
8. Binomial Coefficient:
- The coefficients in the expansion of a binomial raised to a power, represented by combinations.
- Example: In (x+y)²=x²+2xy+y², 1, 2, and 1 are the binomial coefficients.
Tips and Tricks on Coefficient
- Identify the Variable: Find the variable in the term; the number in front is the coefficient.
- Check for Signs: Note the plus (+) or minus (-) sign before the number, as it affects the coefficient.
- Implicit Coefficients: If no number is written, the coefficient is 1; if only a minus sign, the coefficient is -1.
- Combine Like Terms: Add or subtract the coefficients of like terms (e.g., 2x+3x=5x2x + 3x = 5x2x+3x=5x).
- Distributive Property: Multiply the coefficient by each term inside parentheses (e.g., 3(x+4)=3x+123(x + 4) = 3x + 123(x+4)=3x+12).
Coefficient Examples
| Simplify: Expression: 6x Solution: Coefficient: 6 | Simplify: Expression: −4y Solution: Coefficient: -4 |
| Simplify: Expression: 3a²b Solution: Coefficient: 3 | Simplify: Expression: 7x³ + 2x Solution: Coefficient of x³: 7 Coefficient of x: 2 |
| Simplify: Expression: −5m²n+9n Solution: Coefficient of m²n: -5 Coefficient of n: 9 | Simplify: Expression: x Solution: Coefficient: 1 (implicit) |
| Simplify: Expression: −z Solution: Coefficient: -1 (implicit) | Simplify: Expression: 4p²q³ Solution: Coefficient: 4 |
| Simplify: Expression: 8t−3t² Solution: Coefficient of t: 8 Coefficient of t²: -3 | Simplify: Expression: 2x⁴−7x²+x−5 Solution: Coefficient of x⁴: 2 Coefficient of x²: -7 Coefficient of x: 1 (implicit) |
How do you identify the coefficient in an expression?
To identify the coefficient, look for the number directly in front of the variable. For example, in 5x, the coefficient is 5.
What is a leading coefficient?
The leading coefficient is the coefficient of the term with the highest degree in a polynomial. It is significant in determining the polynomial’s behavior.
Can coefficients be negative?
Yes, coefficients can be negative. For example, in −4y, the coefficient is -4.
How do coefficients affect the graph of a polynomial?
The coefficients determine the steepness, direction, and width of the graph of a polynomial. The leading coefficient, in particular, affects the end behavior of the graph.
How do you find the coefficient in a term with multiple variables?
Identify the numerical part that multiplies all the variables. For example, in 5x²y, the coefficient is 5.
Are coefficients always integers?
No, coefficients can be any real numbers, including fractions and decimals. For example, in 0.5x, the coefficient is 0.5.
Can coefficients be zero?
Yes, a coefficient can be zero, which means the term does not contribute to the expression. For example, in
0⋅x, the term is effectively zero.
How do coefficients relate to derivatives in calculus?
In calculus, the coefficient of a term in a polynomial is multiplied by the exponent during differentiation. For example, the derivative of
5𝑥³ is 15𝑥² (5 multiplied by 3).
How are coefficients used in systems of equations?
In systems of equations, coefficients are used to form the equations that describe the relationships between variables. They are key in methods like substitution and elimination.
What is an implicit coefficient?
An implicit coefficient is the coefficient that is understood to be 1 if no number is written in front of the variable. For example, in x, the coefficient is implicitly 1.
Share :
Practice Test
What is the coefficient of \(x^3\) in the polynomial \(4x^3 - 2x^2 + 7x - 1\)?
4
-2
6
-1
of 10
In the expression \(2a^2b - 3ab^2 + 4b\), what is the coefficient of \(ab^2\)
2
-3
4
6
of 10
What is the coefficient of \(y^2\) in the polynomial \(5x^2 - 3xy^2 + 2y^2\)?
5
-3
2
0
of 10
In the polynomial \(7x^3 + 4x^2 - x + 6\), what is the coefficient of the constant term?
7
4
-1
6
of 10
What is the coefficient of \(x^2\) in the polynomial \(9x^3 - 4x^2 + 2x + 8\)?
9
-4
2
8
of 10
In the polynomial \(6a - 3b + 4ab\), what is the coefficient of \(a\)?
6
-3
5
7
of 10
What is the coefficient of \(x\) in the polynomial \(2x^3 + 7x - 9\)?
2
7
-9
0
of 10
In the polynomial \(x^2 - 5x + 6\), what is the coefficient of \(x^2\)?
-5
6
1
0
of 10
What is the coefficient of \(z^2\) in the polynomial \(3z^3 - 2z^2 + z + 4\)?
3
-2
1
4
of 10