Compound Interest

Compound Interest is the interest on a loan or deposit calculated based on both the initial principal and the accumulated interest from previous periods. Unlike simple interest, which is calculated only on the principal amount, compound interest grows at a faster rate, as it is calculated periodically (annually, semi-annually, quarterly, or monthly).

What is Compound Intrest?

Compound interest is the interest on a principal amount that accumulates over time, calculated on both the initial principal and the interest that has been added. This results in exponential growth, as interest is earned on interest, leading to higher returns compared to simple interest.

How Compound Interest Works

Compound interest works by calculating interest on the initial principal, which also includes all of the accumulated interest from previous periods. Here’s a step-by-step explanation:

1. Principal Amount: The initial amount of money invested or borrowed.
2. Interest Rate: The percentage at which interest is calculated.
3. Compounding Period: The frequency with which interest is added to the principal (e.g., annually, semi-annually, quarterly, monthly).
4. Interest Calculation: At each compounding period, interest is calculated on the current principal, which includes previously added interest.
5. Growth: Over time, the amount grows exponentially as interest is added on top of interest already earned.

Example Calculation:
Suppose you invest $1,000 at an annual interest rate of 5%, compounded annually.

• Year 1: $1,000 + ($1,000 * 0.05) = $1,050
• Year 2: $1,050 + ($1,050 * 0.05) = $1,102.50
• Year 3: $1,102.50 + ($1,102.50 * 0.05) = $1,157.63

The Power of Compound Interest

Compound interest is a powerful financial concept that allows your money to grow exponentially over time. Here’s why it’s so impactful:

Exponential Growth : Unlike simple interest, which is calculated only on the principal, compound interest is calculated on both the initial principal and the accumulated interest. This means your investment grows faster and faster as time goes on.

Time Factor : The longer you leave your money invested, the more powerful compound interest becomes. Even small amounts can grow significantly if given enough time. This is why starting early is crucial.

Interest on Interest : Compound interest means earning interest on the interest already earned. This snowball effect can turn modest investments into substantial sums over long periods.

Compounding Interest Periods

The frequency with which interest is added to the principal amount in an investment or loan is known as the compounding period. Different compounding periods can significantly affect the total amount of interest accrued. Here’s a closer look at the various compounding periods:

Yearly Compounding

Interest is compounded once per year. With yearly compounding, interest is added to the principal balance once every year. This is the simplest form of compounding. Although the frequency is the lowest compared to other compounding periods, it still allows the principal to grow over time.

Example: If you invest $1,000 at an annual interest rate of 5%, after one year, your investment grows to $1,050. After two years, it grows to $1,102.50, and so on.

Quarterly Compounding

Interest is compounded four times per year. In quarterly compounding, interest is calculated and added to the principal balance every three months. This results in more frequent additions of interest to the principal, leading to faster growth compared to yearly compounding.

Example: A $1,000 investment at a 5% annual interest rate, compounded quarterly, will result in approximately $1,050.95 after one year. The interest is calculated at 1.25% (5% divided by 4) every quarter.

Monthly Compounding

Interest is compounded twelve times per year. Monthly compounding means interest is calculated and added to the principal balance every month. This frequent addition of interest accelerates the growth of the investment compared to quarterly or yearly compounding.

Example: Investing $1,000 at a 5% annual interest rate, compounded monthly, will result in approximately $1,051.16 after one year. The interest is calculated at approximately 0.4167% (5% divided by 12) every month.

Daily Compounding

Interest is compounded 365 times per year. With daily compounding, interest is calculated and added to the principal balance every day. This is the most frequent compounding period commonly available and results in the fastest growth of the investment.

Example: A $1,000 investment at a 5% annual interest rate, compounded daily, will grow to approximately $1,051.27 after one year. The interest is calculated at approximately 0.0137% (5% divided by 365) every day.

Impact on Investment

The more frequent the compounding period, the more interest will be accrued. This is because interest is calculated and added to the principal more often, leading to a higher amount of interest in subsequent periods.

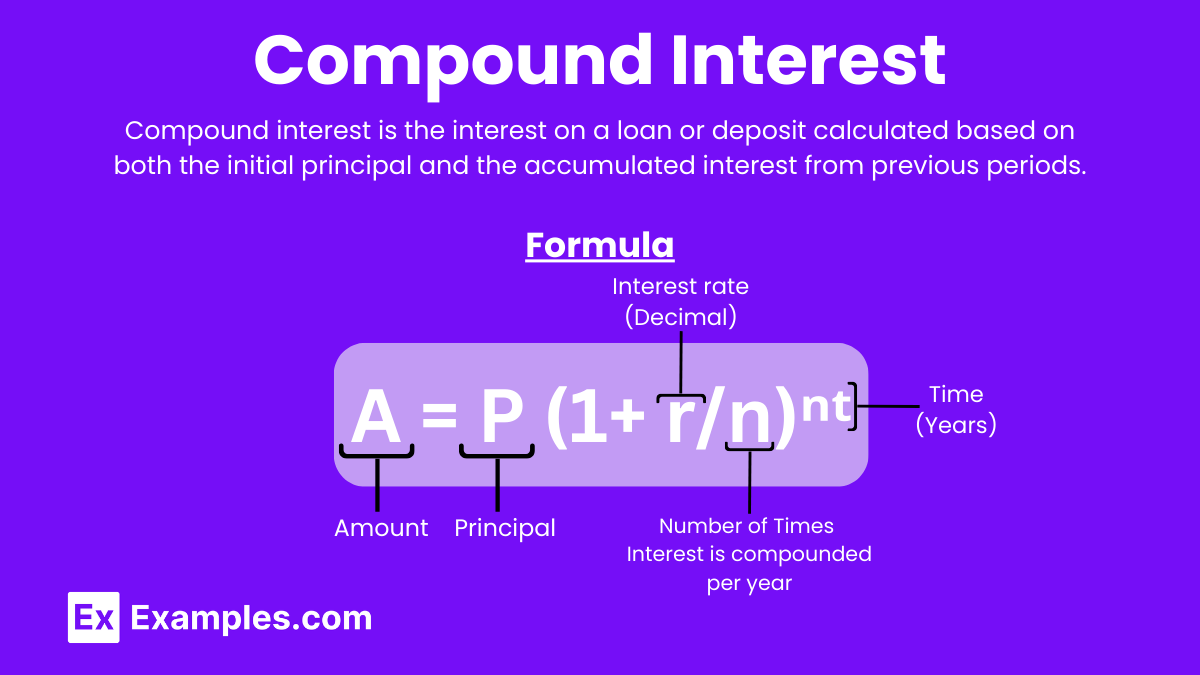
Formula

The formula to calculate compound interest is:
[ A = P (1 + r/n ]ⁿᵗ
Where:

• ( A ) = the future value of the investment/loan, including interest
• ( P ) = the principal investment/loan amount
• ( r ) = the annual interest rate (decimal)
• ( n ) = the number of times interest is compounded per year
• ( t ) = the number of years the money is invested/borrowed for

By understanding and leveraging different compounding periods, you can optimize your investment strategy for maximum returns.

Advantages and Disadvantages of Compound Interest

Advantages	Disadvantages
Exponential Growth	Potential for Higher Debt
Compound interest allows investments to grow exponentially over time as interest is earned on both the principal and accumulated interest.	For loans, compound interest can lead to significantly higher debt if payments are not managed properly.
Higher Returns Over Time	Complexity in Calculations
Long-term investments benefit greatly from compound interest, resulting in higher returns compared to simple interest.	Understanding and calculating compound interest can be complex, especially with varying compounding periods.
Encourages Long-Term Savings	Interest Rate Sensitivity
Compound interest encourages saving and investing for the long term, as the benefits become more pronounced over time.	Small changes in interest rates can have a significant impact on the total amount of interest accrued.
Better Financial Planning	Variable Compounding Periods
Helps in better financial planning and achieving long-term financial goals through systematic investments.	Different financial products have different compounding periods, making it difficult to compare them directly.
Reinvestment of Earnings	Inflation Impact
Earnings from interest are reinvested, leading to increased wealth accumulation.	Inflation can erode the real value of returns from compound interest, especially in low-interest environments.
Maximizes Returns	Requires Patience
Maximizes the returns on investments by leveraging the power of compounding over multiple periods.	Requires a long-term perspective and patience to see significant benefits.

Tools for Calculating Compound Interest

• Online Calculators: Use websites like Investopedia or Bankrate for quick compound interest calculations.
• Spreadsheet Software: Utilize Excel or Google Sheets with built-in financial functions like FV.
• Mobile Apps: Download financial calculator apps from the App Store or Google Play.
• Financial Calculators: Invest in a dedicated financial calculator, such as the Texas Instruments BA II Plus.

Compound Interest Examples

Example 1: Annual Compounding

You invest $1,000 at an annual interest rate of 5%, compounded annually. After one year, your investment grows to $1,050. After two years, it grows to $1,102.50. By the end of the third year, your investment is worth $1,157.63. The growth accelerates because you earn interest on the interest already added.

Example 2: Monthly Compounding

You deposit $2,000 in a savings account with an annual interest rate of 6%, compounded monthly. Each month, the interest is calculated and added to the principal. After one month, the balance becomes $2,010. After two months, it’s $2,020.10, and it continues to grow each month. By the end of the year, your savings will have grown to approximately $2,127.16 due to monthly compounding.

Example 3: Quarterly Compounding

A company invests $5,000 in a bond that pays 8% interest per year, compounded quarterly. The interest is added every three months. After the first quarter, the investment grows to $5,100. After the second quarter, it’s $5,202.50, and this process continues. By the end of the year, the investment will grow to about $5,432.43 due to the effect of quarterly compounding.

Example 4: Semi-Annual Compounding

You put $3,000 in a fixed deposit with a 4% annual interest rate, compounded semi-annually. Interest is calculated and added to the principal twice a year. After six months, the amount grows to $3,060. After a year, it’s $3,121.20. After two years, the balance will be approximately $3,244.90, showing the benefit of semi-annual compounding.

Example 5: Daily Compounding

You invest $1,500 in a high-yield savings account with an annual interest rate of 3%, compounded daily. Interest is added every day. After one day, the balance is $1,500.12. After one month, it’s approximately $1,503.76. After one year, your investment will grow to about $1,545.47 due to the daily compounding effect.