Derivative of Sec Square x

The derivative of sec²(x) is a fundamental concept in calculus, an area of mathematics that deals with changes. Calculating this derivative involves trigonometric functions and their rates of change, integrating concepts from algebra and the behavior of functions. This calculation is essential in various applications, including statistics where it helps in understanding trends and making predictions using methods like the least squares method. The outcome, 2sec⁡²(𝑥)tan⁡(𝑥), reflects how changes in 𝑥 influence the square and square roots of the secant function, connecting deeply with continuous (rational and irrational numbers) and discrete mathematics (integers). Understanding this derivative paves the way for advanced problem-solving in both theoretical and applied mathematics.

What is Derivative of Sec²x?

Derivative of Sec Square x Formula

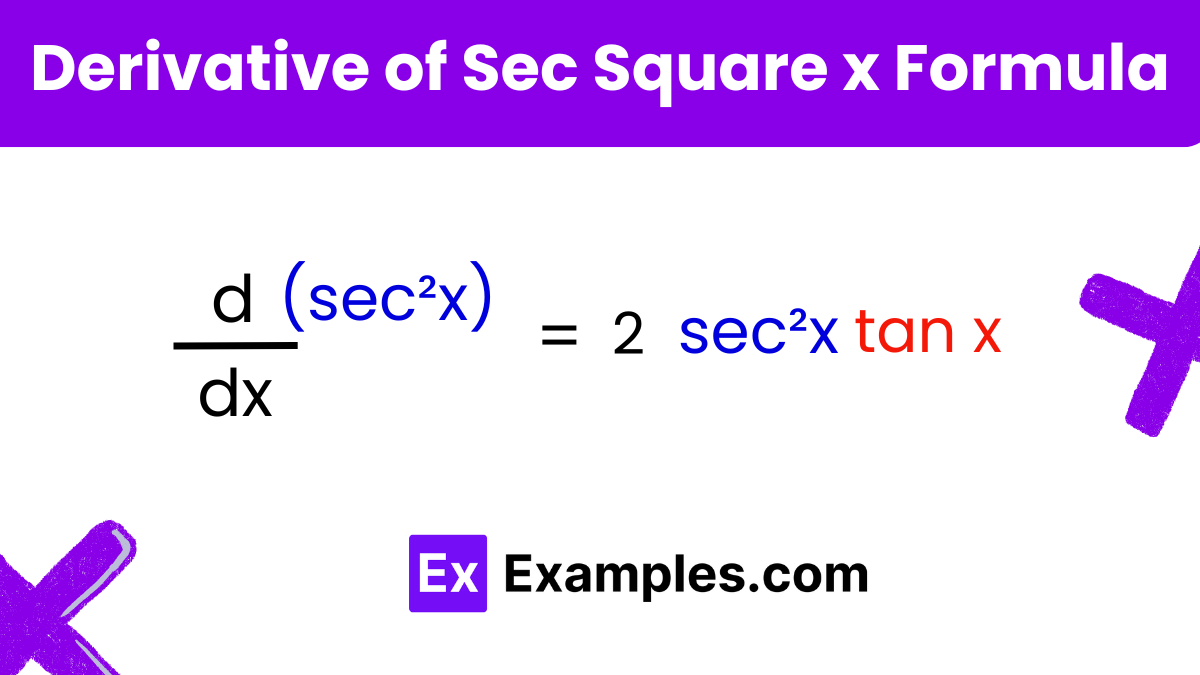
The derivative of sec⁡²(x) with respect to 𝑥 is given by the formula:

This result is derived using the chain rule, applying the derivative of sec⁡(𝑥), which is sec⁡(𝑥)tan⁡(𝑥), and then multiplying by the derivative of the outer function 𝑢⁡², where 𝑢 = sec⁡(𝑥).

Derivative of Sec^2x Using Chain Rule

To find the derivative of sec⁡²(x) using the chain rule, you follow these steps:

1. Identify the Inner Function: Let 𝑢 = sec⁡(𝑥). Then, sec⁡⁡²(x) can be rewritten as 𝑢⁡².
2. Differentiate the Inner Function: The derivative of sec⁡(𝑥) is sec⁡(𝑥)tan⁡(𝑥). Therefore, 𝑑𝑢/𝑑𝑥 = sec⁡(𝑥)tan⁡(𝑥).
3. Apply the Chain Rule: The chain rule states that 𝑑/𝑑𝑥[𝑢⁡²] = 2𝑢⋅𝑑𝑢/𝑑𝑥. Substituting 𝑢=sec⁡(𝑥) and 𝑑𝑢/𝑑𝑥 = sec⁡(𝑥)tan⁡(𝑥), you get:

𝑑/𝑑𝑥[sec⁡²(𝑥)] = 2sec⁡(𝑥)⋅sec⁡(𝑥)tan⁡(𝑥) = 2sec⁡⁡²(𝑥)tan⁡(𝑥)

Differentiation of Sec Square Using Quotient Rule

To differentiate sec²(x) using the quotient rule, we first recognize that sec⁡(𝑥)=1/cos⁡(𝑥)​, making sec²(𝑥)=(1/cos⁡(𝑥))².

The quotient rule states that for a function 𝑓(𝑥)=𝑔(𝑥)/ℎ(𝑥)​, the derivative is given by:

𝑓′(𝑥) = 𝑔′(𝑥)ℎ(𝑥)−𝑔(𝑥)ℎ′(𝑥)/[ℎ(𝑥)]²

Here, 𝑔(𝑥) = 1 and ℎ(𝑥) = cos⁡(𝑥), so 𝑔′(𝑥)=0 and ℎ′(𝑥) = −sin⁡(𝑥). Applying the quotient rule:

(1cos⁡(𝑥))² = 1/cos⁡²(𝑥)

𝑑/𝑑𝑥[1/cos²(𝑥)] = 0⋅cos⁡(𝑥)−1⋅(−sin⁡(𝑥))/cos⁡²(𝑥)

=sin⁡(𝑥)/cos⁡³(𝑥)

Simplifying this, we recognize sin⁡(𝑥)/cos⁡³(𝑥)​ as sin⁡(𝑥)cos⁡(𝑥)⋅1/cos⁡²(𝑥)​, which is tan⁡(𝑥)⋅sec⁡²(𝑥). Hence, the derivative is:

tan⁡(𝑥)⋅sec⁡²(𝑥)

Derivative of Sec^2x Using Product Rule

To differentiate sec²(x) using the product rule, first express sec⁡²(x) as a product of two functions:

sec⁡²(𝑥) = sec⁡(𝑥)⋅sec⁡(𝑥)

The product rule states that for two functions 𝑓(𝑥)f(x) and 𝑔(𝑥)g(x), the derivative (𝑓𝑔)′ is:

(𝑓𝑔)′=𝑓′𝑔+𝑓𝑔′

Here, both 𝑓(𝑥) and 𝑔(𝑥) are sec⁡(𝑥). Therefore, the derivatives 𝑓′(𝑥) and 𝑔′(𝑥) are sec⁡(𝑥)tan⁡(𝑥), which is the derivative of sec⁡(𝑥).

Applying the product rule:

(sec⁡(𝑥)⋅sec⁡(𝑥))′= sec⁡(𝑥)⋅sec⁡(𝑥)tan⁡(𝑥)+sec⁡(𝑥)⋅sec⁡(𝑥)tan⁡(𝑥)(sec(x)⋅sec(x))′

=sec⁡(𝑥)tan⁡(𝑥)⋅sec⁡(𝑥)+sec⁡(𝑥)tan⁡(𝑥)⋅sec⁡(𝑥)

=2sec⁡(𝑥)tan⁡(𝑥)⋅sec⁡(𝑥)

=2sec⁡²(𝑥)tan⁡(𝑥)

Derivative of Sec Square x Examples

Example 1: Basic Evaluation at 𝑥 = 𝜋/4​

Function: sec²2(x)

Point of Evaluation: 𝑥=𝜋/4​

1. Evaluate sec²(𝜋/4): Recall sec⁡(𝑥) = 1/cos⁡(𝑥). Since cos⁡(𝜋/4) = √2/2, sec⁡(𝜋/4) = √2​, so sec⁡²(𝜋/4) = 2.
2. Evaluate tan⁡(𝜋/4): tan⁡(𝜋/4) = 1.
3. Compute the Derivative: 2⋅2⋅1 = 4.

Result: The derivative 𝑑/𝑑𝑥[sec²(𝑥)] at 𝑥 = 𝜋/4 is 4.

Example 2: Applying the Derivative at 𝑥 = 𝜋/3​

Function: sec²(𝑥)

Point of Evaluation: 𝑥 = 𝜋/3​

1. Evaluate sec⁡²(𝜋/3): Since cos⁡(𝜋/3) = 1/2​, sec⁡(𝜋/3) = 2, so sec⁡²(𝜋3/) = 4.
2. Evaluate tan⁡(𝜋/3): tan⁡(𝜋/3) = √3​.
3. Compute the Derivative: 2⋅4⋅√3 = 8√3​.

Result: The derivative 𝑑/𝑑𝑥[sec⁡⁡²(𝑥)] at 𝑥 = 𝜋/3 is 8√3​.

Example 3: Graphical Understanding at 𝑥 = 0

Function: sec⁡⁡²(x)

Point of Evaluation: 𝑥 = 0

1. Evaluate sec⁡⁡⁡²(0): sec⁡(0) = 1, so sec⁡⁡⁡²(0) = 1.
2. Evaluate tan⁡(0): tan⁡(0) = 0.
3. Compute the Derivative: 2⋅1⋅0 = 0.

Result: The derivative 𝑑/𝑑𝑥[sec⁡⁡⁡²(𝑥)] at 𝑥 = 0 is 0.

FAQs

How do you apply the chain rule to find the derivative of sec⁡²(𝑥)?

To apply the chain rule, differentiate sec⁡(𝑥) to get sec⁡(𝑥)tan⁡(𝑥) and then multiply this by the derivative of sec⁡(𝑥 raised to the power of two, leading to the result 2sec⁡²(𝑥)tan⁡(𝑥).

Why might the derivative of sec⁡²(x) be important in practical applications?

This derivative is crucial in fields like physics and engineering, where trigonometric functions model waves, oscillations, and other phenomena. The derivative sec⁡⁡²(x) helps in understanding how these functions change over time or space.

How does understanding sec⁡²(x) and its derivative assist in learning advanced calculus?

Mastering these concepts aids in tackling more complex problems involving higher-level mathematical theories and applications, such as series expansions and advanced integration techniques.