Square & Square Root of 28
Square & Square Root of 28
Square of 28
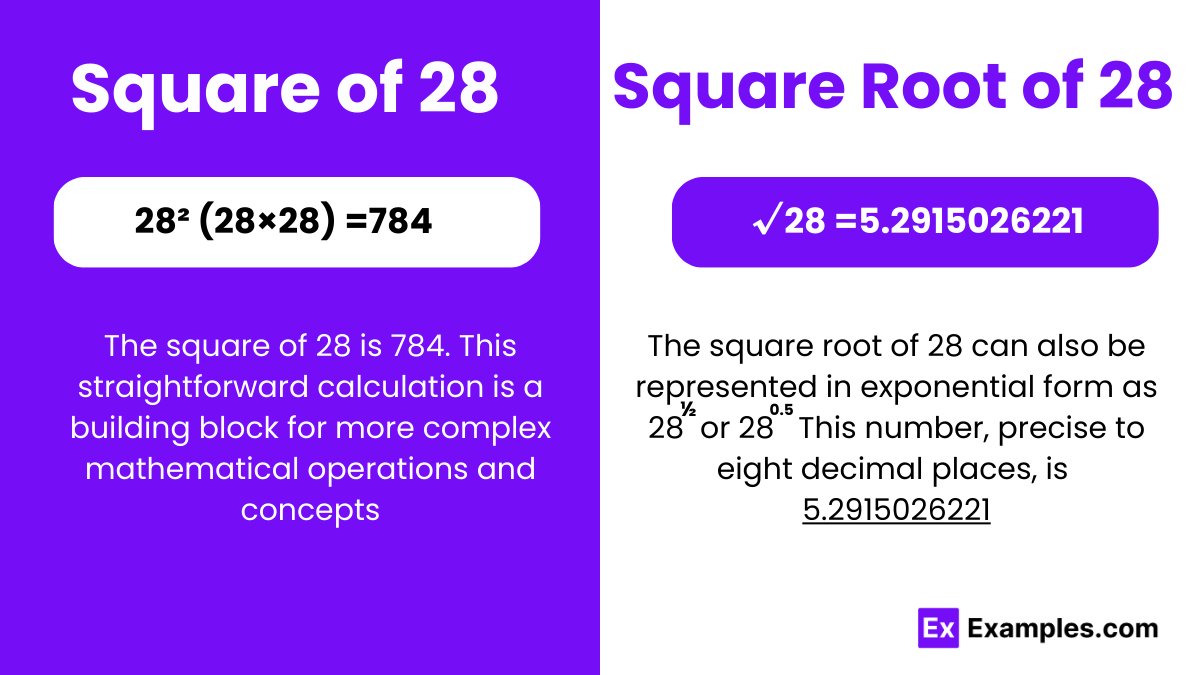
The square Numbers of 28 is calculated by multiplying 28 by itself. Mathematically, it’s expressed as 28². When you calculate it, the result is 784. This operation is commonly used in various mathematical contexts, such as geometry, algebra, and statistics, to represent area or to compute other important values. For example, in geometry, if you know the length of one side of a square is 28 units, then the area of that square would be 28² or 784 square units.
Square Root of 28
The square root of 28 is an irrational number, and it is approximately 5.29 when rounded to two decimal places. This value is derived because 5.29 multiplied by itself (5.29 × 5.29) approximates 28. The square root function determines what number, when multiplied by itself, produces the original number in this case, 28.
Understanding the square root is essential in various fields of mathematics and its applications, such as calculating the diagonal of a rectangle, simplifying algebraic expressions, or in physics and engineering, where it plays a role in formulas and models.
Square Root of 28: 5.29
Exponential Form: 28^½ or 28^0.5
Radical Form: √28
Is the Square Root of 28 Rational or Irrational?
This means it cannot be expressed as a simple fraction where both the numerator and the denominator are integers. The decimal expansion of an irrational numbers goes on indefinitely without repeating, which is the case with the square root of 28. When calculated to several decimal places, it does not settle into a repeating pattern.
Understanding the nature of numbers as rational or irrational is crucial for higher mathematics, including fields like algebra, geometry, and calculus, where such distinctions influence problem-solving approaches and the accuracy of solutions. In practical applications, knowing whether a number is rational or irrational can affect how calculations are performed, particularly in engineering and physics, where precise measurements are critical.
Methods to Find Value of Root 28
Finding the value of the square root of 28 can be approached using several straightforward methods. Here’s a guide to some practical ways:
1. Calculator Method:
Simplest Approach: Use a digital calculator. Type in 28 and press the square root button. This will give you a precise answer quickly, typically showing the square root of 28 as approximately 5.29.
2. Estimation Method:
Basic Guesswork: Since 25(which is 5²) and 36 (which is 6²)are the nearest perfect squares around 28, you know the square root of 28 lies between 5 and 6.
Start by guessing a bit more than 5, as 28 is closer to 25. A reasonable estimate would be around 5.3.
3. Long Division Method:
Traditional Technique: This method is similar to manual division. Start by dividing 28 by an initial guess (like 5.3), take the average of the divisor and the quotient, and use this average as a new guess. Repeat until you reach a stable value.
4. Newton’s Method:
Start with a guess: Suppose the square root is about 5.3.
Improve your guess: Use this easy formula to get a closer number:
Use a formula : New guess = 1/2 (Old guess+ 28/Old guess)
Refine and repeat: Keep using the formula with your new guess until the value stabilizes near the true square root.
Square Root of 28 by Long Division Method
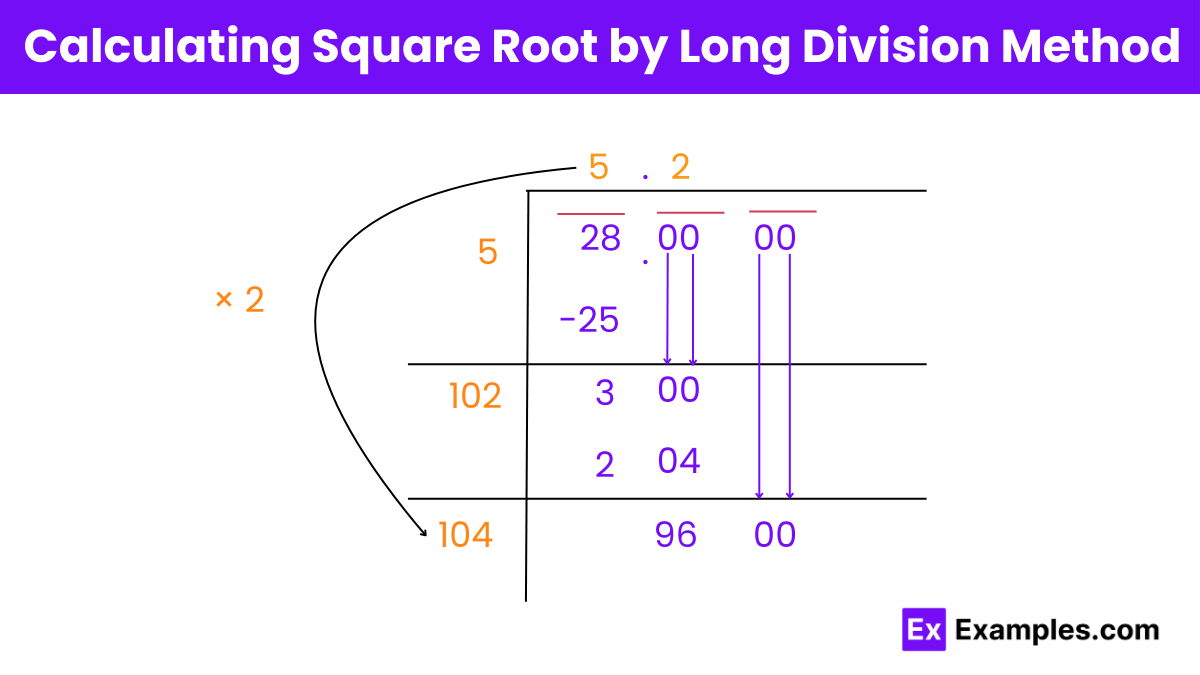
Calculating the square root of 28 using the long division method can seem complex at first, but with a step-by-step explanation, it becomes clear. Here’s how you can do it:
Step 1: Set Up:
Pair the digits of the number from right to left (in this case, 28 is already less than 100, so it’s considered as one pair, 28).
Draw the long division symbol. Place 28 inside it.
Step 2: Find the Largest Number:
Find the largest number whose square is less than or equal to 28. In this case, 5 squared is 25, which is the closest you can get without exceeding 28.
Step 3: Subtract and Bring Down:
Subtract 25 from 28, which leaves you with 3.
Normally, you would bring down the next pair of digits, but since there are no more digits, you would add a decimal point and bring down a pair of zeros, making it 300.
Step 4: Next Digit:
Double the divisor (5) to get 10. Now, find a digit X such that 10 X. X is less than or equal to 300. Here, X = 3 works because 103 × 3 = 309. but that’s slightly too high, so use X = 2 because 102 × 2 = 204.
Step 5: Subtract and Continue:
Place 2 next to the 5, making the new number 52.
Subtract 204 from 300 to get 96.
Bring down another pair of zeros, making it 9600.
Step 6: Repeat the Process:
Double the new number (52) to get 104. Find a number Y such that 104 Y. Y is less than 9600.
Continue this process, finding the next digits until you achieve the desired precision.
Using this method, the square root of 28 begins as 5.2, and you can continue adding more decimal places by repeating the steps. This method requires careful calculation but gives a very precise answer.
28 is Perfect Square root or Not?
A perfect square is defined as a number that is the product of an integer multiplied by itself. For instance, 25 is a perfect square because it equals 5 × 5 and 36 is a perfect square because it equals 6× 6
For 28, there is no integer that, when multiplied by itself, gives exactly 28. The square of 5 is 25, and the square of 6 is 36. Since 28 does not fit this pattern,it is not a perfect square.
FAQ’S
How do you simplify √ 28?
To simplify √28 ,express it as 2√7 by factoring 28 into 4 × 7 and taking the square root of 4.
Is 28 a cube root?
The number 28 on prime factorization gives 2 × 2 × 7. Here, the prime factor 2 is not in the power of 3. Therefore the cube root of 28 is irrational, hence 28 is not a perfect cube.
Share :
