What is the square of 11 using the expansion formula?
110
120
121
130
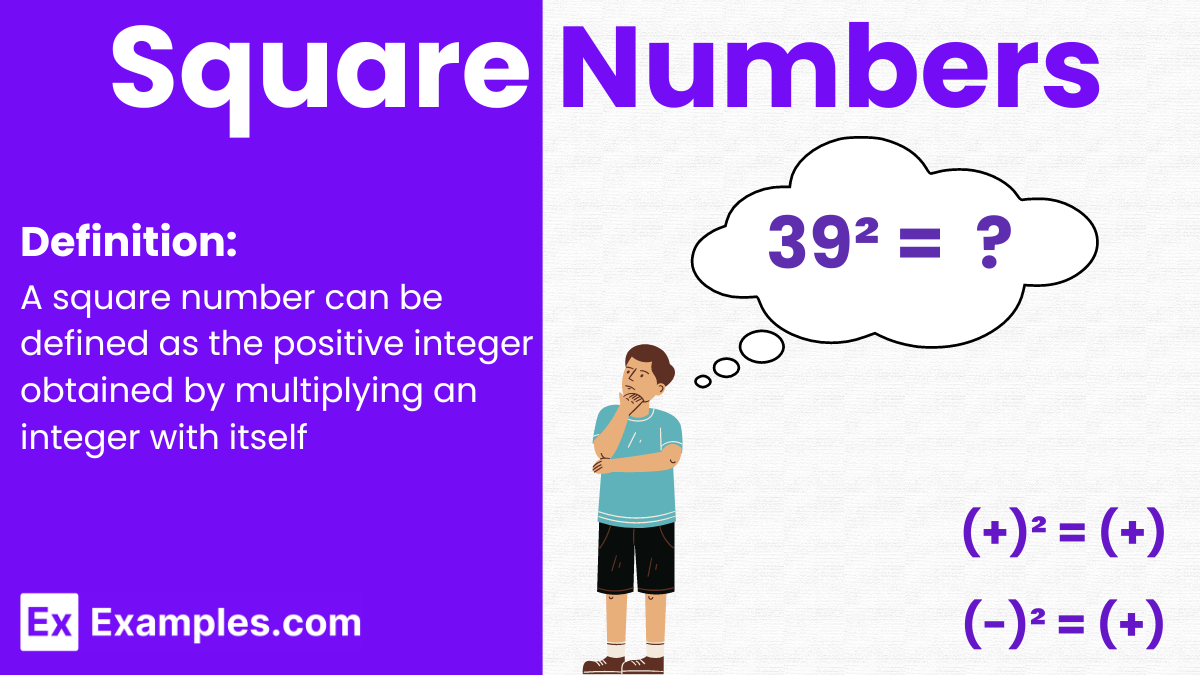
Square numbers, also known as perfect squares, are integers that can be expressed as the product of an integer with itself. This characteristic yields a distinct pattern of numbers, such as 1, 4, 9, 16, and so on. These numbers hold a significant place in mathematics due to their symmetrical properties and their role in various mathematical operations and theorems.
A square number can be defined as the positive integer obtained by multiplying an integer with itself.Square numbers are fundamental in mathematics, illustrating concepts of area and forming the basis of more complex mathematical discussions
A square number is a number obtained when an integer is multiplied by itself.And It is also referred to as “a perfect square.”
Multiplying two positive integers together yields a positive result. And similarly, when two negative integers are multiplied, the outcome is also positive.
(+) × (+) = (+)
(-) × (-) = (+)
Examples of square numbers:
4 × 4 =16
(-5) × (-5) = 25
square numbers involves a simple process based on the definition of what a square number is. A square number, or a perfect square, is the product of an integer multiplied by itself. Here’s how you can find square numbers:
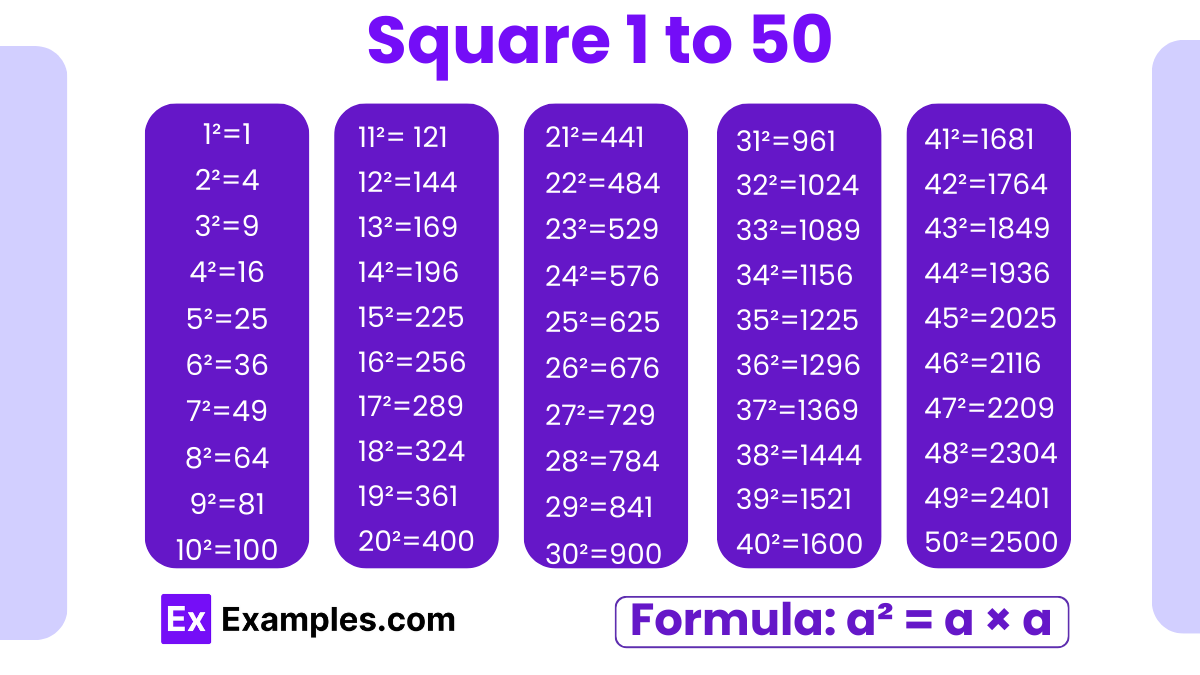
| Number – Square | Number – Square | Number – Square | Number – Square | Number – Square |
|---|---|---|---|---|
| 1²= 1 | 21²=441 | 41²=1681 | 61²=3721 | 81²=6561 |
| 2²=4 | 22²=484 | 42²=1764 | 62²=3844 | 82²=6724 |
| 3²=9 | 23²=529 | 43²=1849 | 63²=3969 | 83²=6889 |
| 4²=16 | 24²=576 | 44²=1936 | 64²=4096 | 84²=7056 |
| 5²=25 | 25²=625 | 45²=2025 | 65²=4225 | 85²=7225 |
| 6²=36 | 26²=676 | 46²=2116 | 66²=4356 | 86²=7396 |
| 7²=49 | 27²=729 | 47²=2209 | 67²=4489 | 87²=7569 |
| 8²=64 | 28²=784 | 48²=2304 | 68²=4624 | 88²=7744 |
| 9²=81 | 29²=841 | 49²=2401 | 69²=4761 | 89²=7921 |
| 10²=100 | 30²=900 | 50²=2500 | 70²=4900 | 90²=8100 |
| 11²=121 | 31²=961 | 51²=2601 | 71²=5041 | 91²=8281 |
| 12²=144 | 32²=1024 | 52²=2704 | 72²=5184 | 92²=8464 |
| 13²=169 | 33²=1089 | 53²=2809 | 73²=5329 | 93²=8649 |
| 14²=196 | 34²=1156 | 54²=2916 | 74²=5476 | 94²=8836 |
| 15²=225 | 35²=1225 | 55²=3025 | 75²=5625 | 95²=9025 |
| 16²=256 | 36²=1296 | 56²=3136 | 76²=5776 | 96²=9216 |
| 17²=289 | 37²=1369 | 57²=3249 | 77²=5929 | 97²=9409 |
| 18²=324 | 38²=1444 | 58²=3364 | 78²=6084 | 98²=9604 |
| 19²=361 | 39²=1521 | 59²=3481 | 79²=6241 | 99²=9801 |
| 20²=400 | 40²=1600 | 60²=3600 | 80²=6400 | 100²=10000 |
Two-digit square numbers are the squares of integers that result in values between 10 and 99.
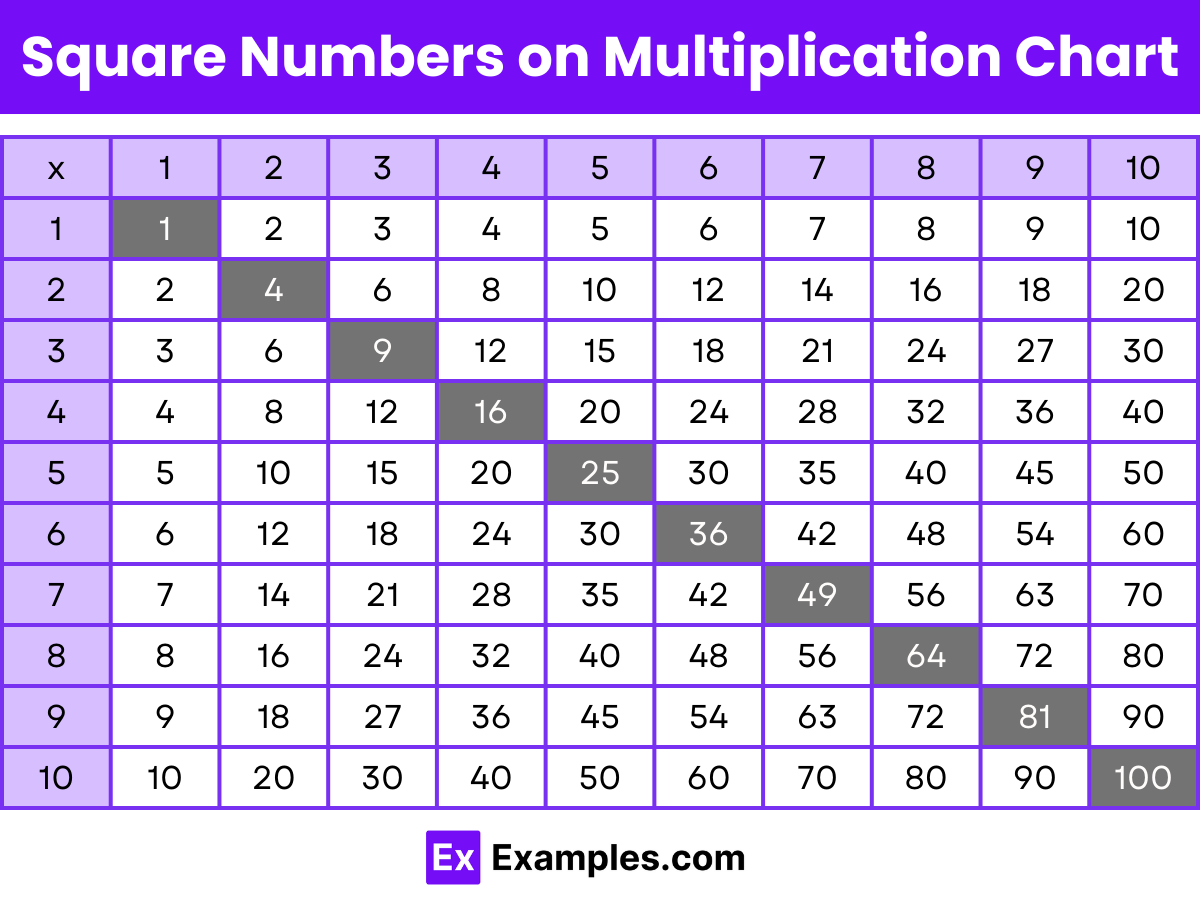
| x | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 1 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 2 | 2 | 4 | 6 | 8 | 10 | 12 | 14 | 16 | 18 | 20 |
| 3 | 3 | 6 | 9 | 12 | 15 | 18 | 21 | 24 | 27 | 30 |
| 4 | 4 | 8 | 12 | 16 | 20 | 24 | 28 | 32 | 36 | 40 |
| 5 | 5 | 10 | 15 | 20 | 25 | 30 | 35 | 40 | 45 | 50 |
| 6 | 6 | 12 | 18 | 24 | 30 | 36 | 42 | 48 | 54 | 60 |
| 7 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | 56 | 63 | 70 |
| 8 | 8 | 16 | 24 | 32 | 40 | 48 | 56 | 64 | 72 | 80 |
| 9 | 9 | 18 | 27 | 36 | 45 | 54 | 63 | 72 | 81 | 90 |
| 10 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | 100 |
Given a dataset: 4, 8, 12. Calculate the sum of squares for this dataset.
Answer: The mean is (4+8+12)/3=8. The squared differences from the mean are (4−8)2=16,(4−8)2=16, (8−8)2=0 and (12−8)2=16, (12−8)2=16. The sum of squares is 16+0+16=32.
Calculate the sum of squares of the first 5 natural numbers.
Answer: Use the formula n(n+1)(2n+1)/6 with n=5: 5(5+1)(2⋅5+1)/6=5⋅6⋅11/6=55
You conducted an experiment and collected the following observed (y) and predicted (y^) values:
Calculate the SSE.
Answer: Calculate the differences (3−2)2=1, (5−4)2=1, (7−6)2=1, (7−6)2=1. The SSE is 1+1+1=3.
Find the sum of squares of 3x2−2x+1 and 2x2+3x−4.
Answer: Squaring each term and then adding gives (3x2)2+(−2x)2+12+(2x2)2+(3x)2+(−4)2 which simplifies to 9x4+4x2+1+4x4+9x2+16, resulting in 13x4+13x2+17.
In a simple linear regression analysis, you have calculated the total sum of squares (SST) as 200 and the regression sum of squares (SSR) as 160. Calculate the residual sum of squares (SSE).
Answer: Since SST=SSR+SSE, we find SSE=SST−SSR=200−160=40.
No, 35 is not a square number. A square number results from multiplying an integer by itself, and no whole number multiplied by itself gives 35.
The square of 24 is 24²=576 Squaring a number means multiplying it by itself, so 24 multiplied by 24 equals 576.
No, 27 is not a square number. Square numbers are integers that can be expressed as the product of another integer with itself, and there is no integer whose square is 27.
The 14 square numbers up to 200 are 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, 169, and 196. These represent the squares of the integers from 1 to 14.
No, 30 is not a square number. Square numbers come from squaring integers, and 30 cannot be obtained by squaring any integer.
No, 60 is not a square number. There is no integer that, when multiplied by itself, results in 60, making it not a perfect square.
The 15th square number is 15²=225 This is because when you multiply 15 by itself, the product is 225.
13 is not a square number because it cannot be formed by squaring any whole number. Square numbers are the product of an integer multiplied by itself, and no integer squared equals 13.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 11 using the expansion formula?
110
120
121
130
What is 8² using repeated addition?
60
62
64
66
Calculate the square of 6
34
35
36
37
What is the square of 14 using the binomial expansion?
190
192
194
196
What is 5²?
15
20
25
30
Calculate the square of 13 using the binomial expansion.
160
169
170
180
What is the square of 10 using simple multiplication?
90
95
100
105
What is 4² ?
12
14
16
18
Calculate the square of 13 using the formula (a+b)² = a² + 2ab + b². Let a = 10 and b = 3.
159
167
169
171
What is 9² using repeated addition?
72
74
79
81
Before you leave, take our quick quiz to enhance your learning!

