What is the volume of a square pyramid with a base side length of 4 cm and a height of 9 cm?
48 cm³
56 cm³
60 cm³
64 cm³


A square pyramid is a three-dimensional geometric figure with a square base and four triangular faces converging at a point called the apex. In mathematics, this shape can be analyzed using algebra to explore its properties, such as volume and surface area. Concepts like square and square roots are vital in calculating these dimensions, especially when dealing with the pyramid’s height or slant height. Additionally, the least squares method, a statistical tool, may be used to optimize real-world pyramid constructions or models involving integers, rational, and irrational numbers. This integration of geometry with other mathematical disciplines like statistics and number theory highlights the interconnected nature of mathematical concepts. and the pyramids are Called after their base, such as
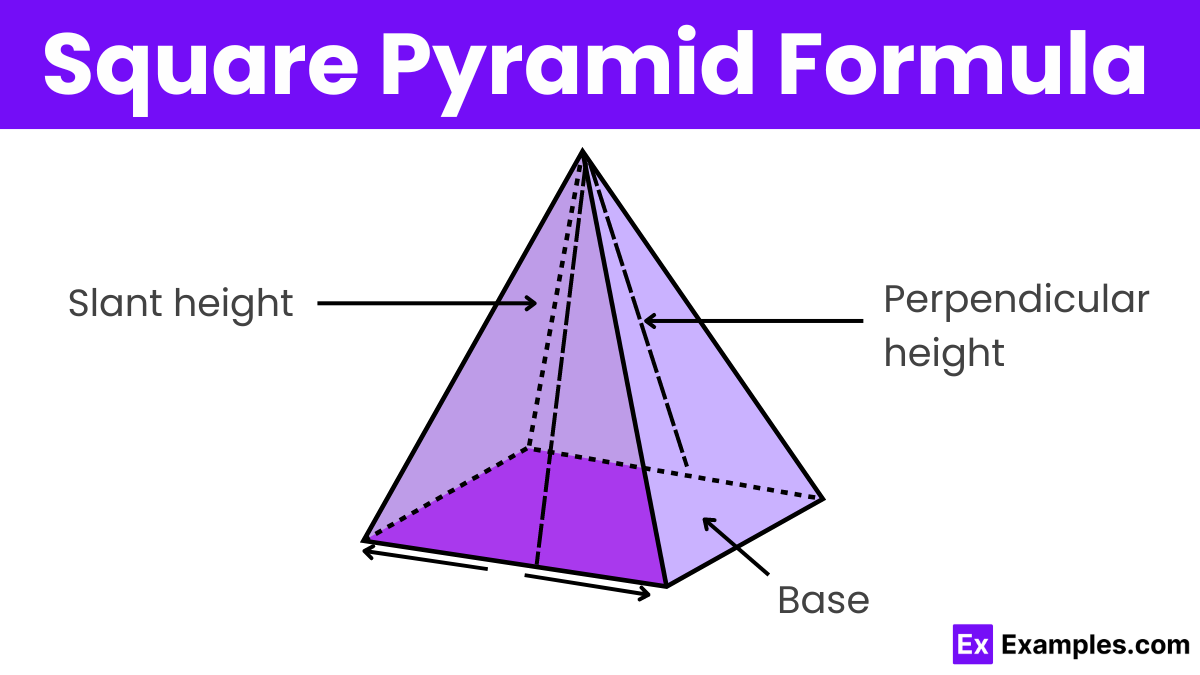
A square pyramid, characterized by a square base and triangular sides that meet at an apex, is a fundamental shape in geometry. Understanding how to calculate its volume and surface area involves specific formulas. Here are the key formulas used for a square pyramid:
The volume 𝑉 of a square pyramid is calculated by the formula:
Where:
Expressed with base side and height, the formula becomes: 𝑉 = 1/3𝑠²ℎ
The surface area 𝐴 of a square pyramid is the sum of the area of the base and the area of the triangular sides. The formula is:
Where:
The lateral surface area is the total area of just the triangular sides and is calculated without including the base:
This formula helps in finding the area of the material needed if the base is not covered.
Problem: Find the volume of a square pyramid with a base side length of 4 meters and a perpendicular height from the base to the apex of 9 meters.
Solution:
Using the formula for the volume of a square pyramid: 𝑉=1/3𝑠²ℎ.
Substitute the given values:
Answer: The volume of the pyramid is 48 cubic meters.
Problem: Calculate the surface area of a square pyramid with a base side length of 3 meters and a perpendicular height of 6 meters.
Solution:
First, calculate the slant height (𝑙) using the Pythagorean theorem (since the slant height is the hypotenuse of the triangle formed by the half of the base and the height):
Now, use the surface area formula:
The surface area of the pyramid is approximately 46.08 square meters.
Calculate the lateral surface area of a square pyramid with a base side length of 5 meters and a vertical height (from the base to the apex) of 12 meters.
Given:
First, we need to determine the slant height (𝑙), which is necessary for calculating the lateral surface area. The slant height can be found using the Pythagorean theorem in the right triangle formed by half the side of the base, the vertical height, and the slant height itself.
The formula for the lateral surface area is:
The lateral surface area of the square pyramid is approximately 122.6 square meters. This calculation shows how the geometry of the pyramid and basic trigonometry come together to solve practical problems involving real-world objects.
Square pyramids can be classified based on their symmetry, the orientation of their apex, and the relative lengths of their edges. Understanding these types helps in applications ranging from architectural design to geometric analysis. Here are the main types of square pyramids:
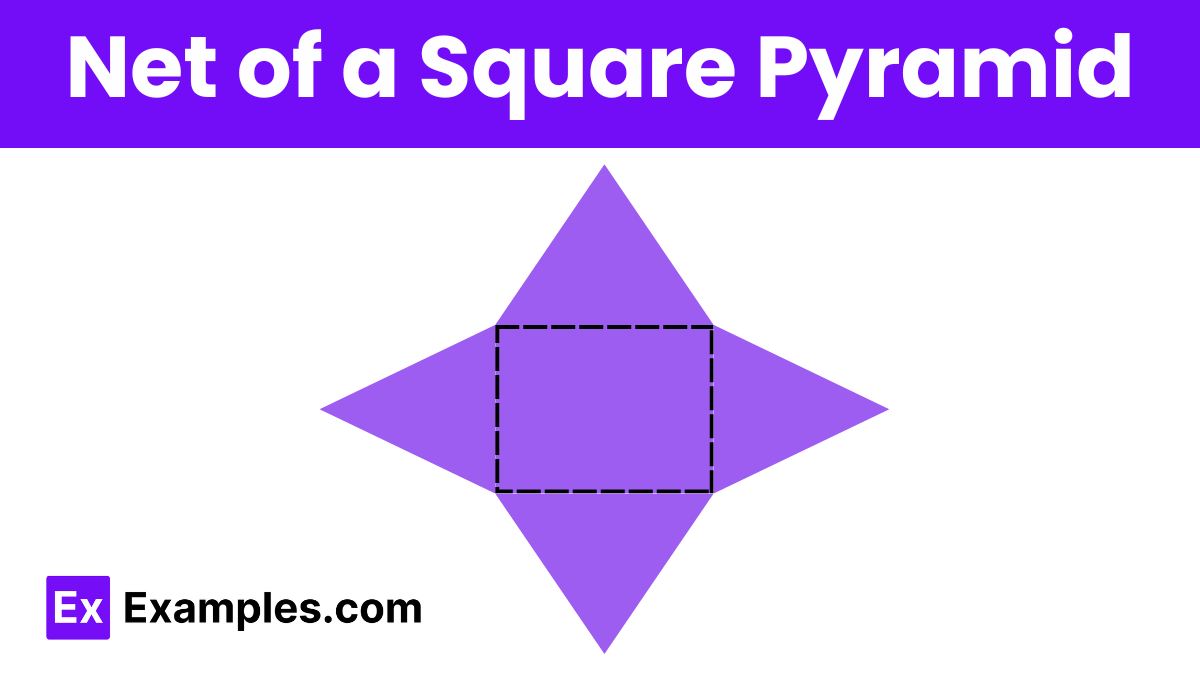
A net of a square pyramid is a two-dimensional representation that can be folded to form the three-dimensional shape of the pyramid. Understanding the net of a square pyramid helps visualize how the sides fit together and is particularly useful in educational contexts for teaching geometry, as well as in practical applications like crafting and architecture.
The net of a square pyramid consists of the following components:
Square pyramids, often studied in geometry and applied in various real-world contexts, have several key characteristics and properties that are crucial for understanding their structure and function. Here are some important notes on square pyramids:
Studying square pyramids helps students understand concepts of volume, surface area, and three-dimensional shape structure, enhancing spatial awareness and geometric visualization skills.
No, square pyramids are used in various fields besides mathematics, including architecture, engineering, and education. They serve as practical examples of geometric principles and are also aesthetically pleasing structures.
A regular square pyramid has its apex directly above the center of the base and is symmetrical, while an oblique square pyramid has an apex that is not aligned above the center of the base, causing the sides to be asymmetrical.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the volume of a square pyramid with a base side length of 4 cm and a height of 9 cm?
48 cm³
56 cm³
60 cm³
64 cm³
Find the slant height of a square pyramid with a base side length of 6 cm and a perpendicular height of 8 cm.
7 cm
8 cm
9 cm
10 cm
What is the lateral surface area of a square pyramid with a base side length of 5 cm and a slant height of 7 cm?
60 cm²
70 cm²
80 cm²
90 cm²
Determine the total surface area of a square pyramid with a base side length of 6 cm and a slant height of 10 cm.
96 cm²
108 cm²
120 cm²
156 cm²
What is the height of a square pyramid with a base area of 25 cm² and a volume of 50 cm³?
5 cm
6 cm
7 cm
8 cm
Calculate the length of the diagonal of the base of a square pyramid with a side length of 7 cm.
7 cm
8 cm
9 cm
10 cm
What is the area of one triangular face of a square pyramid with a base side length of 8 cm and a slant height of 12 cm?
40 cm²
45 cm²
48 cm²
52 cm²
If the height of a square pyramid is 9 cm and the volume is 108 cm³, what is the length of the base side?
4 cm
5 cm
6 cm
7 cm
Find the height of a square pyramid with a base side length of 5 cm and a volume of 100 cm³
12 cm
14 cm
16 cm
18 cm
What is the perimeter of the base of a square pyramid with a base side length of 9 cm?
36 cm
38 cm
40 cm
42 cm
Before you leave, take our quick quiz to enhance your learning!

