Trigonometry

What is Trigonometry?

Trigonometry is a branch of mathematics that studies relationships involving lengths and angles of triangles. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. It primarily deals with the sine, cosine, and tangent functions, which are ratios of sides of right triangles. These functions have profound applications in both theoretical and practical aspects, including physics, engineering, surveying, and computer graphics.

Trigonometric Ratios (Sin, Cos, Tan)

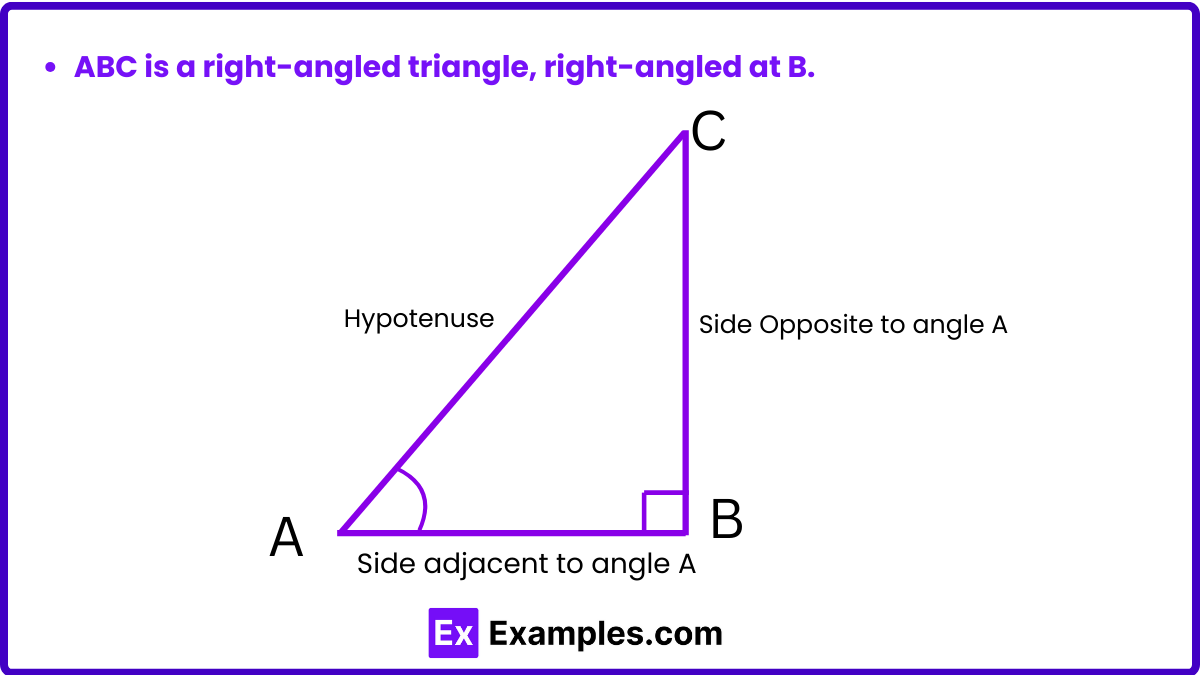
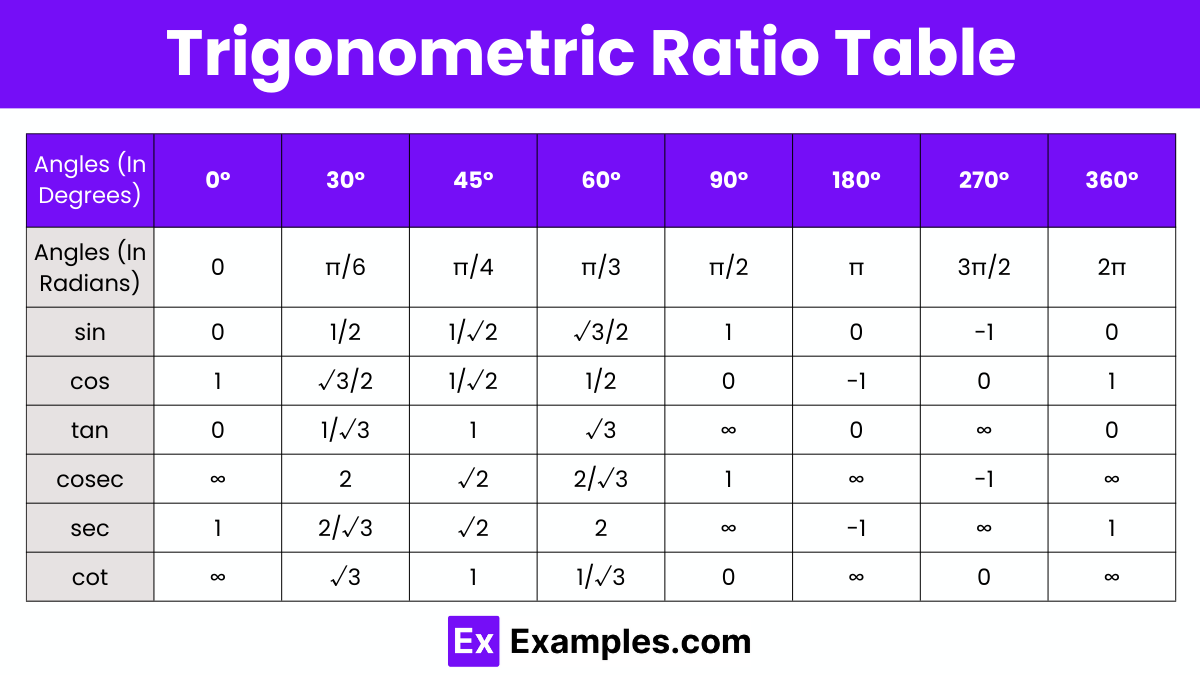
Trigonometric ratios are fundamental to the study of trigonometry and are used to relate the angles and sides of a right triangle. The three primary trigonometric ratios are sine, cosine, and tangent, commonly abbreviated as sin, cos, and tan, respectively. Here’s a breakdown of each:

Sine (sin)

The sine of an angle in a right triangle is the ratio of the length of the opposite side to the length of the hypotenuse. It is defined as: sin⁡(θ)=opposite/hypotenuse​

Cosine (cos)

The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse. It is defined as: cos⁡(θ)=adjacent/hypotenuse​

Tangent (tan)

The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side. It is defined as: tan⁡(θ)=opposite/adjacent

Six Trigonometric Functions

1. Sine (sin)

• Definition: The ratio of the length of the opposite side to the hypotenuse in a right triangle.
• Formula: sin⁡(θ)=opposite/hypotenuse​

2. Cosine (cos)

• Definition: The ratio of the length of the adjacent side to the hypotenuse.
• Formula: cos⁡(θ)=adjacent/hypotenuse​

3. Tangent (tan)

• Definition: The ratio of the length of the opposite side to the adjacent side.
• Formula: tan⁡(θ)=opposite/adjacent​

4. Cotangent (cot)

• Definition: The reciprocal of the tangent or the ratio of the length of the adjacent side to the opposite side.
• Formula: cot⁡(θ)=1/tan⁡(θ)=adjacent/opposite​

5. Secant (sec)

• Definition: The reciprocal of the cosine or the ratio of the hypotenuse to the adjacent side.
• Formula: sec⁡(θ)=1/cos⁡(θ)=hypotenuse/adjacent​

6. Cosecant (csc)

• Definition: The reciprocal of the sine or the ratio of the hypotenuse to the opposite side.
• Formula: csc⁡(θ)=1/sin⁡(θ)=hypotenuse/opposite

Even and Odd Trigonometric Functions

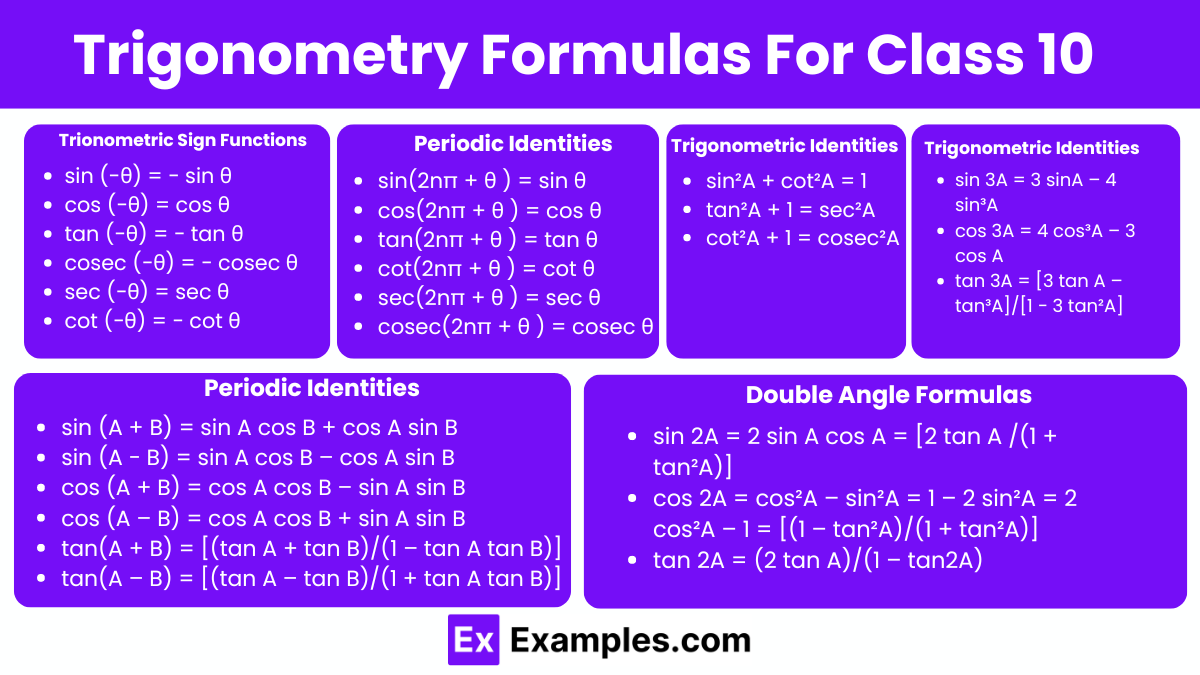
Odd Trigonometric Functions: A trigonometric function is classified as odd if it satisfies the condition f(−x)=−f(x) This implies that the function is symmetric with respect to the origin, meaning that reflecting the function across both axes results in the same graph.

Even Trigonometric Functions: A trigonometric function is classified as even if it satisfies the condition f(−x)=f(x). This indicates that the function is symmetric about the y-axis, meaning that reflecting the function across the y-axis results in the same graph.

Function	Formula	Description
Sine (sin)	(sin(-x) = -sin(x))	Sine is an odd function.
Cosine (cos)	(cos(-x) = cos(x))	Cosine is an even function.
Tangent (tan)	(tan(-x) = -tan(x))	Tangent is an odd function.
Cosecant (csc)	(csc(-x) = -csc(x))	Cosecant is an odd function.
Secant (sec)	(sec(-x) = sec(x))	Secant is an even function.
Cotangent (cot)	(cot(-x) = -cot(x))	Cotangent is an odd function.

Trigonometry Angles

1.Angle Measurement

• Degrees: One of the most common units for measuring angles. A full circle is divided into 360 degrees.
• Radians: Another vital unit for measuring angles, particularly in higher mathematics and applications involving calculus. A full circle is 2π radians. Radians are particularly useful because they simplify the integration and differentiation of trigonometric functions.

2. Standard Angles

• Acute Angles: Angles less than 90 degrees (or π/2 radians).
• Right Angles: Exactly 90 degrees (or π/2 radians).
• Obtuse Angles: Greater than 90 degrees but less than 180 degrees (or between π/2 and π radians).
• Straight Angles: Exactly 180 degrees (or π radians).
• Reflex Angles: More than 180 degrees but less than 360 degrees (or between π and 2π radians).

3. Special Angles in Trigonometry

• 30° (or π/6), 45° (or π/4), and 60° (or π/3) Angles: These angles are commonly used in trigonometry because they correspond to specific, easy-to-remember values of sine, cosine, and tangent.
• 0° and 90° (or 0 and π/2 radians): These are critical for defining the initial and maximal values of the trigonometric functions.

4. Negative Angles and Angle Cycles

• Negative Angles: Rotating in the clockwise direction, contrary to the positive counter-clockwise standard.
• Angle Cycles (Periodicity): Trigonometric functions are periodic, meaning they repeat their values in regular intervals. For instance, sine and cosine have a period of 2π radians, which means they repeat every 2π radians.

5. Angle Sum and Difference Identities

• These identities allow for the calculation of the sine, cosine, and tangent of the sum or difference of two angles, facilitating the simplification of complex trigonometric expressions and proving useful in various applications such as signal processing.

6. Quadrants

• The coordinate plane is divided into four quadrants by the x-axis and y-axis, which affect the sign (+/-) of the trigonometric functions depending on the quadrant an angle terminates in.

Trigonometry values Table

List of Trigonometry Formulas

Download All Trigonometry Formulas

Reciprocal Identities

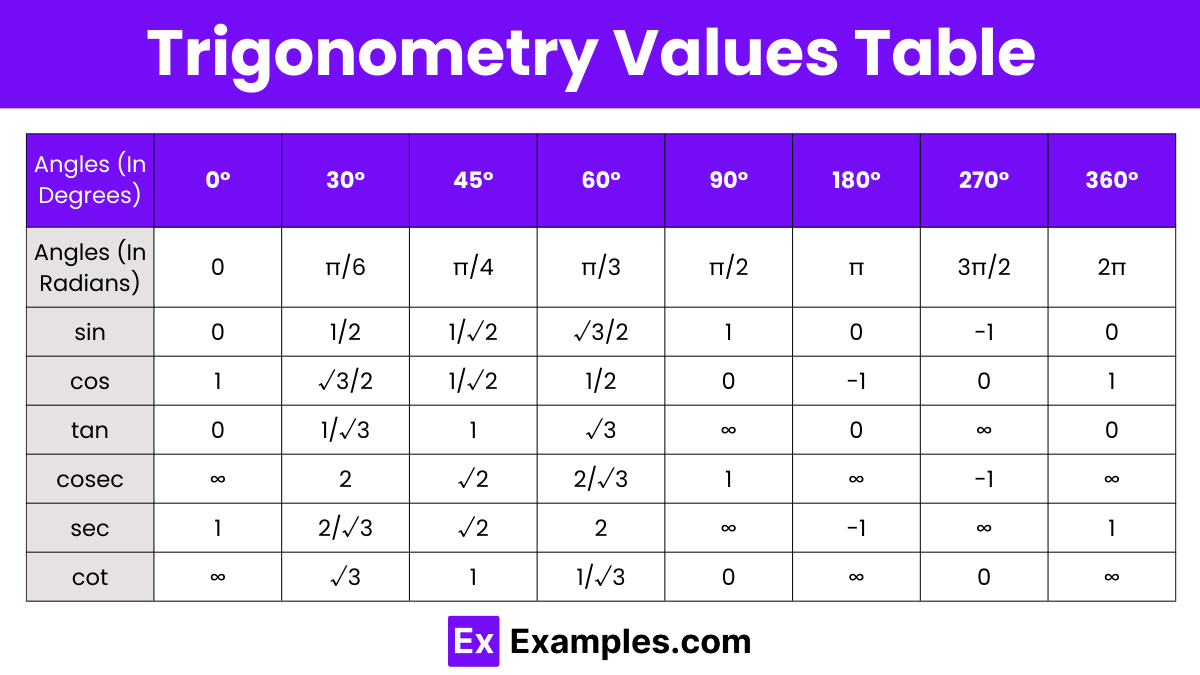
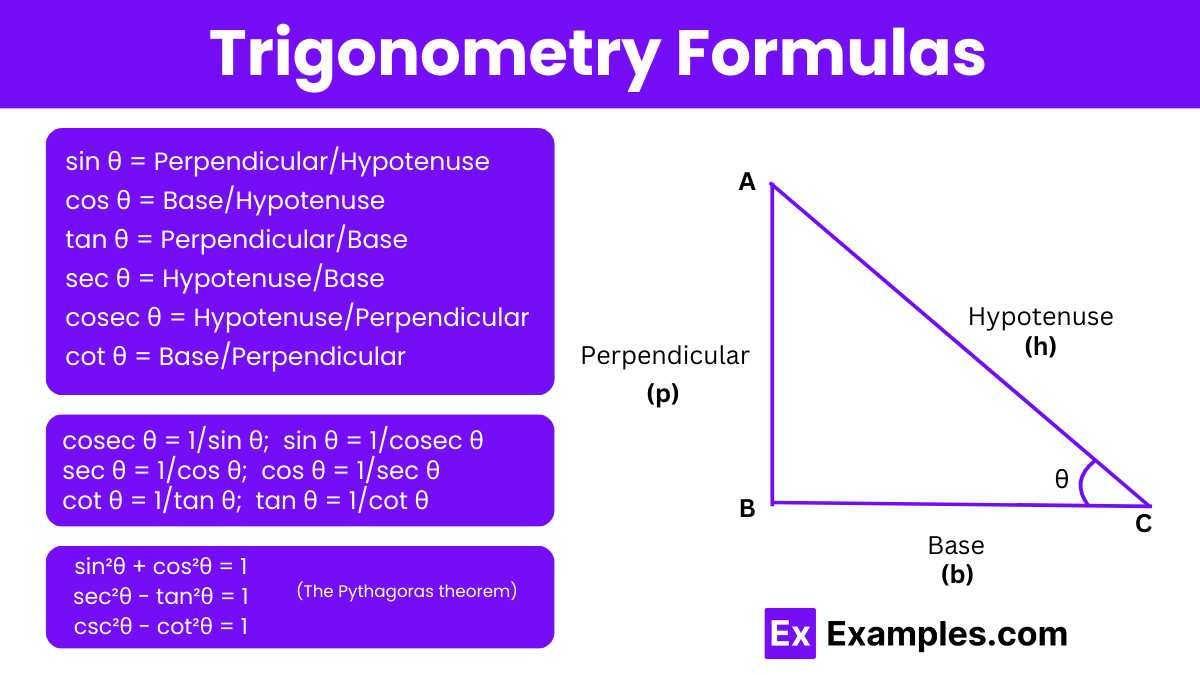
Cosecant, secant, and cotangent are the reciprocals of the basic trigonometric ratios: sine, cosine, and tangent, respectively. These reciprocal identities are derived from the properties of a right-angled triangle and play a crucial role in trigonometry. They are often used to simplify and solve trigonometric problems. The formulas for these reciprocal trigonometric identities, which are essential for various calculations and transformations in trigonometry, are:

• cosec θ = 1/sin θ; sin θ = 1/cosec θ
• sec θ = 1/cos θ; cos θ = 1/sec θ
• cot θ = 1/tan θ; tan θ = 1/cot θ

Pythagorean Identities

The Pythagoras theorem states that in a right-angled triangle, the square of the hypotenuse (ccc) is equal to the sum of the squares of the other two sides (aaa and bbb). Mathematically, this is expressed as: c^² = a² + b^² ,Using this theorem, we can derive Pythagorean identities in trigonometry, which allow us to convert one trigonometric ratio into another. These identities are fundamental in simplifying and solving trigonometric equations.

• sin²θ + cos^²θ = 1
• sec²θ – tan²θ = 1
• csc²θ – cot²θ = 1

Trigonometric Ratio Table

Periodicity Identities (in Radians):

These formulas are used to shift the angles by π/2, π, 2π, etc. They are also called co-function identities.

• sin (π/2 – A) = cos A & cos (π/2 – A) = sin A
• sin (π/2 + A) = cos A & cos (π/2 + A) = – sin A
• sin (3π/2 – A)  = – cos A & cos (3π/2 – A)  = – sin A
• sin (3π/2 + A) = – cos A & cos (3π/2 + A) = sin A
• sin (π – A) = sin A &  cos (π – A) = – cos A
• sin (π + A) = – sin A & cos (π + A) = – cos A
• sin (2π – A) = – sin A & cos (2π – A) = cos A
• sin (2π + A) = sin A & cos (2π + A) = cos A

Cofunction Identities (in Degrees):

• sin(90°−x) = cos x
• cos(90°−x) = sin x
• tan(90°−x) = cot x
• cot(90°−x) = tan x
• sec(90°−x) = cosec x
• cosec(90°−x) = sec x

Sum & Difference Identities

• sin(x+y) = sin(x)cos(y)+cos(x)sin(y)
• cos(x+y) = cos(x)cos(y)–sin(x)sin(y)
• 𝑡𝑎𝑛(𝑥+𝑦)= (𝑡𝑎𝑛 𝑥+𝑡𝑎𝑛𝑦) / (1−𝑡𝑎𝑛 𝑥.𝑡𝑎𝑛 𝑦)
• sin(x–y) = sin(x)cos(y)–cos(x)sin(y)
• cos(x–y) = cos(x)cos(y) + sin(x)sin(y)
• tan(x-y) = (tan x – tan y) (1+tan x.tan y)

Triple Angle Identities

• Sin 3x = 3sin x – 4sin³x
• Cos 3x = 4cos³x-3cos x
• Tan 3x = (3tanx – tan³x)/(1-3tan²x)

Inverse Trigonometry Formulas

• sin⁻¹ (–x) = – sin⁻¹ x
• cos⁻¹ (–x) = π – cos⁻¹ x
• tan⁻¹ (–x) = – tan⁻¹ x
• cosec⁻¹ (–x) = – cosec^⁻¹ x
• sec⁻¹ (–x) = π – sec⁻¹ x
• cot^⁻¹ (–x) = π – cot⁻¹ x

Trigonometric Sign Functions

• sin (-θ) = − sin θ
• cos (−θ) = cos θ
• tan (−θ) = − tan θ
• cosec (−θ) = − cosec θ
• sec (−θ) = sec θ
• cot (−θ) = − cot θ

Trigonometry Formulas For Class 10

Trigonometric Sign Functions

• sin (-θ) = − sin θ
• cos (−θ) = cos θ
• tan (−θ) = − tan θ
• cosec (−θ) = − cosec θ
• sec (−θ) = sec θ
• cot (−θ) = − cot θ

Trigonometric Identities

1. sin²A + cot²A = 1
2. tan²A + 1 = sec²A
3. cot²A + 1 = cosec²A

Periodic Identities

• sin(2nπ + θ ) = sin θ
• cos(2nπ + θ ) = cos θ
• tan(2nπ + θ ) = tan θ
• cot(2nπ + θ ) = cot θ
• sec(2nπ + θ ) = sec θ
• cosec(2nπ + θ ) = cosec θ

Complementary Ratios

Quadrant I

• sin(π/2 − θ) = cos θ
• cos(π/2 − θ) = sin θ
• tan(π/2 − θ) = cot θ
• cot(π/2 − θ) = tan θ
• sec(π/2 − θ) = cosec θ
• cosec(π/2 − θ) = sec θ

Quadrant II

• sin(π − θ) = sin θ
• cos(π − θ) = -cos θ
• tan(π − θ) = -tan θ
• cot(π − θ) = – cot θ
• sec(π − θ) = -sec θ
• cosec(π − θ) = cosec θ

Quadrant III

• sin(π + θ) = – sin θ
• cos(π + θ) = – cos θ
• tan(π + θ) = tan θ
• cot(π + θ) = cot θ
• sec(π + θ) = -sec θ
• cosec(π + θ) = -cosec θ

Quadrant IV

• sin(2π − θ) = – sin θ
• cos(2π − θ) = cos θ
• tan(2π − θ) = – tan θ
• cot(2π − θ) = – cot θ
• sec(2π − θ) = sec θ
• cosec(2π − θ) = -cosec θ

Sum and Difference of Two Angles

• sin (A + B) = sin A cos B + cos A sin B
• sin (A − B) = sin A cos B – cos A sin B
• cos (A + B) = cos A cos B – sin A sin B
• cos (A – B) = cos A cos B + sin A sin B
• tan(A + B) = [(tan A + tan B)/(1 – tan A tan B)]
• tan(A – B) = [(tan A – tan B)/(1 + tan A tan B)]

Double Angle Formulas

• sin 2A = 2 sin A cos A = [2 tan A /(1 + tan²A)]
• cos 2A = cos²A – sin²A = 1 – 2 sin²A = 2 cos²A – 1 = [(1 – tan²A)/(1 + tan²A)]
• tan 2A = (2 tan A)/(1 – tan²A)

Triple Angle Formulas

• sin 3A = 3 sinA – 4 sin³A
• cos 3A = 4 cos³A – 3 cos A
• tan 3A = [3 tan A – tan³A]/[1 − 3 tan²A]

FAQs