Drag Force Formula

What is Drag Force Formula?

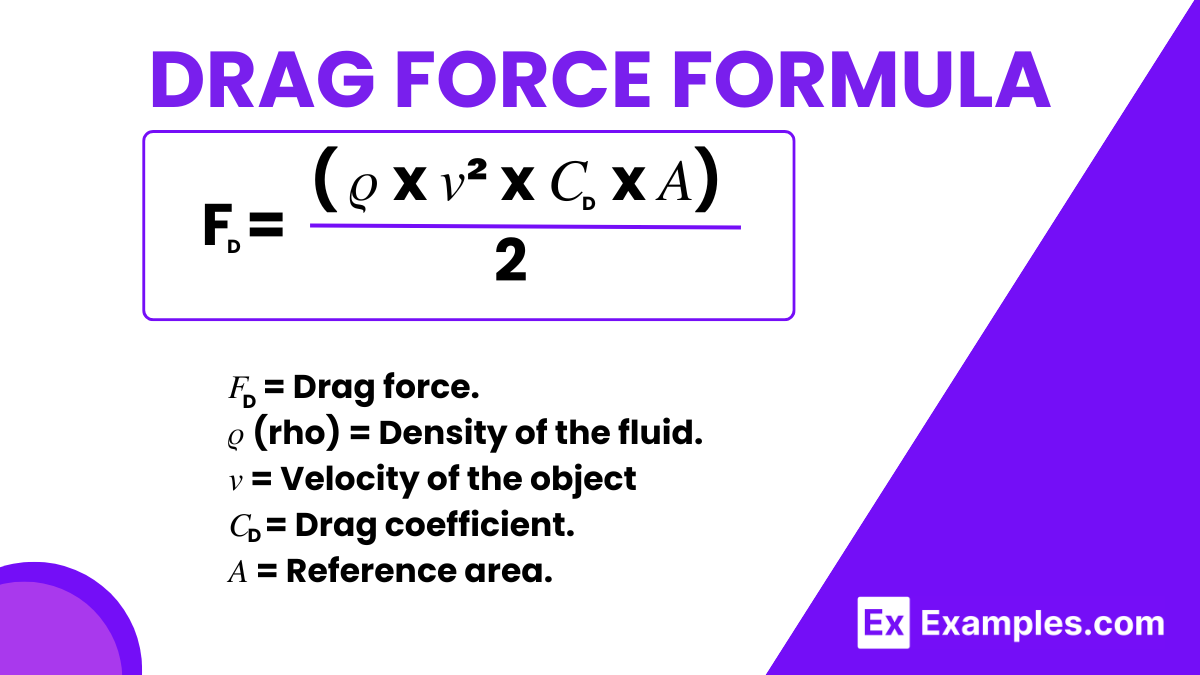
The drag force formula is a fundamental equation in physics that quantifies the resistance experienced by an object as it moves through a fluid, such as air or water. The formula was derived from principles laid down by Sir George Stokes in the mid-19th century, although the concept of drag itself was studied by earlier scientists like Leonardo da Vinci. The drag force formula is crucial for calculating how much force opposes an object’s motion, affecting everything from the design of aircraft to the speed of swimmers. The formula is represented as :

𝐹ₔ= ( 𝜌 x 𝑣²x 𝐶ₔ x 𝐴 ) / 2

• 𝐹ₔ is the drag force,
• 𝜌 (rho) represents the density of the fluid,
• 𝑣 is the velocity of the object relative to the fluid,
• 𝐶ₔ​ is the drag coefficient, which depends on the shape of the object and the nature of the flow,
• 𝐴 is the reference area (the frontal area of the object facing the fluid).

This formula enables engineers and scientists to predict how much drag an object will experience under different conditions, which is vital for optimizing performance and efficiency in a variety of fields within physics. By adjusting parameters like shape (to alter 𝐶ₔ​) or size (to alter 𝐴), designers can influence how an object interacts with its fluid environment.

Applications of Drag Force Formula

1. Aerospace Engineering: Engineers design aircraft shapes that minimize drag to enhance fuel efficiency and performance during flight.
2. Automotive Design: Automakers apply the formula to create car designs that reduce air resistance, thereby improving speed and reducing fuel consumption.
3. Sports Equipment Design: The formula helps in designing sports gear, such as golf balls and racing bicycles, to reduce drag and maximize performance.
4. Environmental Engineering: It is used in wind turbine design to optimize the shapes of blades for maximum energy efficiency from wind.
5. Urban Planning: City planners use the drag force formula to determine how wind flows between buildings, affecting everything from wind comfort to the dispersal of pollutants.
6. Marine Engineering: Shipbuilders apply the formula to design hulls of ships and underwater vehicles that move through water with reduced resistance.

Example Problems on Drag Force Formula

Problem 1: Calculating Drag Force on a Car

Question: A car is traveling at a speed of 27 m/s (about 60 mph) through air with a density of 1.225 kg/m³. The frontal area of the car is 2.2 m², and its drag coefficient is 0.3. Calculate the drag force acting on the car.

Solution: Use the drag force formula: 𝐹ₔ = ( 𝜌 x 𝑣² x 𝐶ₔ x 𝐴 ) / 2

Plug in the values: 𝐹𝐷=( 1.225 × (27)² × 0.3 × 2.2 ) / 2

𝐹𝐷 ≈ ( 1.225 × 729 × 0.3 × 2.2 ) / 2

𝐹𝐷≈183.36525 N

Thus, the drag force on the car is approximately 183.37 newtons.

Problem 2: Finding the Velocity for a Given Drag Force

Question: A skydiver with a frontal area of 0.7 m² and a drag coefficient of 1.0 falls through the air (density = 1.2 kg/m³). What velocity must the skydiver reach for the drag force to be 800 N?

Solution: Rearrange the formula to solve for velocity 𝑣v: 𝐹ₔ = (𝜌 x 𝑣² x 𝐶ₔ x 𝐴) / 2

800 = ( 1.2 × 𝑣² × 1.0 × 0.7 ) / 2

800=0.42×𝑣²

𝑣² =8000.42

𝑣 ≈ √1904.76

𝑣≈43.64 m/s

Therefore, the skydiver must reach a velocity of about 43.64 m/s to experience a drag force of 800 newtons.

Problem 3: Effect of Changing Area on Drag Force

Question: If the frontal area of a bicycle and rider is increased by 50% from 0.5 m² to 0.75 m² while traveling at 12 m/s, and the drag coefficient remains constant at 0.9 with air density at 1.225 kg/m³, calculate the new drag force.

Solution: Calculate the initial drag force with the original area:

𝐹𝐷 = (1.225 × (12)² × 0.9 × 0.5 ) / 2

𝐹𝐷 = ( 1.225 × 144 × 0.9 × 0.5 ) / 2

𝐹𝐷=39.78 N

Now calculate with the increased area:

𝐹𝐷 = ( 1.225 × (12)² × 0.9 × 0.75 ) / 2

𝐹𝐷=59.67 N

Thus, with an increased area, the new drag force is approximately 59.67 newtons, showing how sensitive drag force is to changes in area.

FAQs

How Do You Calculate Drag from Force?

Use the drag force formula: 𝐹D = ( 𝜌 x 𝑣² x 𝐶𝐷 x A ) / 2. By substituting values for air density (𝜌), velocity (𝑣), drag coefficient (𝐶D​), and area (𝐴).

What Is the Formula for Lift and Drag?

For lift: 𝐿 = ( 𝜌 x 𝑣² x 𝐶𝐿 x 𝐴 ) / 2. For drag: 𝐷 = ( 𝜌 x 𝑣² x 𝐶𝐷 x A ) / 2. 𝐶𝐿​ is the lift coefficient.

How Do You Calculate Drag from Weight?

Drag isn’t directly calculated from weight. It depends on velocity, fluid density, drag coefficient, and object area. By using 𝐹𝐷 = ( 𝜌 x 𝑣² x 𝐶𝐷 x 𝐴 ) / 2.