Equations

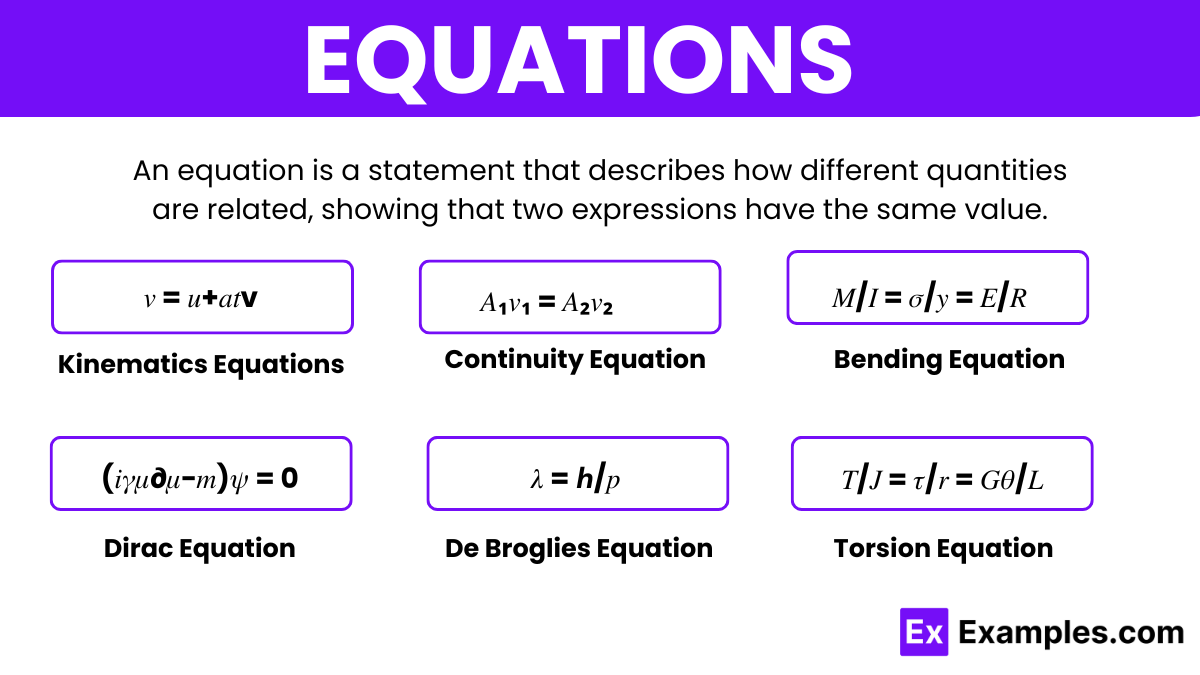
Equations are mathematical statements that assert the equality of two expressions. They play a fundamental role in various fields of science and engineering. An equation consists of two sides, typically separated by an equal sign (=), indicating that the quantities on both sides are equal. Equations can be as simple as (x + 2 = 5), where the solution (x = 3) satisfies the equation, or as complex as Einstein’s mass-energy equivalence formula (E = mc²).

Equations are used to describe relationships between variables and constants, model real-world phenomena, and solve problems ranging from basic arithmetic to advanced calculus. In algebra, equations are used to find unknown values, while in physics, they describe laws of nature. Understanding how to manipulate and solve equations is essential for studying mathematics and its applications in various disciplines.

Kinematics Equations

Kinematics equations describe the motion of objects without considering the forces causing the motion. These equations are fundamental in physics, helping to predict future positions and velocities of moving objects. They are essential for solving problems related to uniform and accelerated motion.

A key kinematics equation is: 𝑣=𝑢+𝑎𝑡v

Derivation of Van Der Waals Equation

The Van der Waals equation modifies the Ideal Gas Law to account for real gas behavior, considering the finite size of gas molecules and intermolecular attractions. Derived by Johannes Diderik van der Waals in 1873, it corrects the Ideal Gas Law by introducing two parameters: 𝑎a, representing intermolecular forces, and 𝑏b, accounting for the volume occupied by gas molecules. This equation provides a more accurate description of gas behavior under non-ideal conditions.

The Van der Waals equation is: (𝑃+𝑎/𝑉²)(𝑉−𝑏)=𝑅𝑇

Continuity Equation

The Continuity Equation is a fundamental principle in fluid dynamics, expressing the conservation of mass in a fluid flow. It states that the mass flow rate of a fluid must remain constant from one cross-section of a pipe to another if the fluid is incompressible. This equation ensures that fluid mass is neither created nor destroyed within a flow system, which is crucial for analyzing fluid behavior in various engineering applications.

The Continuity Equation is: 𝐴₁𝑣₁=𝐴₂𝑣₂

Derivation of Bending Equation

The Bending Equation describes the relationship between the bending moment, the moment of inertia, the curvature, and the stress in a beam subjected to bending. It is derived from the theory of elasticity and beam theory, which considers how beams deform under various loads. This equation is crucial in structural engineering for designing beams to ensure they can withstand applied loads without excessive deformation or failure.

The Bending Equation is: 𝑀/𝐼=𝜎/𝑦=𝐸/𝑅​

Navier StokesEquation

The Navier-Stokes equation describes the motion of viscous fluid substances, such as liquids and gases. This fundamental equation in fluid dynamics accounts for various forces acting on the fluid, including pressure, viscous, and external forces. Derived from Newton’s second law of motion, it helps predict how fluids flow under different conditions and is essential for understanding weather patterns, ocean currents, and airflow over aircraft wings.

The Navier-Stokes equation in its most general form is: 𝜌(∂𝑢/∂𝑡+(𝑢⋅∇)𝑢) = −∇𝑝+𝜇∇²𝑢+𝑓

Derivation of Torsion Equation

The Torsion Equation describes the relationship between torque, shear stress, and the geometrical properties of a circular shaft subjected to twisting. Derived from the theory of elasticity, it helps in determining the stress distribution within the shaft and is crucial for designing mechanical components like drive shafts and axles to ensure they can withstand applied torsional loads without failing.

The Torsion Equation is: 𝑇/𝐽=𝜏/𝑟=𝐺𝜃/𝐿​

Dirac Equation

The Dirac Equation is a fundamental equation in quantum mechanics formulated by Paul Dirac in 1928. It describes the behavior of relativistic spin-½ particles, such as electrons, and incorporates both quantum mechanics and special relativity. This equation successfully predicted the existence of antimatter and provided a theoretical framework for understanding the intrinsic angular momentum (spin) of particles. It is crucial for studying the behavior of particles at high energies and has profound implications in particle physics and quantum field theory.

The Dirac Equation is: (𝑖𝛾𝜇∂𝜇−𝑚)𝜓 = 0

Einstein Field Equation

The Einstein Field Equation is the cornerstone of Albert Einstein’s General Theory of Relativity, formulated in 1915. It describes how matter and energy influence the curvature of spacetime, which we perceive as gravity. This equation revolutionized our understanding of gravity, showing it as a geometric property of spacetime rather than a force. The equation is essential for studying cosmology, black holes, and the dynamics of astronomical objects.

The Einstein Field Equation is: 𝑅𝜇𝜈−1/2𝑅𝑔𝜇𝜈+Λ𝑔𝜇𝜈 = 8𝜋𝐺𝑐⁴𝑇𝜇𝜈​

Wave Nature of Matter and De Broglies Equation

The wave nature of matter and De Broglie’s equation revolutionized our understanding of quantum mechanics. According to De Broglie, particles such as electrons exhibit wave-like properties. His equation, λ = h/p, where λ is the wavelength, h is Planck’s constant, and p is the momentum, demonstrates that every moving particle has an associated wavelength. This concept, known as wave-particle duality, bridges the gap between classical and quantum physics, explaining phenomena like electron diffraction. De Broglie’s theory underpins much of modern physics, influencing the development of wave mechanics and the study of quantum behavior in particles.

De Broglie’s Equation is: 𝜆=ℎ/𝑝​

Derivation of One Dimensional Wave Equation

The one-dimensional wave equation describes how waves propagate through a medium in a single spatial dimension. It is fundamental in various fields, including acoustics, electromagnetics, and fluid dynamics. Derived from Newton’s second law and Hooke’s law, it models the relationship between the displacement of points in the medium and time. This equation helps in understanding wave behavior, such as speed, frequency, and wavelength.

The One-Dimensional Wave Equation is: ∂²𝑦/∂𝑡² = 𝑣²∂²𝑦/∂𝑥²​

Boltzmann Equation

The Boltzmann Equation, formulated by Ludwig Boltzmann in 1872, describes the statistical behavior of a thermodynamic system not in equilibrium. It provides a link between the microscopic properties of individual particles and the macroscopic properties of the system, such as temperature and pressure. The equation is essential for studying the dynamics of gases, predicting how they evolve over time due to collisions and external forces.

The Boltzmann Equation is: ∂𝑓/∂𝑡+𝑣⋅∇𝑓+𝑎⋅∂𝑓/∂𝑣 = (∂𝑓/∂𝑡)꜀ₒₗₗ​

Faqs