Solve the equation 2x + 5 = 13.
x = 3
x = 4
x = 5
x = 6

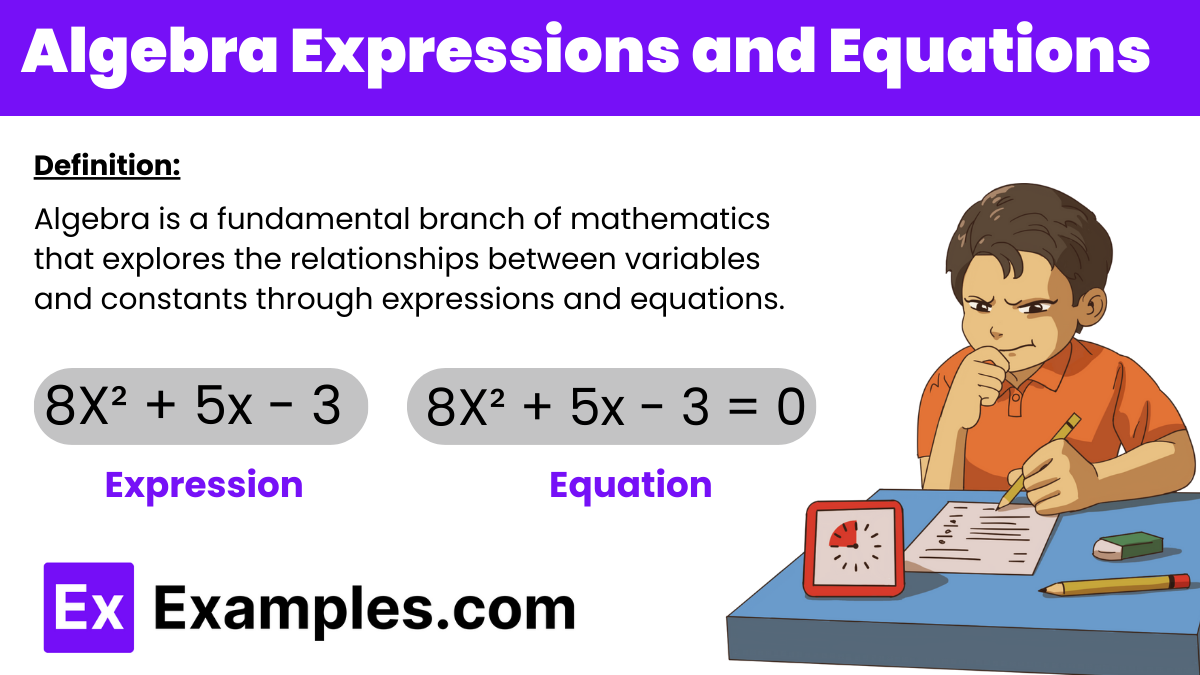
Algebra is a fundamental branch of mathematics that explores the relationships between variables and constants through expressions and equations. Algebra expressions consist of terms combined using operations like addition, subtraction, multiplication, and division, often containing variables that represent unknown values. Equations, on the other hand, set two expressions equal to each other, forming the basis for solving problems by finding the values of these variables. This field is essential in developing critical thinking and problem-solving skills, applicable in various real-world contexts from engineering to economics. Understanding how to manipulate and solve these algebraic expressions and equations is crucial for students and professionals alike
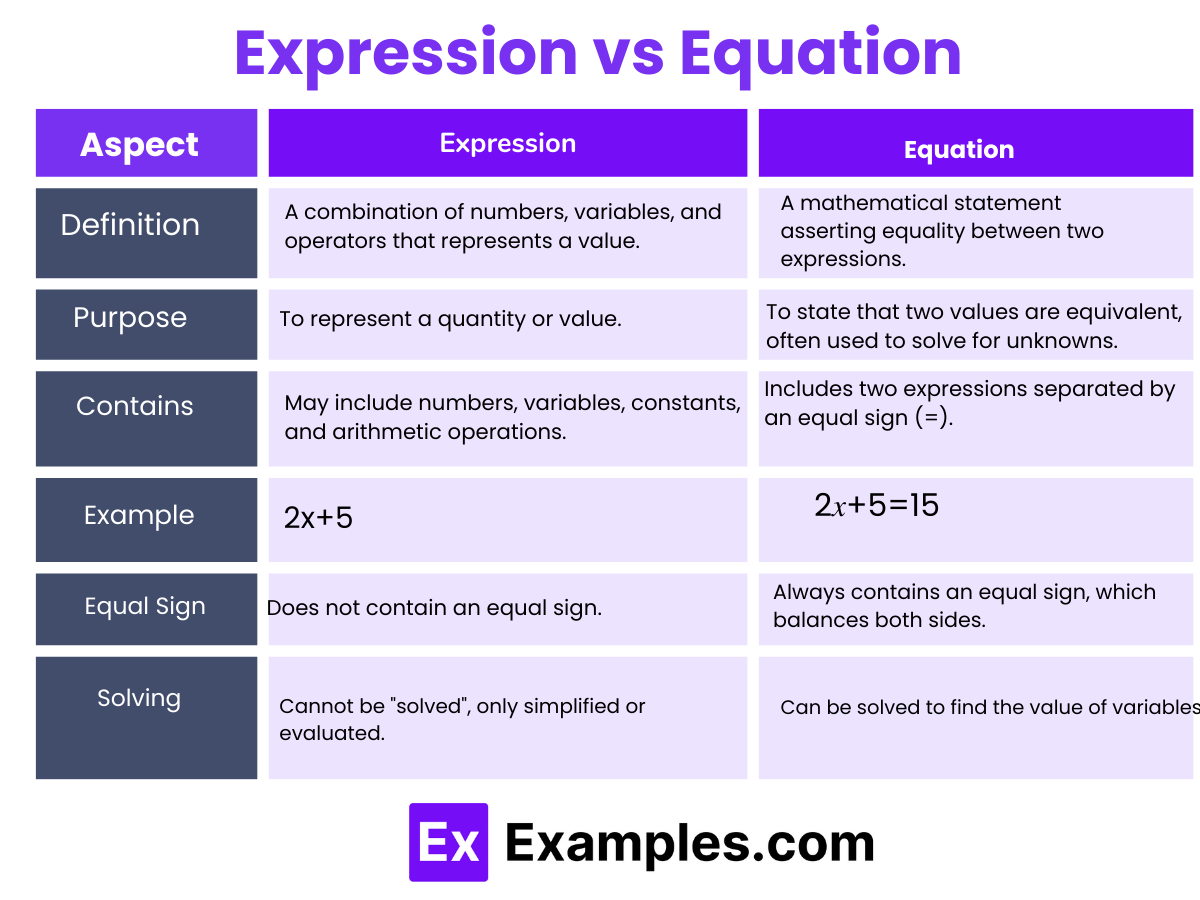
In mathematics, particularly in algebra, the terms “expressions” and “equations” play fundamental roles and serve different functions within problem-solving contexts. Understanding each and their relationship can greatly enhance your ability to manipulate and solve algebraic problems. Here’s a breakdown:
An algebraic expression is a combination of variables, numbers, and operations (such as addition, subtraction, multiplication, and division). Expressions do not contain equal signs; instead, they represent values that can be simplified but not solved. For example, the expression 2x+5 combines the variable xxx with numbers through multiplication and addition. Algebraic expressions can be as simple as a single term, like 5x5x5x, or more complex with multiple terms, like 3x²−4x+7
An algebraic equation, on the other hand, is a statement that asserts the equality of two expressions, featuring an equal sign (=). Equations are solvable, meaning you can manipulate them to find the value(s) of the variable(s) that make the equation true. For instance, the equation 2x+5=15 can be solved by finding the value of x that balances both sides of the equal sign.
Both algebraic expressions and equations are used to describe relationships between quantities and to solve problems. Expressions can be part of equations; they can be evaluated given certain values, simplified, or even transformed into equations by including an equality to another expression. Equations can be solved to find unknown values, analyzed, and applied in various practical scenarios like physics, engineering, and economics.
Solving algebraic equations is a systematic process that involves manipulating the equation to isolate the variable and simplify the expression. Here’s a step-by-step guide on how to approach solving algebraic equations:
Simplify Both Sides: Start by simplifying both sides of the equation. Combine like terms and simplify any algebraic expressions.
Isolate the Variable: Use addition or subtraction to move terms that contain the variable to one side of the equation and all constant terms to the other side.
Undo Multiplication or Division: If the variable is multiplied by a coefficient or divided, use the opposite operation to isolate the variable. For multiplication, divide both sides by the same number, and for division, multiply both sides.
Check for More Solutions: Some equations, like quadratic equations, may have more than one solution. Be sure to explore all potential solutions.
Verify Your Solution: Substitute the solution back into the original equation to verify that it works. This step ensures that the solution is correct and that no errors were made during the process.
Consider Special Cases: Be aware of special cases, such as no solution (when the equation simplifies to a contradiction, like 3=23) or infinite solutions (when the equation simplifies to a tautology, like 0=00).
Algebraic expressions and equations form the backbone of algebra, each serving a specific purpose in mathematical problem-solving. Understanding the distinction between them and how to handle each is crucial in mastering algebra.
An algebraic expression is a combination of variables, numbers, and arithmetic operations (such as addition, subtraction, multiplication, and division). It does not include an equality sign, so it cannot be solved but can be simplified. Expressions are used to represent values and can be part of broader mathematical formulas or functions.
Purpose: To represent relationships or formulas involving variables without asserting equality.
An algebraic equation, on the other hand, involves variables and numbers connected by arithmetic operations, but crucially, it includes an equality sign. This equality defines a problem that needs solving: finding the value(s) of the variables that make the equation true.
Purpose: To solve for unknown values, usually represented by variables, that satisfy the equality condition set by the equation.
Expressions:
Equations:
To illustrate, consider an equation and an expression:
For the expression, you might be asked to simplify or evaluate it for a specific value of x. For the equation, you would solve it to find the value of xxx that makes it true. Solving 3x+5=11 involves isolating x by first subtracting 5 from both sides, resulting in 3x=6, and then dividing both sides by 3 to find x=2
Start by expanding the expression, using the distributive property to eliminate parentheses. Multiply each term inside the parentheses by the term outside, if applicable.
Example: a(b+c)=ab+ac
Like terms are terms that contain the same variables raised to the same power. Add or subtract coefficients of like terms to simplify the expression.
Example: 3x+4x=7x
2y²+5−3y²+1=−y²+6
If there are any coefficients that can be simplified (for example, if they are fractions), do this to make the expression cleaner.
Example:
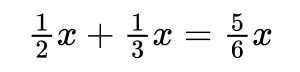
Order the terms in a standardized form, usually from highest to lowest degree of the variable (for example, in descending powers of x). This step isn’t necessary for simplification per se, but it helps in readability and is standard practice.
Example: x²+2x−x²+3→2x+3
If there’s a common factor in all terms, factor it out. This is particularly useful if the expression is part of an equation.
Example: 4x²−8x=4x(x−2)
If the expression includes fractions, simplify them by finding the greatest common divisor for the numerator and denominator or by rationalizing the denominator if needed.
Example: 4x/8x²=1/2x
Look for opportunities to use special products or identities (like squares of binomials or difference of squares) to further simplify the expression.
Example: x²−9=(x+3)(x−3)
The five basic algebraic expressions include monomials (3x), binomials (3x + 4), trinomials (x² + 2x + 1), polynomials (2x³ + 3x² + x + 4), and rational expressions ((x+1)/(x-1)).
The five types of algebraic expressions are monomials, binomials, trinomials, polynomials, and rational expressions. Each type varies in the number of terms and complexity of the operations involved.
The 20 algebraic identities include essential formulas like (a + b)² = a² + 2ab + b², (a – b)² = a² – 2ab + b², and a² – b² = (a + b)(a – b), among others, used to simplify and solve equations.
The four basic algebra formulas are:
a² – b² = (a + b)(a – b)
(a + b)² = a² + 2ab + b²
(a – b)² = a² – 2ab + b²
(a + b)(a – b) = a² – b²
Examples of basic algebraic expressions include 3x + 2, 5y – 7, x² + 4x + 4, 7a – 3b + 5, and 4x² – 9. These expressions consist of variables, constants, and operations.
Three examples of algebraic expressions are 2x + 5, 4y – 7, and x² – 3x + 2. These expressions represent different combinations of variables and constants.
To write basic algebraic expressions, identify the variables, determine the constants and coefficients, and combine them using operations such as addition, subtraction, multiplication, and division. For example, 3x + 4y – 5.
To solve an algebra equation, combine like terms, use inverse operations to isolate the variable, simplify both sides of the equation, and check the solution. For example, to solve 2x + 3 = 7, subtract 3 and divide by 2.
To simplify algebra expressions, combine like terms, use the distributive property, reduce fractions, and simplify radicals if present. For instance, 2x + 3x simplifies to 5x.
An example of an expression is 2x + 3, while an equation is 2x + 3 = 7. An expression shows a mathematical phrase, whereas an equation shows a mathematical statement with equality.
To do algebra easily, understand the basics, practice regularly, break problems into smaller steps, and use algebraic rules and formulas. Use resources like textbooks, online tutorials, and practice problems to enhance skills.
To calculate algebraic expressions, substitute the given values for variables, perform the operations according to the order of operations (PEMDAS), and simplify the result. For example, for 3x + 4 when x = 2, calculate 3(2) + 4 = 6 + 4 = 10.
Terms in an equation are the individual components separated by addition or subtraction signs. Each term consists of constants, coefficients, and variables. For example, in the equation 3x + 5 = 11, 3x and 5 are terms.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Solve the equation 2x + 5 = 13.
x = 3
x = 4
x = 5
x = 6
Simplify the expression 3(2x - 4) + 5.
6x - 7
6x - 12
6x - 9
6x - 10
What is the value of x if 4(x - 2) = 3x + 6?
x = 0
x = 2
x = 6
x = 12
Solve for y in the equation 5y - 3 = 2y + 9.
y = 4
y = 6
y = 8
y = 10
Solve for x: 7 - 2x = 3x + 12.
x = -1
x = 2
x = -2
x = 1
If 3x - 4 = 2(x + 1), what is x?
x = -1
x = 0
x = 1
x = 2
Simplify the expression (2x + 3) - (x - 4).
x + 7
x - 1
3x + 7
3x - 1
What is the value of x if 2(x + 5) = 4x + 6?
x = 2
x = 4
x = 6
x = 8
Solve the equation 8 - 3x = 2x + 1.
x = 1
x = -1
x = 2
x = -2
If 4x + 7 = 3x - 5, what is x?
x = -12
x = -6
x = 6
x = 12
Before you leave, take our quick quiz to enhance your learning!

