What is the cube root of 27?
2
3
4
5
of 10
Cube Root 1-40
Cube Root 1-40
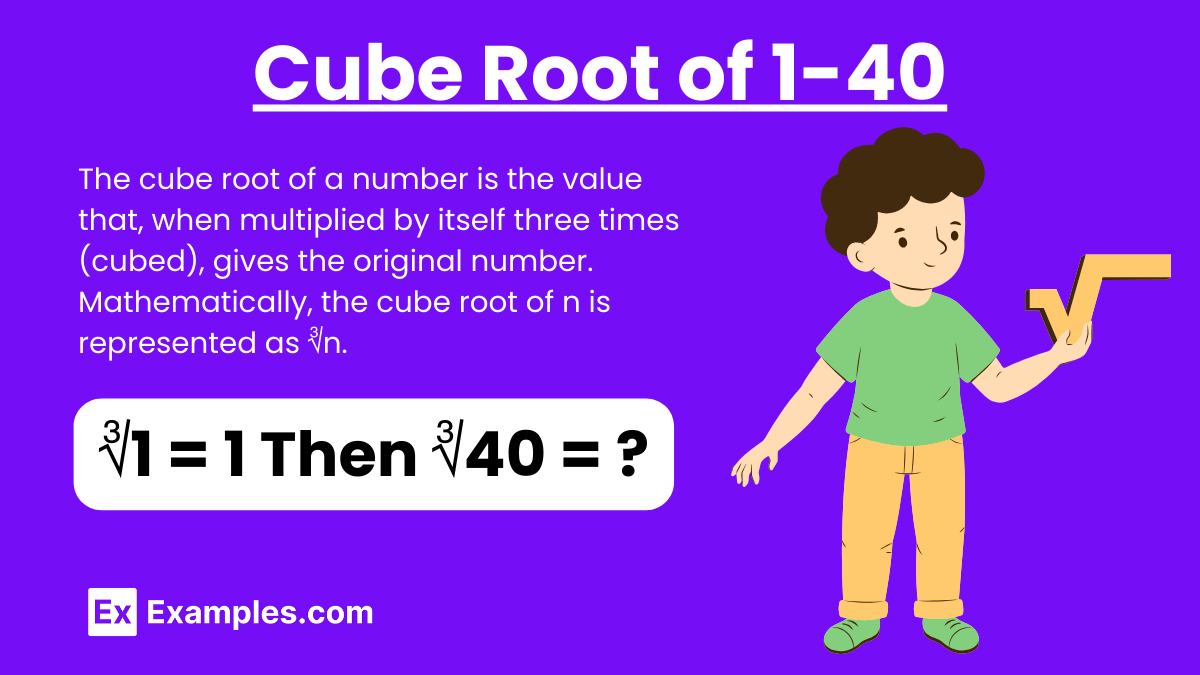
The cube root of a number is the value that, when multiplied by itself three times (cubed), gives the original number. Mathematically, the cube root of n is represented as ∛n​. For example, the cube root of 27 is 3, because 3×3×3=27.
Learning the cube roots of these numbers helps build a strong foundation in algebra and calculus, providing insight into how numbers and their powers relate. This knowledge is beneficial for students, educators, and professionals in fields such as engineering, physics, and computer science, where the manipulation and understanding of numbers are essential.
The following table provides the cube roots of numbers from 1 to 40, expressed both numerically and in words, to facilitate easy reference and comprehension.
Download Cube Root 1-40 in PDF
Cube Root 1-40
Download Cube Root 1-40 in PDF
| Cube Root of 1-40 Values | In Number | In Words |
|---|---|---|
| ∛1 | 1 | One |
| ∛2 | 1.2599 | One point two five nine nine |
| ∛3 | 1.4422 | One point four four two two |
| ∛4 | 1.5874 | One point five eight seven four |
| ∛5 | 1.7100 | One point seven one zero zero |
| ∛6 | 1.8171 | One point eight one seven one |
| ∛7 | 1.9129 | One point nine one two nine |
| ∛8 | 2 | Two |
| ∛9 | 2.0801 | Two point zero eight zero one |
| ∛10 | 2.1544 | Two point one five four four |
| ∛11 | 2.2239 | Two point two two three nine |
| ∛12 | 2.2894 | Two point two eight nine four |
| ∛13 | 2.3513 | Two point three five one three |
| ∛14 | 2.4101 | Two point four one zero one |
| ∛15 | 2.4662 | Two point four six six two |
| ∛16 | 2.5198 | Two point five one nine eight |
| ∛17 | 2.5713 | Two point five seven one three |
| ∛18 | 2.6207 | Two point six two zero seven |
| ∛19 | 2.6684 | Two point six six eight four |
| ∛20 | 2.7144 | Two point seven one four four |
| ∛21 | 2.7589 | Two point seven five eight nine |
| ∛22 | 2.8020 | Two point eight zero two zero |
| ∛23 | 2.8439 | Two point eight four three nine |
| ∛24 | 2.8845 | Two point eight eight four five |
| ∛25 | 2.9240 | Two point nine two four zero |
| ∛26 | 2.9625 | Two point nine six two five |
| ∛27 | 3 | Three |
| ∛28 | 3.0366 | Three point zero three six six |
| ∛29 | 3.0723 | Three point zero seven two three |
| ∛30 | 3.1072 | Three point one zero seven two |
| ∛31 | 3.1414 | Three point one four one four |
| ∛32 | 3.1748 | Three point one seven four eight |
| ∛33 | 3.2075 | Three point two zero seven five |
| ∛34 | 3.2396 | Three point two three nine six |
| ∛35 | 3.2711 | Three point two seven one one |
| ∛36 | 3.3019 | Three point three zero one nine |
| ∛37 | 3.3322 | Three point three three two two |
| ∛38 | 3.3617 | Three point three six one seven |
| ∛39 | 3.3906 | Three point three nine zero six |
| ∛40 | 3.4190 | Three point four one nine zero |
Understanding the cube roots of numbers from 1 to 40 provides valuable insights into the nature of cubic relationships and volume calculations. Cube roots help in solving equations involving three-dimensional shapes and are crucial in fields like engineering, physics, and computer science. This foundational knowledge aids in grasping more complex mathematical concepts and problem-solving techniques. By mastering the cube roots of these numbers, students can strengthen their mathematical skills and enhance their ability to tackle a variety of real-world applications.
Share :
Practice Test
If the cube root of a number is 5, what is the number?
25
50
125
200
of 10
What is the cube root of 64?
3
4
5
6
of 10
Find the cube root of 1728.
10
12
14
16
of 10
Which number has a cube root of 15?
3375
1968
1000
1728
of 10
Which number has a cube root of 16?
1728
1000
3000
4096
of 10
What is the cube root of 729?
8
9
10
11
of 10
What is the cube root of 27?
3
6
7
8
of 10
Which number has a cube root of 22?
10648
8000
5000
1728
of 10
Which number has a cube root of 40?
64
640
6400
64000
of 10