Which of the following numbers is divisible by 6?
123
246
135
789

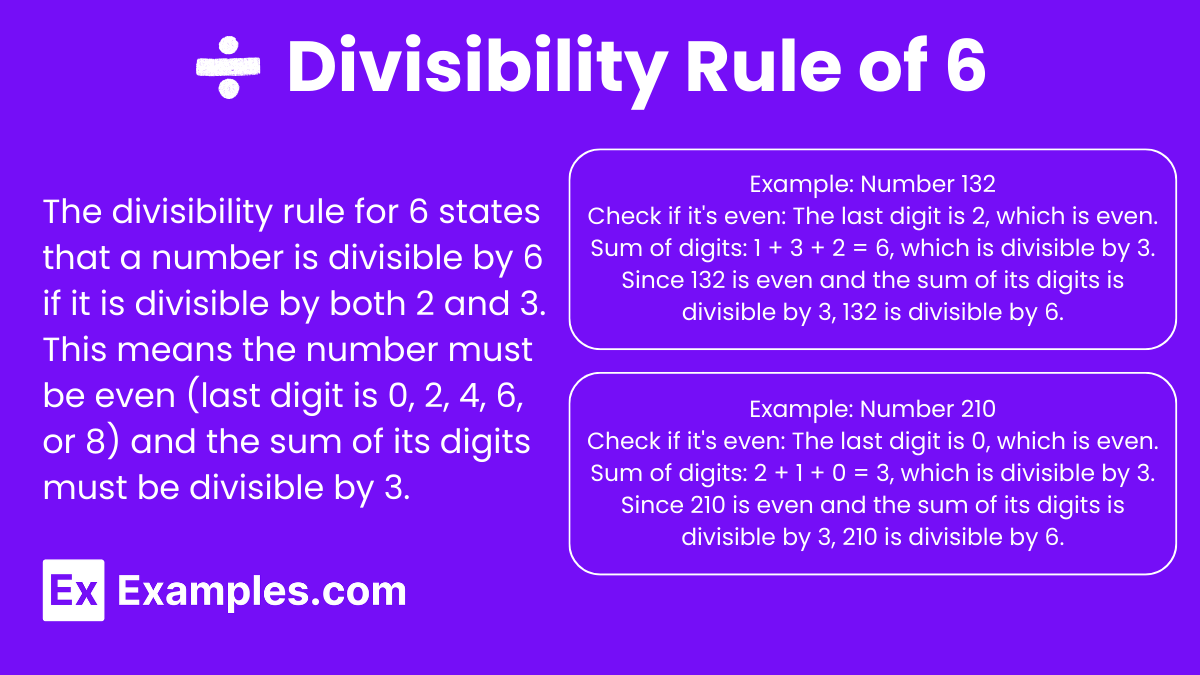
The divisibility rule for 6 states that a number is divisible by 6 if it is divisible by both 2 and 3, meaning the number must be even (last digit 0, 2, 4, 6, or 8) and the sum of its digits must be divisible by 3. This rule integrates the principles of addition, subtraction, multiplication, and division, providing a streamlined approach to evaluating divisibility among integers, a key subset of rational numbers. In algebra, understanding and applying the divisibility rules enhances problem-solving efficiency and simplifies equation manipulation. Since divisibility concerns only integers, this rule does not apply to irrational numbers, which cannot be expressed as fractions or ratios. Mastery of this rule is essential in foundational mathematics, facilitating the efficient breakdown and evaluation of numbers in both educational and practical contexts.
Download Proof of Divisibility Rule of 6 in PDF

Download Proof of Divisibility Rule of 6 in PDF
Let’s use the number 2436 to demonstrate the proof of the divisibility rule of 6 step-by-step:
Step 1: Consider the number 2436.
Step 2: Check for divisibility by 2 (evenness).
Step 3: Check for divisibility by 3.
Step 4: Calculate the sum of the digits.
Step 5: Check if the sum from Step 4 is divisible by 3.
Step 6: Apply the divisibility rule of 6.
Step 7: Conclusion from divisibility checks.
Step 8: Summarize the proof of divisibility by 6.
To determine whether a number is divisible by 6, it must satisfy two conditions: the number must be divisible by both 2 and 3. Here is a simple step-by-step process to check divisibility by 6:
Example: Consider the number 132:
The rule for checking divisibility by 7 is a bit more complex than for most other single-digit numbers. Here is a methodical way to determine if a number is divisible by 7:
Example: Consider the number 161:
The divisibility rule for 6 requires that a number must be divisible by both 2 and 3. Here’s how you can easily determine if a number is divisible by 6:
Example: Consider the number 234:
The divisibility rule for 9 is straightforward and involves only the sum of the digits:
Example: Consider the number 729:
Check for Evenness: The last digit of 258 is 8, which is an even number. This satisfies the first condition of being divisible by 2.
Sum of Digits: Calculate the sum of the digits in 258.
2+5+8 = 15
Check Divisibility by 3: The sum, 15, is divisible by 3 (since 15÷3 = 5).
Conclusion: Since 258 meets both conditions, it is divisible by 6.
Check for Evenness: The last digit of 612 is 2, which is even.
Sum of Digits: Calculate the sum of the digits in 612.
6+1+2 = 9
Check Divisibility by 3: The sum, 9, is divisible by 3 (since 9÷3 = 3).
Conclusion: Since 612 meets both conditions, it is divisible by 6.
Check for Evenness: The last digit of 540 is 0, which is even.
Sum of Digits: Calculate the sum of the digits in 540.
5+4+0 = 9
Check Divisibility by 3: The sum, 9, is divisible by 3.
Conclusion: Since 540 meets both conditions, it is divisible by 6.
A number must be even because 6 is a multiple of 2. Therefore, any number divisible by 6 must also be divisible by 2, which means its last digit must be even.
To determine if a number is divisible by 3, sum up all its digits. If the result is divisible by 3 (such as 3, 6, 9, 12, etc.), then the original number is also divisible by 3.
Yes, consider the number 14. It is even (last digit is 4), but the sum of its digits (1 + 4 = 5) is not divisible by 3. Thus, 14 is not divisible by 6.
The smallest number divisible by 6 is 6 itself.
Yes, the divisibility rules apply to negative numbers as well. A negative number is divisible by 6 if, when considered without its negative sign, it fulfills the conditions of being divisible by both 2 and 3.
Understanding the divisibility rule of 6 is helpful in simplifying fractions, finding common denominators, and solving problems that require factorization or checking for multiples.
Yes, it helps in determining whether 6 is a factor of a number, which is crucial in breaking down a number into its prime factors.
While both numbers require the target number to be even, for divisibility by 12, the sum of the digits must be divisible by 3 and the number formed by the last two digits must also be divisible by 4.
No, there are no exceptions. If a number meets the conditions of being even and having digits that sum to a multiple of 3, it is always divisible by 6.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which of the following numbers is divisible by 6?
123
246
135
789
Which of these numbers is not divisible by 6?
432
567
618
624
Determine which number is divisible by 6.
102
214
336
447
Which number is divisible by 6?
915
864
734
982
Which number is divisible by 6?
1234
2340
3451
4567
Find the number that is not divisible by 6.
750
876
981
120
Which of these numbers is divisible by 6?
189
252
375
468
Which number is divisible by 6?
555
642
789
891
Determine the number that is not divisible by 6.
180
242
330
450
Which of these numbers is divisible by 6?
267
312
423
525
Before you leave, take our quick quiz to enhance your learning!

