Divisibility Rule of 8
Divisibility Rule of 8
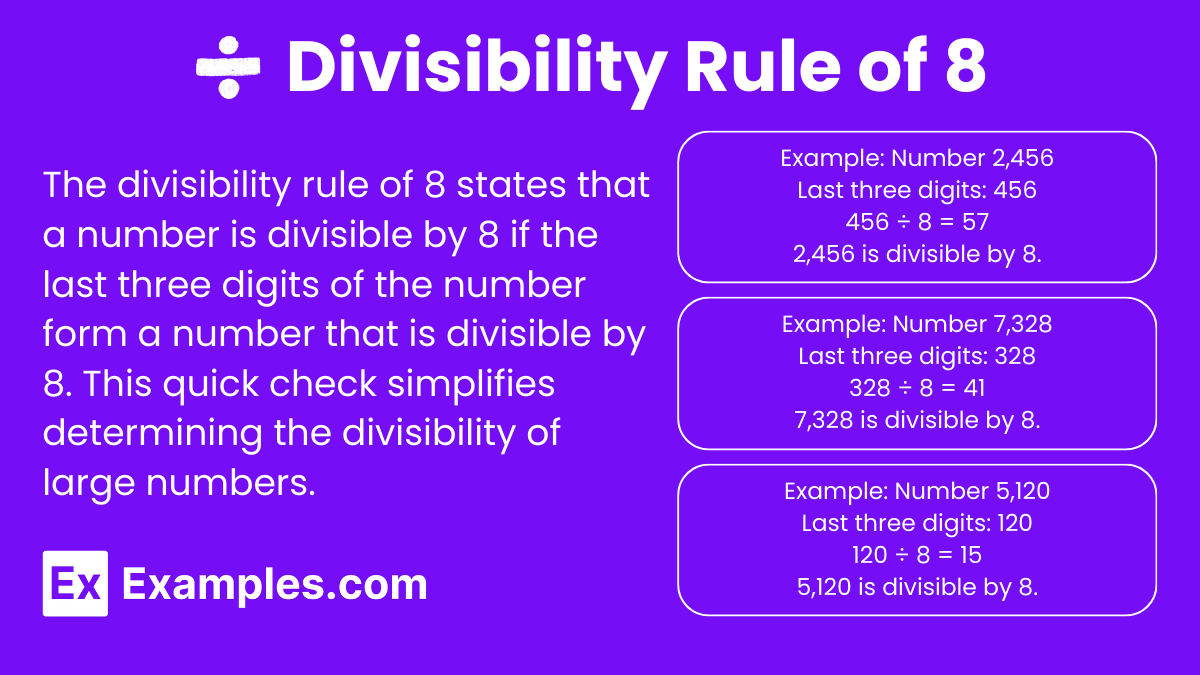
The divisibility rule of 8 states that a number is divisible by 8 if the last three digits of the number form a number that is divisible by 8. This rule simplifies checking large numbers for divisibility. In mathematics, understanding divisibility rules helps in working with various concepts such as rational and irrational numbers, integers, and algebraic expressions. Efficiently performing addition, subtraction, multiplication, and division of numbers often relies on recognizing these divisibility patterns.
Download Proof of the Divisibility Rule of 8 in PDF
What is the Divisibility Rule of 8?
Proof of the Divisibility Rule of 8
Download Proof of the Divisibility Rule of 8 in PDF
Step 1: Consider the Number: Let’s take a number N = 4728 to prove the divisibility rule of 8.
Step 2: Expand the Number: Break the number into its decimal components:
4728 = 4×1000+7×100+2×10+8×1
Step 3: Separate Last Three Digits: Isolate the last three digits (728) and consider the remaining part:
4728 = 4×1000+728
Step 4: Express 1000 in Terms of 8: Note that 1000 can be expressed as 8×125:
4×1000 = 4×(8×125) = 8×(4×125)
Step 5: Divisibility of 8’s Multiples: Since 8×(4×125)is clearly divisible by 8, the divisibility of 4728 depends only on 728.
Step 6: Check Divisibility of Last Three Digits: Now, we need to check if the last three digits, 728, are divisible by 8.
Step 7: Divide the Last Three Digits: Perform the division:
728÷8 = 91
Since 728 is exactly divisible by 8 (without remainder), 728 is divisible by 8.
Step 8: Conclude Divisibility: Thus, since the last three digits of 4728 (i.e., 728) are divisible by 8, the whole number 4728 is also divisible by 8. This confirms the divisibility rule of 8.
Divisibility Rule of 4 and 8
Divisibility Rule of 4
A number is divisible by 4 if the last two digits of the number form a number that is divisible by 4.
Example:
- For the number 1324, check the last two digits (24). Since 24 ÷ 4 = 6, 1324 is divisible by 4.
Divisibility Rule of 8
A number is divisible by 8 if the last three digits of the number form a number that is divisible by 8.
Example:
- For the number 5472, check the last three digits (472). Since 472 ÷ 8 = 59, 5472 is divisible by 8.
Divisibility Rule of 8 and 9
Divisibility Rule of 8
A number is divisible by 8 if the last three digits of the number form a number that is divisible by 8.
Example:
- For the number 7368, check the last three digits (368). Since 368 ÷ 8 = 46, 7368 is divisible by 8.
Divisibility Rule of 9
A number is divisible by 9 if the sum of its digits is divisible by 9.
Example:
- For the number 729, sum the digits: 7 + 2 + 9 = 18. Since 18 ÷ 9 = 2, 729 is divisible by 9.
Divisibility Test of 8 and 11
Divisibility Test of 8
A number is divisible by 8 if the last three digits of the number form a number that is divisible by 8.
Example:
- For the number 4968, check the last three digits (968). Since 968 ÷ 8 = 121, 4968 is divisible by 8.
Divisibility Test of 11
A number is divisible by 11 if the difference between the sum of the digits in the odd positions and the sum of the digits in the even positions is either 0 or a multiple of 11.
Example:
For the number 5831:
- Sum of digits in odd positions: 5 + 3 = 8
- Sum of digits in even positions: 8 + 1 = 9
- Difference: |8 – 9| = 1 (not divisible by 11)
Since the difference is not 0 or a multiple of 11, 5831 is not divisible by 11.
For the number 462:
- Sum of digits in odd positions: 4 + 2 = 6
- Sum of digits in even positions: 6
- Difference: |6 – 6| = 0 (divisible by 11)
Since the difference is 0, 462 is divisible by 11.
Divisibility Rule of 8 Examples
Example 1:
Number: 2,456
- Check the last three digits: 456
- Calculation: 456 ÷ 8 = 57 (an integer)
- Conclusion: 2,456 is divisible by 8.
Example 2:
Number: 7,328
- Check the last three digits: 328
- Calculation: 328 ÷ 8 = 41 (an integer)
- Conclusion: 7,328 is divisible by 8.
Example 3:
Number: 5,120
- Check the last three digits: 120
- Calculation: 120 ÷ 8 = 15 (an integer)
- Conclusion: 5,120 is divisible by 8.
Example 4:
Number: 1,672
- Check the last three digits: 672
- Calculation: 672 ÷ 8 = 84 (an integer)
- Conclusion: 1,672 is divisible by 8.
Example 5:
Number: 10,024
- Check the last three digits: 024 (or simply 24)
- Calculation: 24 ÷ 8 = 3 (an integer)
- Conclusion: 10,024 is divisible by 8.
FAQs
Why is the divisibility rule of 8 useful?
This rule simplifies the process of determining whether large numbers are divisible by 8 without performing full division, saving time and effort.
How can I quickly check if 7,256 is divisible by 8?
Check the last three digits (256). Since 256 ÷ 8 = 32, 7,256 is divisible by 8.
Does the divisibility rule of 8 apply to all numbers?
Yes, this rule applies to any whole number, regardless of its size.
Can a number with fewer than three digits be checked for divisibility by 8?
Yes, if the number has fewer than three digits, you can directly check if that number is divisible by 8. For example, 64 is divisible by 8 because 64 ÷ 8 = 8.
Is 10,248 divisible by 8?
Check the last three digits (248). Since 248 ÷ 8 = 31, 10,248 is divisible by 8.
What if the last three digits form a number that isn’t easily divisible by 8?
You can perform the division of the last three digits by 8. If the result is an integer (no remainder), then the original number is divisible by 8.
How does the divisibility rule of 8 relate to the rule for 2 and 4?
The rule for 8 is an extension: a number divisible by 8 is also divisible by 4 and 2. However, a number divisible by 2 or 4 is not necessarily divisible by 8.
Is there a pattern in numbers divisible by 8?
Yes, the pattern is that the last three digits form numbers like 000, 008, 016, 024, etc., which are all divisible by 8.
Can this rule be applied to very large numbers, like 1,234,567,888?
Yes, check the last three digits (888). Since 888 ÷ 8 = 111, 1,234,567,888 is divisible by 8.
Share :
