What is the Greatest Common Factor (GCF) of 12 and 16?
2
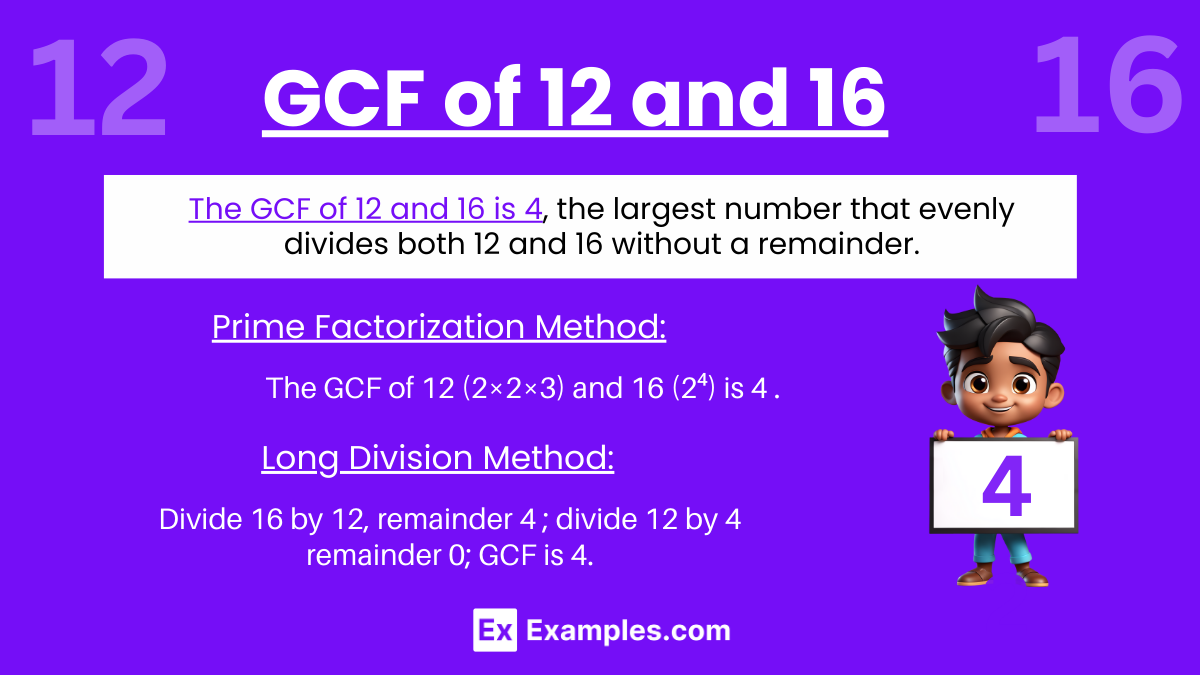
4
6
8

To find the GCF (Greatest Common Factor) of 12 and 16, we start by listing the factors of each number. The factors of 12 are 1, 2, 3, 4, 6, and 12, while the factors of 16 are 1, 2, 4, 8, and 16. The common factors between these two numbers are 1, 2, and 4. The greatest of these common factors is 4. Therefore, the GCF of 12 and 16 is 4, meaning that 4 is the largest numbers that can divide both 12 and 16 without leaving a remainder.
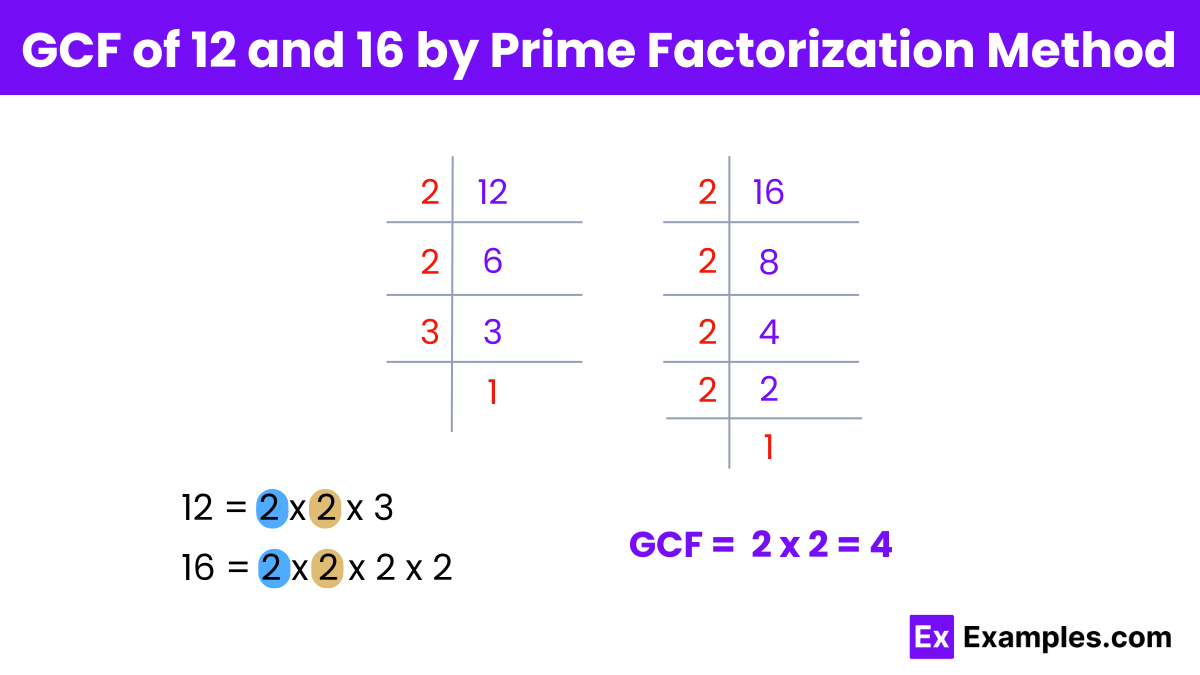
Prime Factorization:
12: The prime factors of 12 are 2 and 3.
12 = 2 × 2 × 3
16: The prime factors of 16 are all 2.
16 = 2 × 2 × 2 × 2
Identify Common Factors:
The common prime factors between 12 and 16 are 2 and 2.
Calculate the GCF:
Multiply the common prime factors together.
GCF = 2 × 2 =4
Therefore, the GCF of 12 and 16 using the prime factorization method is 4.

Divide the larger number by the smaller number:
Divide 16 by 12.
16 ÷ 12 = 1 remainder 4
Replace the larger number with the smaller number and the smaller number with the remainder:
Now, divide 12 by the remainder 4.
12 ÷ 4 = 3 remainder 0
Continue the process until the remainder is 0:
Since the remainder is now 0, the divisor at this step is the GCF.
Therefore, the GCF of 12 and 16 using the long division method is 4.
GCF of 12 and 16 by Listing Common Factors
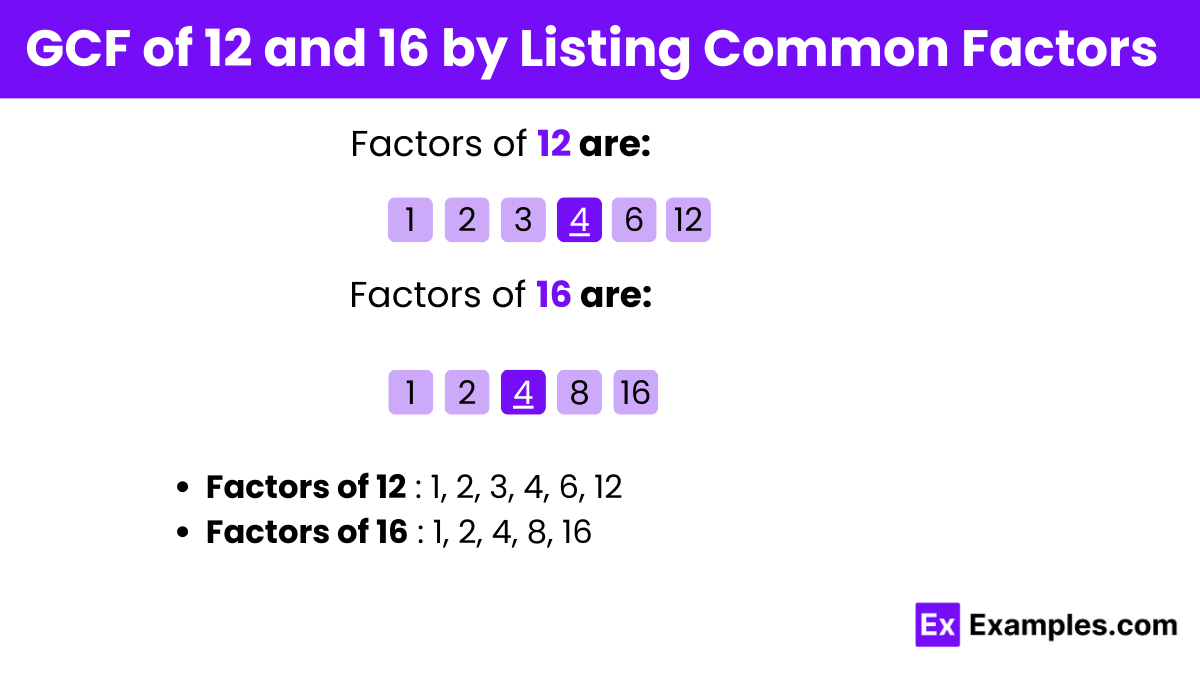
List the factors of each number:
Factors of 12: 1, 2, 3, 4, 6, 12
Factors of 16: 1, 2, 4, 8, 16
Identify the common factors:
Common factors of 12 and 16: 1, 2, 4
Determine the greatest common factor:
The largest common factor is 4.
Therefore, the GCF of 12 and 16 by listing common factors is 4.
The GCF of 12 and 16 is 4.
The GCF is used to simplify fractions by dividing the numerator and the denominator by the GCF.
No, they are not relatively prime because their GCF is 4, not 1.
Yes, the GCF can be one of the numbers if one number is a multiple of the other. However, for 12 and 16, the GCF is 4, which is not one of the numbers.
To verify, check the divisors. Divide both numbers by 4 to see that 4 is the largest number that divides both 12 and 16 without a remainder, confirming the GCF is correct.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the Greatest Common Factor (GCF) of 12 and 16?
2
4
6
8
Which number is a common factor of both 12 and 16?
3
5
4
7
How many factors do 12 and 16 have in common?
2
3
4
5
What is the smallest common factor of 12 and 16?
1
2
4
6
Which number is not a factor of both 12 and 16?
2
4
6
8
If you multiply the GCF of 12 and 16 by 2, what is the result?
6
8
10
12
The GCF of 12 and 16 is a factor of which of the following numbers?
8
12
16
All of the above
What is the GCF of 12, 16, and 24?
2
4
6
12
The sum of the GCF of 12 and 16 and the smallest prime number is:
5
6
7
8
If the GCF of 12 and 16 is divided by 2, what is the result?
1
2
3
4
Before you leave, take our quick quiz to enhance your learning!

