What is the result of multiplying 81 by 4?
324
325
326
327

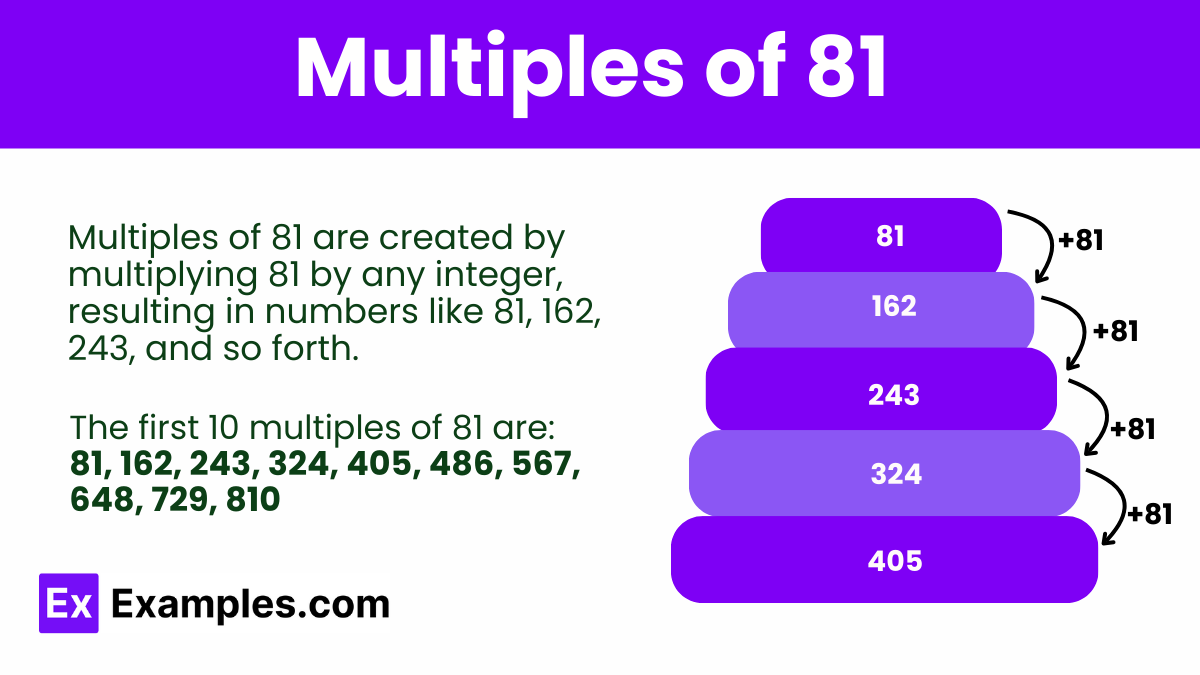
Multiples of 81 are numbers that can be expressed as 81 × n, where n is an integer. These multiples are not necessarily even and follow a pattern, increasing by 81 each time (e.g., 81, 162, 243, 324, 405). Multiples of 81 are crucial in mathematics, especially in algebraic concepts, squares, square roots, and fractions. They play a fundamental role in understanding number properties and performing various arithmetic operations efficiently. Recognizing these multiples aids in grasping more complex mathematical ideas and solving algebraic equations. Multiples serve as essential building blocks in number theory, helping to explore patterns, relationships, and the behavior of numbers within mathematical frameworks. Understanding multiples of 81 enhances mathematical proficiency and problem-solving skills.
Multiples of 81 are numbers that can be expressed as 81×n, where n is an integer. These numbers are always even and include values like 81, 162, 243, 324, and so on.
Prime Factorization of 81: 3 x 3 x 3 x 3
First 10 Multiples of 81 are 81, 162, 243, 324, 405, 486, 567, 648, 729, 810,
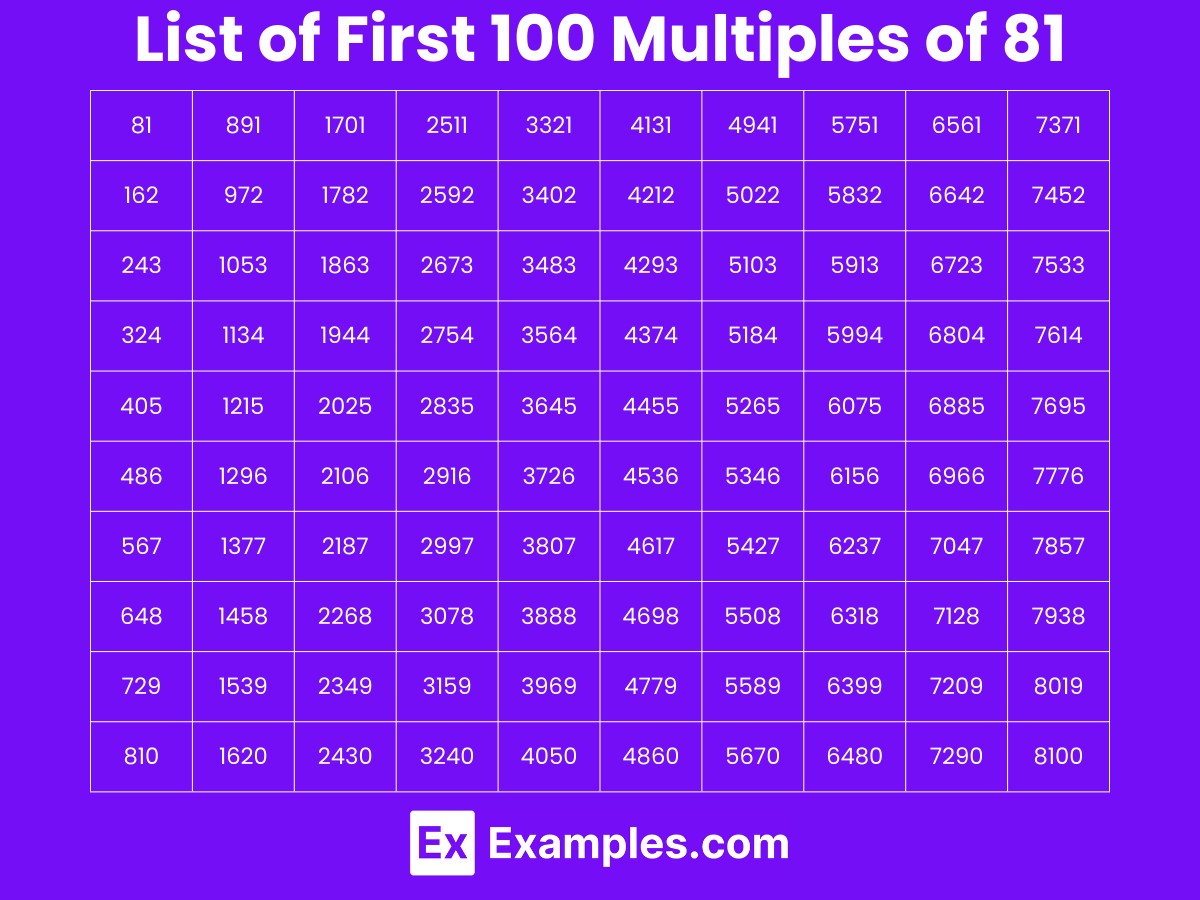
First 50 Multiples of 81 are 81, 162, 243, 324, 405, 486, 567, 648, 729, 810, 891, 972, 1053, 1134, 1215, 1296, 1377, 1458, 1539, 1620, 1701, 1782, 1863, 1944, 2025, 2106, 2187, 2268, 2349, 2430, 2511, 2592, 2673, 2754, 2835, 2916, 2997, 3078, 3159, 3240, 3321, 3402, 3483, 3564, 3645, 3726, 3807, 3888, 3969, 4050
| Number | Reason | Remainder |
|---|---|---|
| 81 | 81 × 1 = 81, which is exactly divisible by 81 | 0 |
| 162 | 81 × 2 = 162, which is exactly divisible by 81 | 0 |
| 243 | 81 × 3 = 243, which is exactly divisible by 81 | 0 |
| 324 | 81 × 4 = 324, which is exactly divisible by 81 | 0 |
| 135 | 135 ÷ 81 is not an integer; 135 is not a multiple of 81 | 54 |
| Number | Reason | Remainder |
|---|---|---|
| 81 | 81 × 1 = 81, which is exactly divisible by 81 | 0 |
| 162 | 81 × 2 = 162, which is exactly divisible by 81 | 0 |
| 243 | 81 × 3 = 243, which is exactly divisible by 81 | 0 |
| 324 | 81 × 4 = 324, which is exactly divisible by 81 | 0 |
| 405 | 81 × 5 = 405, which is exactly divisible by 81 | 0 |
| 486 | 81 × 6 = 486, which is exactly divisible by 81 | 0 |
| 567 | 81 × 7 = 567, which is exactly divisible by 81 | 0 |
| 648 | 81 × 8 = 648, which is exactly divisible by 81 | 0 |
| 729 | 81 × 9 = 729, which is exactly divisible by 81 | 0 |
| 810 | 81 × 10 = 810, which is exactly divisible by 81 | 0 |
| 891 | 81 × 11 = 891, which is exactly divisible by 81 | 0 |
| 972 | 81 × 12 = 972, which is exactly divisible by 81 | 0 |
| 1053 | 81 × 13 = 1053, which is exactly divisible by 81 | 0 |
| 1134 | 81 × 14 = 1134, which is exactly divisible by 81 | 0 |
| 1215 | 81 × 15 = 1215, which is exactly divisible by 81 | 0 |
| 1296 | 81 × 16 = 1296, which is exactly divisible by 81 | 0 |
| 1377 | 81 × 17 = 1377, which is exactly divisible by 81 | 0 |
| 1458 | 81 × 18 = 1458, which is exactly divisible by 81 | 0 |
| 1539 | 81 × 19 = 1539, which is exactly divisible by 81 | 0 |
| 1620 | 81 × 20 = 1620, which is exactly divisible by 81 | 0 |
| 1701 | 81 × 21 = 1701, which is exactly divisible by 81 | 0 |
| 1782 | 81 × 22 = 1782, which is exactly divisible by 81 | 0 |
| 1863 | 81 × 23 = 1863, which is exactly divisible by 81 | 0 |
| 1944 | 81 × 24 = 1944, which is exactly divisible by 81 | 0 |
| 2025 | 81 × 25 = 2025, which is exactly divisible by 81 | 0 |
| 2106 | 81 × 26 = 2106, which is exactly divisible by 81 | 0 |
| 2187 | 81 × 27 = 2187, which is exactly divisible by 81 | 0 |
| 2268 | 81 × 28 = 2268, which is exactly divisible by 81 | 0 |
| 2349 | 81 × 29 = 2349, which is exactly divisible by 81 | 0 |
| 2430 | 81 × 30 = 2430, which is exactly divisible by 81 | 0 |
| 2511 | 81 × 31 = 2511, which is exactly divisible by 81 | 0 |
| 2592 | 81 × 32 = 2592, which is exactly divisible by 81 | 0 |
| 2673 | 81 × 33 = 2673, which is exactly divisible by 81 | 0 |
| 2754 | 81 × 34 = 2754, which is exactly divisible by 81 | 0 |
| 2835 | 81 × 35 = 2835, which is exactly divisible by 81 | 0 |
| 2916 | 81 × 36 = 2916, which is exactly divisible by 81 | 0 |
| 2997 | 81 × 37 = 2997, which is exactly divisible by 81 | 0 |
| 3078 | 81 × 38 = 3078, which is exactly divisible by 81 | 0 |
| 3159 | 81 × 39 = 3159, which is exactly divisible by 81 | 0 |
| 3240 | 81 × 40 = 3240, which is exactly divisible by 81 | 0 |
| 3321 | 81 × 41 = 3321, which is exactly divisible by 81 | 0 |
| 3402 | 81 × 42 = 3402, which is exactly divisible by 81 | 0 |
| 3483 | 81 × 43 = 3483, which is exactly divisible by 81 | 0 |
| 3564 | 81 × 44 = 3564, which is exactly divisible by 81 | 0 |
| 3645 | 81 × 45 = 3645, which is exactly divisible by 81 | 0 |
| 3726 | 81 × 46 = 3726, which is exactly divisible by 81 | 0 |
| 3807 | 81 × 47 = 3807, which is exactly divisible by 81 | 0 |
| 3888 | 81 × 48 = 3888, which is exactly divisible by 81 | 0 |
| 3969 | 81 × 49 = 3969, which is exactly divisible by 81 | 0 |
| 4050 | 81 × 50 = 4050, which is exactly divisible by 81 | 0 |
| 4131 | 81 × 51 = 4131, which is exactly divisible by 81 | 0 |
| 4212 | 81 × 52 = 4212, which is exactly divisible by 81 | 0 |
| 4293 | 81 × 53 = 4293, which is exactly divisible by 81 | 0 |
| 4374 | 81 × 54 = 4374, which is exactly divisible by 81 | 0 |
| 4455 | 81 × 55 = 4455, which is exactly divisible by 81 | 0 |
| 4536 | 81 × 56 = 4536, which is exactly divisible by 81 | 0 |
| 4617 | 81 × 57 = 4617, which is exactly divisible by 81 | 0 |
| 4698 | 81 × 58 = 4698, which is exactly divisible by 81 | 0 |
| 4779 | 81 × 59 = 4779, which is exactly divisible by 81 | 0 |
| 4860 | 81 × 60 = 4860, which is exactly divisible by 81 | 0 |
| 4941 | 81 × 61 = 4941, which is exactly divisible by 81 | 0 |
| 5022 | 81 × 62 = 5022, which is exactly divisible by 81 | 0 |
| 5103 | 81 × 63 = 5103, which is exactly divisible by 81 | 0 |
| 5184 | 81 × 64 = 5184, which is exactly divisible by 81 | 0 |
| 5265 | 81 × 65 = 5265, which is exactly divisible by 81 | 0 |
| 5346 | 81 × 66 = 5346, which is exactly divisible by 81 | 0 |
| 5427 | 81 × 67 = 5427, which is exactly divisible by 81 | 0 |
| 5508 | 81 × 68 = 5508, which is exactly divisible by 81 | 0 |
| 5589 | 81 × 69 = 5589, which is exactly divisible by 81 | 0 |
| 5670 | 81 × 70 = 5670, which is exactly divisible by 81 | 0 |
| 5751 | 81 × 71 = 5751, which is exactly divisible by 81 | 0 |
| 5832 | 81 × 72 = 5832, which is exactly divisible by 81 | 0 |
| 5913 | 81 × 73 = 5913, which is exactly divisible by 81 | 0 |
| 5994 | 81 × 74 = 5994, which is exactly divisible by 81 | 0 |
| 6075 | 81 × 75 = 6075, which is exactly divisible by 81 | 0 |
| 6156 | 81 × 76 = 6156, which is exactly divisible by 81 | 0 |
| 6237 | 81 × 77 = 6237, which is exactly divisible by 81 | 0 |
| 6318 | 81 × 78 = 6318, which is exactly divisible by 81 | 0 |
| 6399 | 81 × 79 = 6399, which is exactly divisible by 81 | 0 |
| 6480 | 81 × 80 = 6480, which is exactly divisible by 81 | 0 |
| 6561 | 81 × 81 = 6561, which is exactly divisible by 81 | 0 |
| 6642 | 81 × 82 = 6642, which is exactly divisible by 81 | 0 |
| 6723 | 81 × 83 = 6723, which is exactly divisible by 81 | 0 |
| 6804 | 81 × 84 = 6804, which is exactly divisible by 81 | 0 |
| 6885 | 81 × 85 = 6885, which is exactly divisible by 81 | 0 |
| 6966 | 81 × 86 = 6966, which is exactly divisible by 81 | 0 |
| 7047 | 81 × 87 = 7047, which is exactly divisible by 81 | 0 |
| 7128 | 81 × 88 = 7128, which is exactly divisible by 81 | 0 |
| 7209 | 81 × 89 = 7209, which is exactly divisible by 81 | 0 |
| 7290 | 81 × 90 = 7290, which is exactly divisible by 81 | 0 |
| 7371 | 81 × 91 = 7371, which is exactly divisible by 81 | 0 |
| 7452 | 81 × 92 = 7452, which is exactly divisible by 81 | 0 |
| 7533 | 81 × 93 = 7533, which is exactly divisible by 81 | 0 |
| 7614 | 81 × 94 = 7614, which is exactly divisible by 81 | 0 |
| 7695 | 81 × 95 = 7695, which is exactly divisible by 81 | 0 |
| 7776 | 81 × 96 = 7776, which is exactly divisible by 81 | 0 |
| 7857 | 81 × 97 = 7857, which is exactly divisible by 81 | 0 |
| 7938 | 81 × 98 = 7938, which is exactly divisible by 81 | 0 |
| 8019 | 81 × 99 = 8019, which is exactly divisible by 81 | 0 |
| 8100 | 81 × 100 = 8100, which is exactly divisible by 81 | 0 |
Odd Numbers: All multiples of 81 are odd numbers, meaning they end in 1, 3, 5, 7, or 9.
Divisibility: A number is a multiple of 81 if it can be divided by 81 with no remainder.
Factors: Multiples of 81 have 81 as one of their factors.
Infinite Sequence: There are infinitely many multiples of 81, extending indefinitely as 81, 162, 243, 324, 405, and so on.
Arithmetic Pattern: The difference between consecutive multiples of 81 is always 81.
81: 81×1 = 81
162: 81×2 = 162
243: 81×3 = 243
810: 81×10 = 810
1620: 81×20 = 1620
2430: 81×30 = 2430
Time: 81 hours in three days is a multiple of 81 because 81×1=81.
Money: $81 is a multiple of 81 because 81×1=81.
Measurements: 243 inches in 20.25 feet is a multiple of 81 because 81×3=243.
Counting by Eighty-Ones: When counting by eighty-ones (81, 162, 243, 324…), you are listing the multiples of 81.
Odd Numbers: Any number that is a multiple of 81, such as 162 or 243, is a multiple of 81 because it can be divided evenly by 81.
Multiples of 81 are numbers that can be expressed as 81 times an integer (e.g., 81, 162, 243, etc.).
To find multiples of 81, multiply 81 by any integer (e.g., 81×1=81, 81×2=162).
Yes, 81 is a multiple of itself because 81×1=81.
Yes, all multiples of 81 are odd numbers since 81 is an odd number.
The smallest multiple of 81 is 81 itself.
Yes, 162 is a multiple of 81 because 81×2=162.
There are infinitely many multiples of 81.
The 10th multiple of 81 is 810 (81×10=810).
Yes, multiples of 81 are also multiples of 9 because 81 is a multiple of 9.
To find multiples of 87, multiply 87 by integers (e.g., 87 × 1 = 87, 87 × 2 = 174, etc.).
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the result of multiplying 81 by 4?
324
325
326
327
Which of the following is not a multiple of 81?
162
243
324
500
What is the least multiple of 81 greater than 500?
486
567
648
729
Which number is the seventh multiple of 81?
567
648
729
810
What is the difference between the third and sixth multiples of 81?
162
243
324
405
What is the sum of the first three multiples of 81?
486
243
324
648
Which multiple of 81 is equal to 9 times 10?
729
810
900
972
What is the greatest multiple of 81 less than 1000?
729
810
972
1000
If you subtract 81 from 648, which multiple of 81 do you get?
486
567
729
810
Which of these numbers is the tenth multiple of 81?
810
891
972
1080
Before you leave, take our quick quiz to enhance your learning!

