Square Root Tricks
Square Root Tricks
Mastering square root tricks can significantly enhance your mathematical efficiency, especially when dealing with both rational and irrational numbers in algebraic calculations. Understanding square roots simplifies operations involving perfect squares, integers, and helps solve equations swiftly. The least square method in statistics leverages square root calculations to minimize error. Additionally, working with square and square roots aids in grasping relationships in complex numbers. Recognizing these patterns improves mental maths and numerical accuracy in various mathematical disciplines.
Definition of Square Root
Tricks to Calculate Square Root
- Prime Factorization: Break down the number into its prime factors. Pair the same prime factors together, and take one from each pair. Multiply these together to find the square root.
- Estimation Method: Find the closest perfect squares around the number, then estimate based on the number’s position relative to those perfect squares. Adjust your estimate using division.
- Long Division Method: Use the long division method to find the square root. Divide the number into pairs of digits starting from the decimal point, then find the largest number that, when squared, is less than or equal to each pair.
- Approximation with Decimals: Use decimal approximations for numbers that aren’t perfect squares. For example, knowing 2=1.414= and 3=1.732 helps with quick calculations.
- Use of Square Root Tables: For historical or practical contexts, refer to square root tables that provide pre-calculated square roots for various numbers, allowing for quick look-ups of values.
Square Root Trick for 4 Digit Numbers
- To estimate the square root of a 4-digit number, start by pairing the digits from right to left. Refer to a chart that links the unit digits of perfect squares to possible unit digits of their roots. Use the first pair of digits to determine which two consecutive perfect squares this value falls between, establishing a possible range for the tens digit of the square root. For instance, if 𝑛 is between 𝑎² and 𝑏², then 𝑎 is the tens digit of the desired square root. Specifically, the digits 5 and 10 have unique properties, as their squares do not repeat, making their identification easier in this process.
- If the unit digit is not 5 or 10, further steps are required. Multiply the potential tens digit options 𝑎a and 𝑏. If 𝑎𝑏ab is less than or equal to 𝑛, select 𝑏; otherwise, select 𝑎a. This method helps approximate the square root by narrowing down the possible values efficiently.
Example
- Divide the Number into Two-Digit Pairs: Break the number into pairs starting from the right. For example, for 5625, consider 56 and 25 as two-digit pairs.
- Estimate the First Digit: Find the closest square root of the first two-digit pair. For 56, 7² = 49 is close, so estimate 7.
- Find the Second Digit: Subtract 7² from 56, leaving 7. Bring down the next pair 25 to get 725.
- Double the First Digit and Find the Approximate Second Digit: Double 7 to get 14. Find a digit 𝑥 such that 14𝑥×𝑥 is less than or equal to 725. 145×5 = 725, so the second digit is 55.
- Combine to Get the Square Root: The approximate square root of 5625 is 75, which is exact in this case because 75×75 = 5625.
Square Root Trick for 5 Digit Numbers
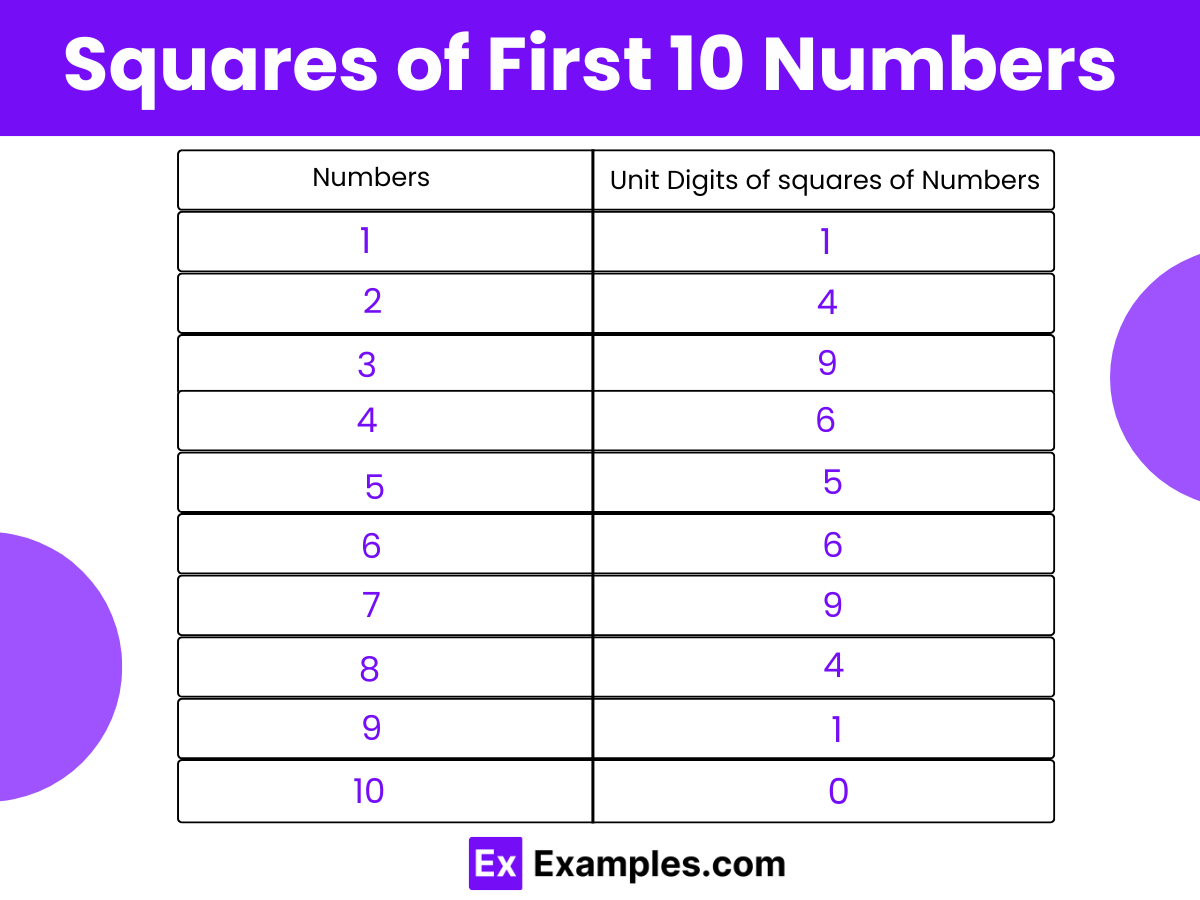
To estimate the square root of a 5-digit number, begin by pairing the digits, starting from right to left. Then, consult a chart that matches the unit digits of numbers with the possible unit digits of their square roots to determine possible values. Analyze the group of the first three digits, called 𝑛, and identify the two perfect squares between which 𝑛n lies (√𝑎²<𝑛<√𝑏²). This process helps establish that 𝑎<𝑛<𝑏, so 𝑎a will be the tens digit of the square root.
There are only two numbers whose squares do not repeat—5 and 10—and their unique properties should be used in determining the unit digit. If the unit digit identified in Step 2 is 5 or 10, that value is used directly. If not, multiply the digits 𝑎 and 𝑏. If 𝑎𝑏≤𝑛, choose 𝑏 as the units digit; otherwise, choose 𝑎. This structured approach helps estimate the square root by narrowing down potential values using logic and patterns in the squares of numbers.
Example
- Estimate the First Digit: Determine the nearest perfect square that is less than or equal to the first two or three digits of the number. For example, for 23409, consider 234. The nearest perfect square is 15² = 2251 So, the estimate for the first digit is 15.
- Subtract and Form New Number: Subtract 22500 (which is 15²×100) from 23409. This leaves 909.
- Divide and Find Second Digit: Double 15 to get 30. Add a digit 𝑥 to 30 and find 𝑥 such that (30𝑥×𝑥) is less than or equal to 909. Here, 303×3 = 909, so the next digit is 3.
- Combine Digits: The approximate square root of 23409 is 153.
More About on Square Root
Examples for Square Root Tricks
Example 1: 15225
- Pair the Digits:
Group the digits from right to left: 15 and 225. - Match the Unit Digit:
The number ends with 5, so the possible unit digit of its square root is also 5. - Consider the First Group:
Take the first group of three digits, which is 15. - Determine the Range of Squares:
1515 lies between the squares 9 (from 3² = 9) and 1616 (from 4² = 16), so the tens digit is 3. - Unique Unit Digits:
Since the unit digit is 5, it’s directly taken from Step 2. - Estimated Square Root:
The square root is 35 because 35²=1225
Example 2: 21316
- Pair the Digits:
Group the digits: 21 and 316. - Match the Unit Digit:
The number ends with 6, so possible unit digits are 4 or 6. - Consider the First Group:
Take the first group of three digits, 21. - Determine the Range of Squares:
21 lies between the squares 16 (from 4² = 16) and 25 (from 5² = 25), so the tens digit is 4. - Find Units Digit:
Multiply 4×5 = 20, which is less than or equal to 21, so the unit digit is 6.Estimated Square Root:
The square root is 46.
FAQs
Why do we pair the digits from right to left when estimating square roots?
Pairing the digits from right to left helps in visualizing and separating the whole number and fractional parts of the number, which is crucial for accurately estimating square roots. It allows you to work with the digits in manageable chunks.
How does matching the unit digit with the chart help in estimating the square root?
Matching the unit digit with a square root chart helps narrow down possible values for the square root’s unit digit. The chart leverages patterns in squares of numbers, making it easier to find potential values without calculations.
Why do we focus on the first group of digits when estimating square roots?
The first group of digits is crucial because it determines the primary range in which the square root lies. Identifying this range provides the tens digit and establishes the general magnitude of the square root.
How do we determine the tens digit of the square root?
To find the tens digit, identify the two perfect squares between which the first group of digits lies. The lower square’s root represents the tens digit, as it is closest to the number but still less than or equal to it.
Why are 5 and 10 unique when estimating square roots?
The numbers 5 and 10 are unique because their squares are 25 and 100, respectively. This makes their unit digits easy to identify directly, without further calculation, simplifying the estimation process.
Share :
