Square & Square Root of 441
Square & Square Root of 441
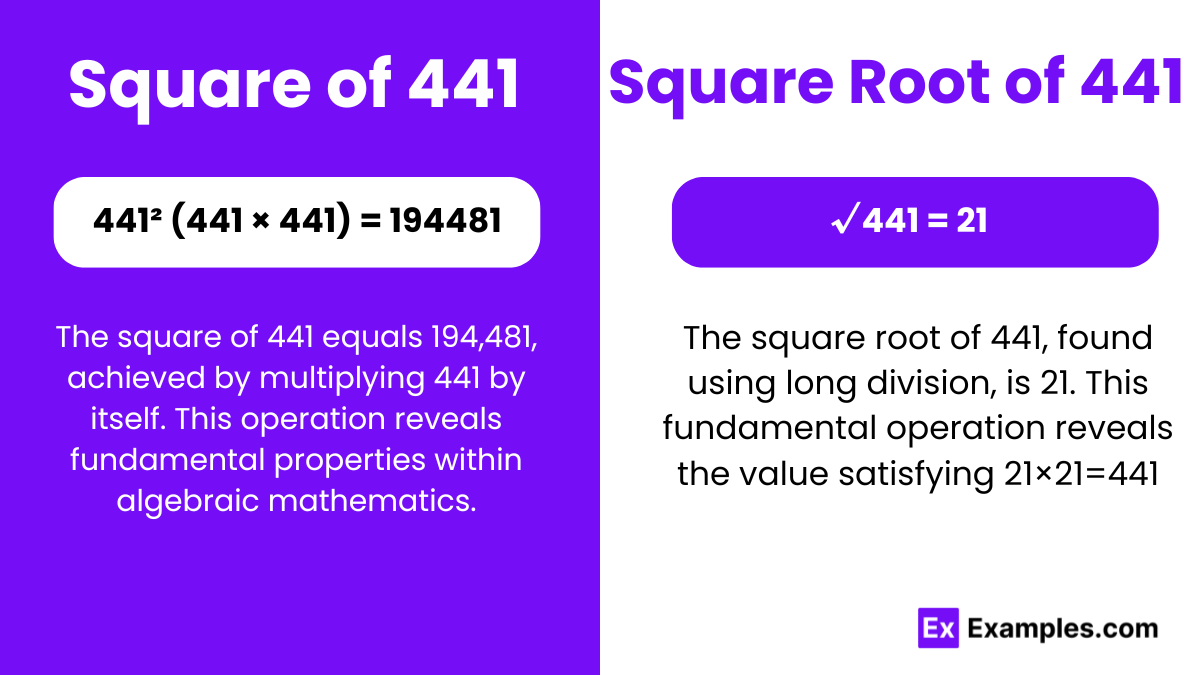
Within the realm of algebraic mathematics, squares and square roots are pivotal concepts. Squaring a number, like 441, involves multiplying it by itself, yielding 194481. This operation is foundational, crucial in exploring rational and irrational numbers. Understanding these basics enriches comprehension of mathematical relationships and patterns. Squares reveal inherent properties of numbers, while square roots unravel complex numerical mysteries. These concepts illuminate mathematical landscapes, guiding explorations into fractional territories. Mastery of squares and square roots empowers mathematicians to navigate diverse mathematical terrains, uncovering the elegance and complexity woven within algebraic frameworks.
Square of 441
The square number 441 equals 194,481, derived by multiplying 441 by itself, a fundamental operation in algebraic mathematics, revealing inherent properties of numbers.
Square Root of 441
The square root of 441 is 21. This fundamental operation in mathematics uncovers the value that, when multiplied by itself, equals 441.
Square Root of 441: 21
Exponential Form: 441^½ or 441^0.5
Radical Form: √441
Is the Square Root of 441 Rational or Irrational?
Yes, the square root of 441 is a rational number
Rational Numbers: Rational numbers are those that can be expressed as the quotient of two integers, where the denominator is not zero. In other words, they can be written as fractions of the form a/b where ( a ) and ( b ) are integers and ( b ≠ 0). Rational numbers include integers and fractions. Examples of rational numbers include 1/2, -3 and 5/7
Irrational Numbers: Irrational numbers cannot be expressed as fractions of integers. Their decimal representations are non-repeating and non-terminating. These numbers cannot be represented as finite or repeating decimals. Examples of irrational numbers include the square roots of non-perfect squares like √2, √3, and √5 , as well as transcendental numbers like 𝜋
In essence, rational numbers can be expressed as fractions with finite or repeating decimals, whereas irrational numbers cannot be represented in this way, having non-repeating, non-terminating decimal expansions.
Methods to Find Value of Root 441
There are several methods to find the value of the square root of 441:
Prime Factorization Method: Break down 441 into its prime factors, which are 3 × 3 × 7 × 7. Since the square root of 441 is 21, it’s the product of the prime factors in pairs: (3 × 7) = 21.
Long Division Method: Use the long division algorithm to find the square root of 441 by approximation. Start with a guess (e.g., 20) and refine iteratively.
Using a Calculator: Most calculators have a square root function. Simply input 441 and find the square root directly.
Estimation: Since 441 is close to the perfect square of 400 (20 × 20) and 484 (22 × 22), you can estimate that its square root is likely between 20 and 22, closer to 21.
Choose the method that suits your preference or the tools available to you.
Square Root of 441 by Long Division Method
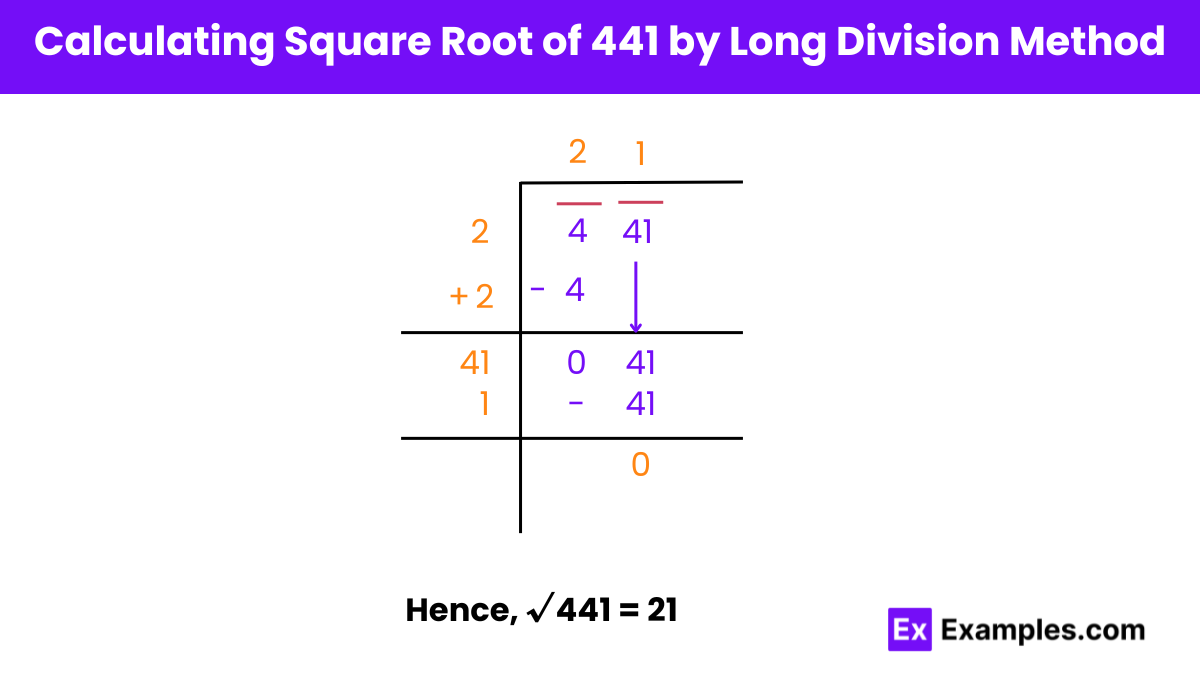
Finding the Square Root of 441 by Long Division
Step 1: Setting Up
Write the number 441. Pair the digits from the right end. In this case, pair 41 under one bar and 4 under the second bar.
Step 2: Initial Estimation
Find a number that, when multiplied by itself, results in a number equal to or less than 4.
Step 3: Iterative Calculation
Multiply the quotient obtained in the previous step by 2 (or add it with itself) and write it as the new divisor’s ten’s place digit. Then, bring down the next pair of numbers (in this case, 41).
Step 4: Refinement
Choose a number for the unit’s place of the divisor in such a way that when it is multiplied with the new divisor, it results in 41 or a number closest to 41.
Conclusion: Obtaining the Square Root
The value of the square root of 441, obtained by the long division method, is 21.
Is 441 Perfect Square root or Not
Yes, the number 441 is a perfect square
Yes, 441 is a perfect square because it can be expressed as the square of an integer, specifically (21 \times 21), which equals 441. Therefore, the square root of 441 is a whole number (21), making it a perfect square.
FAQ’S
What is the significance of the square root of 441?
The square root of 441 is important in mathematics as it represents the side length of a square with an area of 441 square units.
What are some real-life applications of the square root of 441?
In geometry, the square root of 441 can represent the side length of a square garden or plot of land measuring 441 square units.
Is the square root of 441 rational or irrational?
The square root of 441 is rational because it can be expressed as the ratio of two integers, specifically 21/1.
Share :
