Average Speed Formula
Average Speed Formula
What is Average Speed Formula?
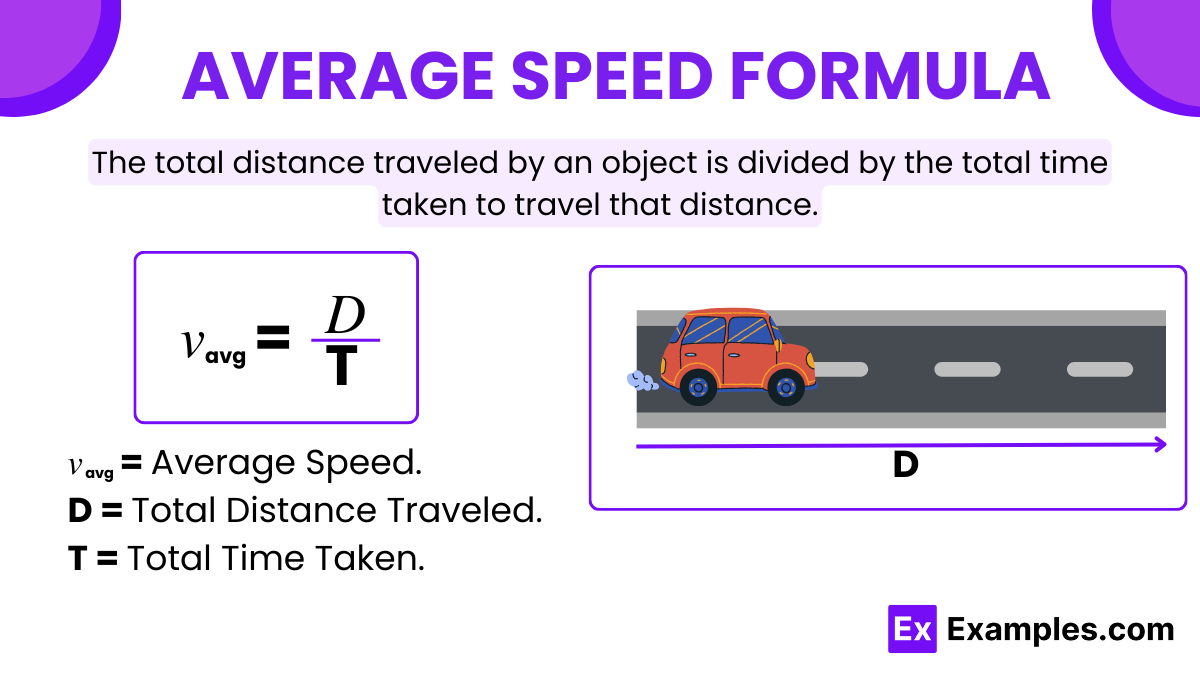
The concept of average speed is a fundamental aspect of physics, providing a clear measure of how fast an object moves over a distance during a given period. Essentially, the average speed formula helps us calculate the total distance traveled divided by the total time taken to travel that distance. Mathematically, it is expressed as:
- 𝑣ₐᵥ₉ = Average Speed.
- D = Total Distance Traveled.
- T = Total Time Taken
This formula is used extensively in various physics calculations and real-world applications, such as in calculating travel times, in sports to measure speeds, or in traffic management.
The development of the concept of average speed dates back to the early days of classical mechanics. While the specific individual who first defined the average speed formula isn’t clearly recorded in the annals of history, it was during the Renaissance period that significant contributions were made by scientists such as Galileo Galilei. His experiments and rigorous documentation laid the groundwork for what would eventually be encapsulated in the laws of motion by Sir Isaac Newton. Galileo’s systematic observations of motion helped articulate the idea that the average speed of an object could be determined by simple measurements of distance and time.
Derivation Of Average Speed Formula
Define the total distance and total time: Consider an object travels different distances 𝑑₁, 𝑑₂, 𝑑₃, …, 𝑑ₙ over corresponding time intervals t₁, t₂, t₃, …, tₙ. The total distance traveled, 𝐷, is the sum of all individual distances:
Sum up the total time: Similarly, the total time taken, 𝑇, is the sum of all time intervals:
Apply the average speed formula: The average speed ( 𝑣ₐᵥ₉ ) is then calculated by dividing the total distance by the total time:
Average Speed with Two Speeds
Step 1: Identify the Speeds and Corresponding Time Intervals
First, determine the two speeds at which the object travels, 𝑣₁ and 𝑣₂, and the respective time intervals for each speed, 𝑡₁ and 𝑡₂. These values are essential for the calculation.
Step 2: Calculate the Distances for Each Speed
Next, calculate the distance traveled at each speed. This is done by multiplying each speed by its corresponding time interval:
- Distance traveled at speed 𝑣₁ is 𝑑₁ = 𝑣₁ × 𝑡₁.
- Distance traveled at speed 𝑣₂ is 𝑑₂ = 𝑣₂ × 𝑡₂.
Step 3: Sum the Total Distance and Total Time
Add together the distances traveled during each interval to find the total distance,𝐷:
𝐷 = 𝑑₁ + 𝑑₂
Similarly, add the time intervals to find the total time, 𝑇:
𝑇 = 𝑡₁ + 𝑡₂
Step 4: Calculate the Average Speed
Finally, compute the average speed by dividing the total distance by the total time:
𝑣ₐᵥ₉ = 𝐷 / 𝑇
Applications of Average Speed Formula
- Travel Planning: Travelers use the average speed formula to estimate travel times for road trips, helping them plan their departures and arrivals effectively.
- Athletics: Coaches calculate the average speed of athletes during races or training sessions to assess performance improvements and set training targets.
- Academics: Teachers incorporate the average speed formula in physics and mathematics curriculums to teach students about motion, speed, and distance relationships.
- Traffic Management: Urban planners and traffic engineers apply the average speed formula to analyze traffic flow, optimize signal timings, and improve road safety.
- Logistics: Logistics companies estimate delivery times by calculating the average speed of vehicles over delivery routes, enhancing efficiency and customer satisfaction.
- Law Enforcement: Police use the average speed formula to determine whether drivers are adhering to speed limits, especially in areas with average speed cameras.
- Science Experiments: Students and researchers use the average speed formula to analyze results in experiments involving motion, such as those studying the behavior of different animals or mechanical objects.
Example Problems of Average Speed Formula
Problem 1: Road Trip Calculation
Question: Sarah drives to work every morning. She drives 15 miles at 30 mph to get out of her neighborhood and then 45 miles at 60 mph on the highway. What is her average speed for the entire trip?
Solution:
Calculate the time for each part of the trip:
Neighborhood: 𝑡₁ = 15 miles / 30 mph = 0.5 hours
Highway: 𝑡₂ = 45 miles / 60 mph = 0.75 hours
Calculate the total time and distance:
Total time 𝑇 = 0.5 + 0.75 = 1.25 hours
Total distance 𝐷 = 15 + 45 = 60 miles
Apply the average speed formula:
𝑣ₐᵥ₉ = 60 miles / 1.25 hours = 48 mph
Answer: Sarah’s average speed for her entire trip is 48 mph.
Problem 2: Runner’s Pace
Question: A runner completes the first half of a 10 km race in 25 minutes and the second half in 35 minutes. What is the runner’s average speed for the race?
Solution:
Convert minutes to hours for ease of calculation:
Total time 𝑇 = ( 25 + 35 ) / 60=1 hour
The total distance 𝐷 is 10 km.
Calculate the average speed:
𝑣ₐᵥ₉ = 10 km / 1 hour=10 km/h
Answer: The runner’s average speed is 10 km/h.
Problem 3: Commuting Time
Question: Alex commutes to school by cycling 2 miles uphill at an average speed of 10 mph, then 2 miles downhill at an average speed of 20 mph. What is his average speed for the entire 4-mile trip?
Solution:
Calculate the time taken for each part of the journey:
Uphill: 𝑡₁=2 miles / 10 mph = 0.2 hours
Downhill: 𝑡₂ = 2 miles / 20 mph = 0.1 hours
Total time 𝑇 = 0.2 + 0.1 = 0.3 hours
Total distance 𝐷 = 2 + 2 = 4 miles
Calculate the average speed:
𝑣ₐᵥ₉ = 4 miles / 0.3 hours ≈13.33 mph
Answer: Alex’s average speed for his commute is approximately 13.33 mph.
FAQs
How to Find Average Speed Without Distance and Time?
Without distance and time, average speed can be estimated using other variables like RPM and gear ratios in mechanical systems.
What is the Average Constant Speed Formula?
The average constant speed formula is 𝑣ₐᵥ₉ = Total Distance / Total Time, essential for consistent speed calculations.
Why is Speed Calculated as an Average?
We calculate speed as an average to simplify understanding motion over varying speeds and distances, providing a clear overall performance measure.
Share :
