Continuity Equation

The continuity equation is a fundamental principle in fluid mechanics, expressing the conservation of mass in a fluid system. It states that the rate at which mass enters a system is equal to the rate at which it leaves, ensuring mass is neither created nor destroyed. Mathematically, for an incompressible fluid, it is represented as ( A₁V₁ = A₂V₂ ), where ( A ) is the cross-sectional area and ( v ) is the fluid velocity. This equation is crucial for analyzing fluid flow in various applications, from pipelines to air ducts, ensuring efficient and predictable fluid transport.

What is the Continuity Equation?

The continuity equation is a mathematical expression of the conservation of mass principle in fluid dynamics. It states that for any incompressible fluid, the mass flow rate must remain constant from one cross-section of a pipe or channel to another. In its simplest form for an incompressible fluid, it is written as:

( A₁v₁ = A₂v₂ )

where ( A₁ ) and ( A₂ ) are the cross-sectional areas of the fluid at points 1 and 2, respectively, and ( v₁ ) and ( v₂ ) are the fluid velocities at these points. For a compressible fluid, the equation is more complex and accounts for changes in fluid density:

∂t/∂ρ​ + ∇ ⋅ (ρv)=0

where ( p ) is the fluid density, ( t ) is time, and ( v ) is the velocity vector field of the fluid. The continuity equation ensures that mass is neither created nor destroyed within the flow system.

Continuity Principle 

The continuity principle, also known as the principle of mass conservation, is a fundamental concept in fluid dynamics that states the mass of fluid entering a system must equal the mass of fluid leaving the system, provided there is no accumulation of mass within the system. This principle ensures that mass is conserved over time within a fluid flow.

For an incompressible fluid, the principle can be expressed using the continuity equation:

[ A₁ V₁ = A₂ V₂ ]

where:

• ( A₁ ) and ( A₂ ) are the cross-sectional areas at two different points in the flow.
• ( V₁ ) and ( V₂ ) are the fluid velocities at these respective points.

This equation indicates that if the cross-sectional area of the flow decreases, the fluid velocity must increase, and vice versa, to maintain a constant mass flow rate.

For a compressible fluid, the continuity principle is expressed as:

∂t/∂ρ​ + ∇ ⋅ (ρv)=0

where:

• ( ρ ) is the fluid density.
• ( t ) is time.
• ( v ) is the velocity vector field of the fluid.
• ( ∇ ) represents the divergence operator, which measures the rate of change of density within the flow.

The continuity principle is crucial in engineering and physics for designing and analyzing systems involving fluid flow, such as pipelines, air ducts, and natural streams.

Integral Form

The integral form of the continuity equation is a way to express the conservation of mass for a fluid within a given region. Think of it as ensuring that the amount of fluid inside a balloon stays constant unless it flows in or out through the balloon’s surface.

Here’s the simplified idea:

1. Control Volume: Imagine a fixed region in space (like a balloon or a box) where fluid can flow in and out. This is called the control volume.
2. Mass Inside the Volume: The total mass of fluid inside this volume can change over time.
3. Flow Across the Surface: Fluid can enter or leave the control volume through its surface.

The continuity equation says that the rate at which the mass inside the volume changes plus the rate at which mass is flowing out must equal zero. In simple terms, this means:

• If more fluid enters the volume than leaves, the amount of fluid inside increases.
• If more fluid leaves the volume than enters, the amount of fluid inside decreases.
• If the same amount enters and leaves, the amount of fluid inside stays the same.

Mathematically, this is written as:

d/dt∫VρdV+∫Sρv⋅ndA=0

where:

• ( d/dt∫VρdV ) is the total mass inside the control volume.
• ( ∫Sρv⋅ndA=0 ) is the net flow of mass across the surface of the control volume.

For an incompressible fluid (where density ρ is constant), this simplifies to saying that the fluid entering and leaving the control volume must be equal, ensuring mass is conserved.

Flow Rate Formula

The basic formula for flow rate (𝑄Q) is:

𝑄=𝐴×𝑣Q=A×v

where:

• 𝑄Q is the flow rate.
• 𝐴A is the cross-sectional area of the pipe or channel.
• 𝑣v is the fluid velocity (how fast the fluid is moving).

Breaking it Down

1. Cross-Sectional Area (𝐴A):
   • This is the size of the opening through which the fluid is flowing.
   • For a circular pipe, it’s the area of the circle: 𝐴=𝜋𝑟2A=πr2, where 𝑟r is the radius of the pipe.
2. Fluid Velocity (𝑣v):
   • This is how fast the fluid is moving through the pipe.
   • It’s usually measured in meters per second (m/s) or feet per second (ft/s).

Fluid Dynamics

Fluid dynamics is the study of how fluids (liquids and gases) move and the forces acting on them. It encompasses concepts like laminar and turbulent flow, the continuity equation for mass conservation, and Bernoulli’s principle for pressure and velocity relationships. Navier-Stokes equations describe the motion of viscous fluids, and the Reynolds number predicts flow regimes. Applications include designing aircraft, pipelines, weather forecasting, and understanding ocean currents and blood flow. Fluid dynamics is crucial in engineering, meteorology, oceanography, and medicine for optimizing systems and predicting fluid behavior.

Uses of the Continuity Equation

Engineering : The continuity equation is essential in designing pipelines and hydraulic systems, ensuring consistent fluid flow rates and optimizing efficiency for water, oil, gas, and hydraulic devices like lifts and brakes.

Aerospace : In aerospace, the continuity equation aids in designing aircraft wings and airfoils for smooth airflow, optimizing lift and fuel efficiency, and ensuring proper flow rates in rocket propulsion systems.

Environmental Science : The continuity equation is used to predict and manage water flow in rivers and streams, aiding in flood prevention, habitat conservation, and monitoring pollutant spread in water bodies and the atmosphere.

Medical Field : In the medical field, the continuity equation analyzes blood flow in arteries and veins for diagnosing cardiovascular diseases and ensures consistent airflow in respiratory devices like ventilators.

Industrial Processes : Chemical plants use the continuity equation to manage fluid flow in reactors and pipelines, ensuring proper mixing and reaction rates, while cooling systems in power plants and refrigeration units optimize coolant flow.

Construction and Infrastructure : The continuity equation is crucial for designing efficient drainage and sewage systems to handle varying water flow rates and for ensuring uniform water distribution in agricultural irrigation systems.

Automotive Engineering : In automotive engineering, the continuity equation maintains consistent fuel and air flow in internal combustion engines for optimal performance and efficiency and manages coolant flow to prevent engine overheating.

Maritime Engineering : Maritime engineering uses the continuity equation to ensure stable fluid flow around ship hulls for better maneuverability and fuel efficiency, and to maintain consistent internal and external fluid flow for submarine operations.

Continuity Equation Examples

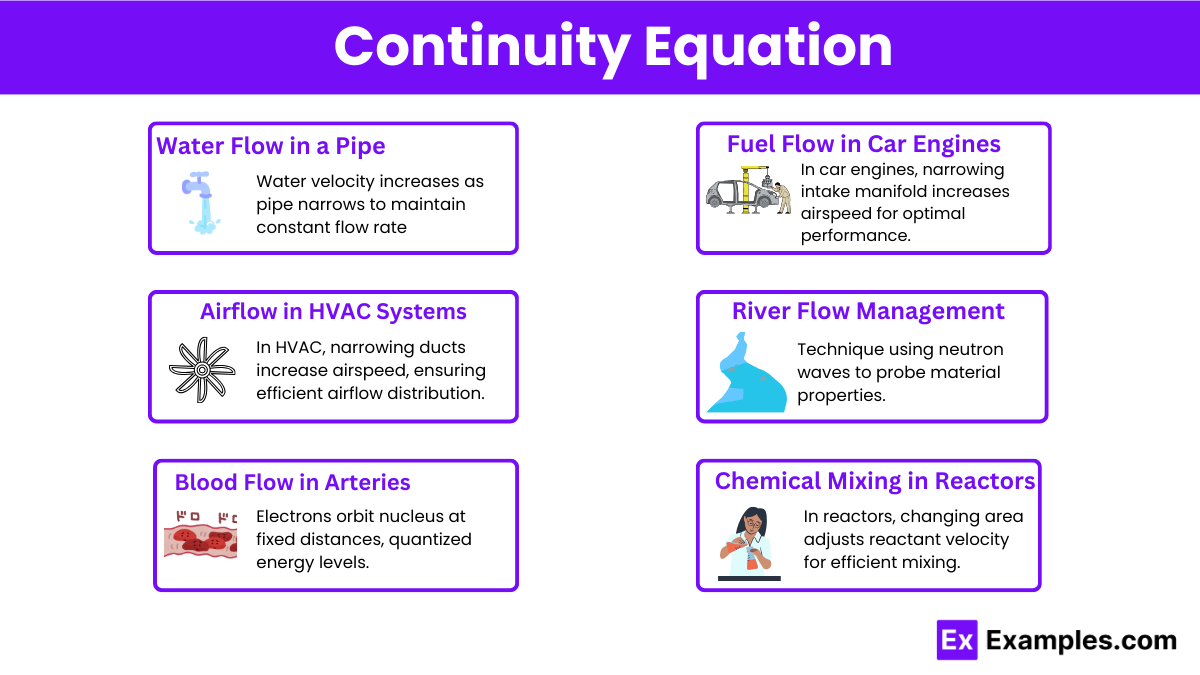
Water Flow in a Pipe : Consider water flowing through a pipe that narrows from a larger diameter to a smaller diameter. The continuity equation ensures that the flow rate remains constant. As the pipe narrows, the velocity of the water increases to maintain the same flow rate, illustrating how the cross-sectional area and velocity are inversely related.

Airflow in HVAC Systems : In HVAC systems, the continuity equation helps design ducts and vents to ensure consistent airflow throughout a building. For example, if a duct narrows, the airspeed increases to maintain the same volume of air, ensuring efficient heating and cooling distribution.

Blood Flow in Arteries : The continuity equation applies to blood flow in the human body. As blood vessels branch into smaller arteries, the velocity of the blood must increase to maintain a constant flow rate. This principle helps in understanding and diagnosing various cardiovascular conditions.

Fuel Flow in Car Engines : In car engines, the continuity equation ensures that the fuel-air mixture flows consistently through the engine’s intake manifold. As the manifold narrows, the airspeed increases, ensuring proper mixing and combustion for optimal engine performance.

River Flow Management : In river management, the continuity equation predicts how changes in the riverbed affect water flow. If a river narrows, the water velocity increases to maintain the same flow rate, helping manage flood risks and water distribution for agriculture.

Chemical Mixing in Reactors : In chemical reactors, the continuity equation ensures that reactants flow consistently through the reactor. As the cross-sectional area of the reactor changes, the velocity of the reactants adjusts to maintain a constant flow rate, ensuring efficient mixing and reaction rates.

Oil Transport in Pipelines : For oil transport, the continuity equation is crucial in designing pipelines. When the pipeline diameter decreases, the oil flow velocity must increase to maintain a steady flow rate, ensuring efficient transport from production sites to refineries.

Cooling Systems in Electronics : In cooling systems for electronics, the continuity equation ensures that coolant flows consistently through narrow channels. As the channels narrow, the coolant velocity increases, maintaining a constant flow rate to effectively dissipate heat and prevent overheating.