Stress Formula

What is Stress Formula?

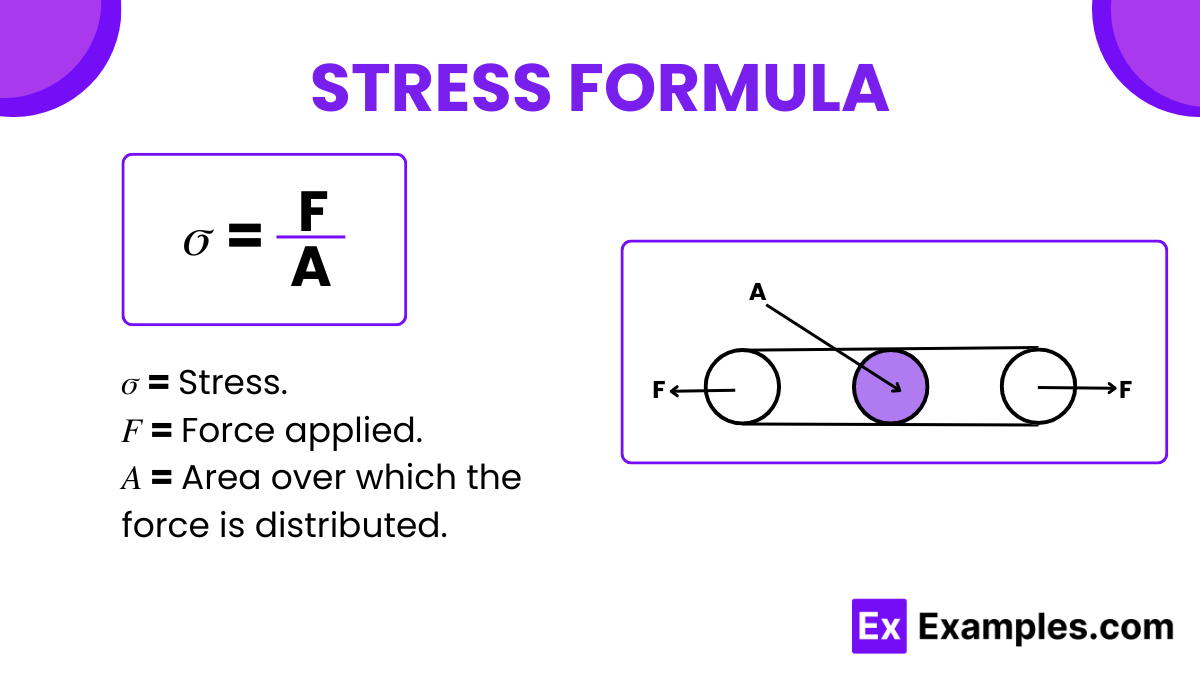
The stress formula in physics quantifies the amount of internal force exerted per unit area within a material. This formula is essential for understanding how materials deform under various forces, a fundamental concept in the field of physics and engineering. we express the formula for stress as

𝜎 = 𝐹 / 𝐴

• 𝜎 represents the stress.
• 𝐹 is the force applied.
• 𝐴 is the area over which the force is distributed.

This concept was developed by Thomas Young, an English polymath, who first described it in the early 19th century. Young’s work laid the foundation for modern material science, particularly in understanding elasticity and structural integrity. The stress formula helps engineers and physicists predict how materials will behave when subjected to different forces, which is critical in designing safe and effective structures, from bridges to airplanes.

Applications of Stress Formula

1. Building and Construction: Engineers calculate the stress on structural components like beams and columns to ensure they can support expected loads without failure.
2. Material Science: Researchers use the stress formula to determine the breaking point of different materials, aiding in the development of stronger and more durable materials.
3. Manufacturing: In industries such as automotive and aerospace manufacturing, the stress formula helps in designing parts that must withstand high forces without deforming.
4. Safety Testing: Companies perform stress tests on products to simulate how they will perform under extreme conditions, ensuring they are safe for public use.
5. Education and Training: Physics educators use the stress formula to teach students about the properties of materials and the principles of force and pressure.

Example Problems on Stress Formula

Problem 1: Calculating Stress in a Beam

Question: A force of 5000 Newtons is applied to a rectangular beam with a cross-sectional area of 0.1 square meters. Calculate the stress on the beam.

Solution: 𝜎 = 𝐹 / 𝐴 = 5000 N / 0.1 m² = 50000 Pa

The stress on the beam is 50000 Pascals.

Problem 2: Stress on a Wire

Question: A thin steel wire with a diameter of 0.5 mm is subjected to a tension force of 300 Newtons. Calculate the stress experienced by the wire.

Solution: First, calculate the cross-sectional area 𝐴 of the wire using the formula for the area of a circle, 𝐴=𝜋𝑟², Where r is the radius.

𝑟=0.5 mm / 2 =0.25 mm=0.00025 m

𝐴 = 𝜋(0.00025 m)² ≈ 1.963×10⁻⁷ m²

Now, apply the stress formula:

𝜎 = 𝐹𝐴 = 300 N / 1.963×10⁻⁷  m² ≈ 1.528 × 10⁹ Pa

The stress on the wire is approximately 1.528 GigaPascals (GPa).

Problem 3: Comparing Stress in Two Columns

Question: Two columns, one with a circular cross-section of radius 10 cm and another with a square cross-section of side 20 cm, each bear a load of 10000 Newtons. Which column experiences more stress?

Solution: Calculate the area for each column:

Circular column area 𝐴₁ = 𝜋𝑟²

𝑟=0.1 m

𝐴₁ = 𝜋(0.1 m)² = 0.0314 m²

Square column area 𝐴₂ = 𝑠²

𝑠 = 0.2 m

𝐴₂ = (0.2 m)² = 0.04 m²

Now, calculate the stress for each column:

Circular column: 𝜎₁ = 10000 N / 0.0314 m² ≈ 318471 Pa

Square column: 𝜎₂ = 10000 N / 0.04 m²=250000 Pa

The circular column experiences more stress than the square column because it has a smaller cross-sectional area.

FAQs

What is Stress and Its Unit?

Stress measures internal force per unit area within materials, quantified in Pascals (Pa).

What is the Formula for Total Stress?

To calculate total stress, use the formula 𝜎=𝐹𝐴​, where 𝐹 represents the force and 𝐴 represents the area.

How Do You Calculate the Stress?

Calculate stress by dividing the force applied (𝐹) by the area (A): 𝜎=𝐹𝐴​.