Factors of 14

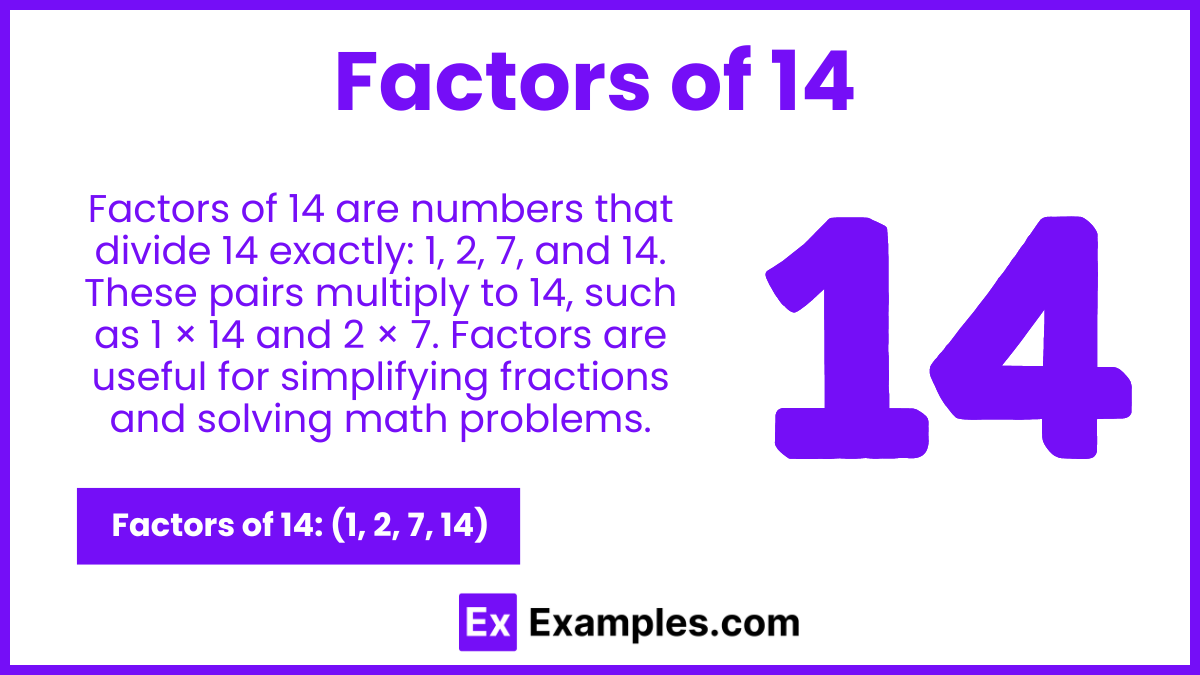
The factors of 14 are 1, 2, 7, and 14. These are the numbers that can divide 14 without leaving a remainder. To determine these factors, start with 1 and the number itself, then check the integers in between. Since 14 is the product of the prime numbers 2 and 7, its prime factorization is 2 × 7. Knowing the factors of a number is essential for simplifying fractions, finding greatest common divisors, and solving problems related to divisibility. This basic understanding helps in various mathematical calculations and applications.

What are the Factors of 14?

The factors of 14 are the numbers that can be multiplied together to get the product of 14, and they include 1, 2, 7, and 14. These numbers divide 14 without leaving any remainder. To find the factors, start with 1 and the number itself, then check the integers in between. The number 14 is a product of the prime numbers 2 and 7, giving it a prime factorization of 2 × 7. Understanding the factors of a number is crucial for various mathematical concepts such as simplifying fractions, finding greatest common divisors, and solving problems related to divisibility.

Factor Pairs of 14

• 1 and 14: Multiplying 1 by 14 equals 14. This pair shows the most basic factorization of 14, illustrating how every number is divisible by 1 and itself.
  
• 2 and 7: Multiplying 2 by 7 equals 14. These numbers are also prime factors of 14, demonstrating that 14 is a composite number made up of these two primes.

Understanding these factor pairs is useful for breaking down numbers in multiplication and division, simplifying fractions, and solving various mathematical problems. Factor pairs are essential in determining least common multiples, greatest common divisors, and in the process of factoring algebraic expressions. This knowledge aids in a deeper comprehension of number properties and their relationships.

How to Calculate Prime Factors of 14

Calculating the prime factors of a number involves breaking down the number into its basic building blocks, which are prime numbers. Prime factors are prime numbers that, when multiplied together, give the original number. Here is a step-by-step guide to finding the prime factors of 14.

Step 1: Understand Prime Numbers

Prime numbers are numbers greater than 1 that have no divisors other than 1 and themselves. Examples include 2, 3, 5, 7, 11, etc.

Step 2: Start with the Smallest Prime Number

Begin with the smallest prime number, which is 2. Check if 14 is divisible by 2.

14 ÷ 2 = 7

Since 14 is divisible by 2, 2 is a prime factor.

Step 3: Divide by the Next Smallest Prime Number

After dividing 14 by 2, you get 7. Now, check if 7 is a prime number.

7 is a prime number because it is only divisible by 1 and 7.

Step 4: List All Prime Factors

Since 7 is a prime number and no further division is needed, the prime factors of 14 are:

2 and 7

Factors of 14 : Examples

Example 1: Finding All Factors of 14

To find all factors of 14, list all numbers that divide 14 without leaving a remainder.

1. Start with 1: 14 ÷ 1 = 14
2. Next is 2: 14 ÷ 2 = 7
3. Check 3: 14 ÷ 3 ≠ whole number
4. Check 4: 14 ÷ 4 ≠ whole number
5. Check 5: 14 ÷ 5 ≠ whole number
6. Check 6: 14 ÷ 6 ≠ whole number
7. Next is 7: 14 ÷ 7 = 2

Thus, the factors of 14 are: 1, 2, 7, and 14.

Example 2: Prime Factorization of 14

Prime factorization involves expressing 14 as a product of its prime factors.

1. Start with 2 (smallest prime number): 14 ÷ 2 = 7
2. Check 7 (next prime number): 7 is already a prime number.

So, the prime factorization of 14 is: 2 × 7.

Example 3: Factor Pairs of 14

Factor pairs are two numbers that, when multiplied, give the original number (14).

1. 1 and 14: 1 × 14 = 14
2. 2 and 7: 2 × 7 = 14

Thus, the factor pairs of 14 are: (1, 14) and (2, 7).

Example 4: Finding Common Factors

If you want to find common factors of 14 and another number, such as 28, list the factors of both numbers and find the common ones.

• 1. Factors of 14: 1, 2, 7, 14
• 2. Factors of 28: 1, 2, 4, 7, 14, 28

Common factors are: 1, 2, 7, and 14.

Example 5: Greatest Common Factor (GCF)

To find the GCF of 14 and another number, say 21, list the factors of both and identify the greatest one.

• 1. Factors of 14: 1, 2, 7, 14
• 2. Factors of 21: 1, 3, 7, 21

Factors of 14 : Tips

Understanding how to find and work with the factors of 14 can be made easier with some useful tips. These tips will help you quickly determine the factors and utilize them effectively in different mathematical scenarios.

1. Start by listing the number 1 and the number itself (14) as the first and last factors.
2. Check divisibility by 2, since 14 is an even number. 2 is a factor, and dividing 14 by 2 gives another factor, which is 7.
3. Remember that prime numbers only have two factors: 1 and the number itself. Check if the resulting quotient is a prime number.
4. Factor pairs can help in identifying all factors quickly. For 14, the pairs are (1, 14) and (2, 7).
5. Use prime factorization to express 14 as a product of prime numbers. For 14, the prime factors are 2 and 7.
6. When finding common factors with another number, list all factors of both numbers and identify the shared ones.
7. To find the greatest common factor (GCF) with another number, determine the highest factor that both numbers share.
8. Use division to verify factors. If a number divides 14 evenly (without a remainder), it is a factor.
9. Recognize that factors come in pairs. For any factor ‘a’ of 14, there exists another factor ‘b’ such that a × b = 14.
10. Practice with different numbers to become familiar with the process of finding factors, which can improve your speed and accuracy.

What is the greatest common factor (GCF) of 14?

The greatest common factor (GCF) of 14 and another number is the largest number that divides both numbers without leaving a remainder. For example, the GCF of 14 and 21 is 7.

How do you use the prime factorization method to find the factors of 14?

To use the prime factorization method to find the factors of 14:

1. Express 14 as a product of its prime factors: 14 = 2 × 7.
2. Use these prime factors to find all combinations of factors: 1, 2, 7, and 14.

What is the sum of all factors of 14?

The sum of all factors of 14 is 1 + 2 + 7 + 14 = 24.

Can 14 have an infinite number of factors?

No, 14 cannot have an infinite number of factors. The factors of any number are finite. For 14, the factors are specifically 1, 2, 7, and 14.

Is 4 a multiple as well as a factor of 14? True or false?

False. 4 is neither a multiple nor a factor of 14. The factors of 14 are 1, 2, 7, and 14. The multiples of 14 include numbers like 14, 28, 42, and so on.

What are the multiples of 14?

The multiples of 14 are the numbers obtained by multiplying 14 with integers. Some of the multiples of 14 include 14, 28, 42, 56, 70, 84, 98, 112, 126, and 140. These numbers are all divisible by 14 without leaving a remainder.