The factors of 18 are the numbers that divide 18 exactly without leaving a remainder. These factors are fundamental in mathematics, playing a crucial role in simplifying fractions, solving equations, and determining common multiples. Knowing the factors of a number like 18 helps in various mathematical operations and real-world applications, such as problem-solving and analytical tasks. Understanding these factors can also aid in recognizing patterns and relationships between numbers, making mathematical concepts easier to grasp and apply. This article explores the factors of 18, how to determine them, and their importance in different contexts.
What are the Factors of 18?
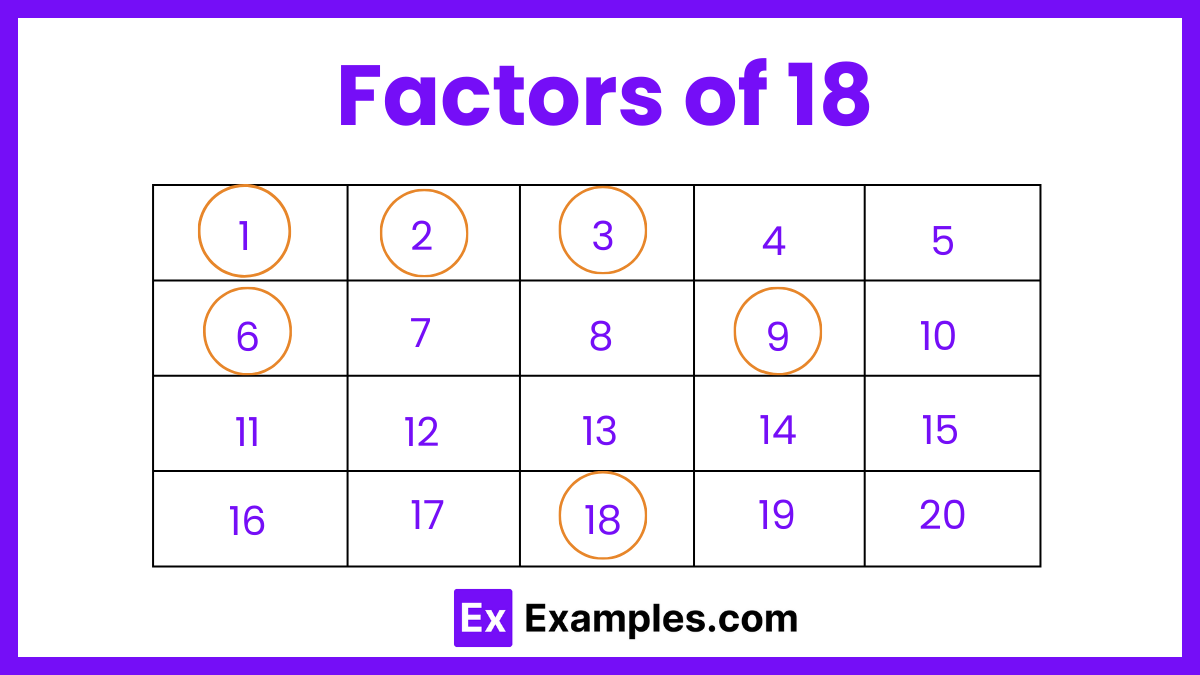
The factors of 18 are the numbers that can divide 18 without leaving a remainder. These include 1, 2, 3, 6, 9, and 18. Identifying these factors is essential in simplifying mathematical problems, finding common multiples, and solving equations. Understanding the factors of 18 helps in various applications, making mathematical concepts easier to apply in real-world scenarios.
Factors Pairs of 18
Understanding factor pairs is essential in breaking down a number into its components. Factor pairs of 18 are sets of two numbers that, when multiplied together, equal 18. These pairs are useful in various mathematical problems, including simplifying equations, finding common denominators, and solving algebraic expressions.
- 1 and 18: Multiplying 1 by 18 gives 18, making 1 and 18 a factor pair. This pair is straightforward, demonstrating that any number multiplied by 1 remains unchanged.
- 2 and 9: The product of 2 and 9 is 18, so 2 and 9 are factor pairs of 18. This pair shows how the number 18 can be broken down into smaller components.
- 3 and 6: When 3 is multiplied by 6, the result is 18, making 3 and 6 a factor pair. This pair is often encountered in basic multiplication and division problems.
How to Calculate Factors of 18?
Calculating the factors of 18 involves finding all the numbers that divide 18 exactly without leaving a remainder. This process is straightforward and can be done by following a few simple steps. Understanding how to find factors is essential in various mathematical tasks, such as simplifying fractions, solving equations, and analyzing number patterns.
Step 1: Start with 1 and 18
Begin by noting that 1 and the number itself (18) are always factors. So, 1 and 18 are the first factor pair.
Step 2: Check for Divisibility by 2
Next, check if 18 is divisible by 2. Since 18 is an even number, it is divisible by 2. This gives the factor pair 2 and 9 (because 18 ÷ 2 = 9).
Step 3: Check for Divisibility by 3
Check if 18 is divisible by 3. Since the sum of the digits of 18 (1 + 8 = 9) is divisible by 3, 18 is also divisible by 3. This results in the factor pair 3 and 6 (because 18 ÷ 3 = 6).
Step 4: Continue Checking Up to the Square Root
Continue this process up to the square root of 18 (approximately 4.24). For each number, check if it divides 18 exactly. In this case, 4 does not divide 18 evenly, so no further factor pairs are found.
Factors of 18 : Examples
Example 1: Verifying 2 as a Factor
To check if 2 is a factor of 18, divide 18 by 2. 18÷2=918÷2=9 Since 9 is a whole number, 2 is a factor of 18. The factor pair here is (2, 9).
Example 2: Verifying 3 as a Factor
To verify if 3 is a factor of 18, divide 18 by 3. 18÷3=618÷3=6 Since 6 is a whole number, 3 is a factor of 18. The factor pair here is (3, 6).
Example 3: Verifying 6 as a Factor
To check if 6 is a factor of 18, divide 18 by 6. 18÷6=318÷6=3 Since 3 is a whole number, 6 is a factor of 18. The factor pair here is (6, 3).
Example 4: Verifying 9 as a Factor
To determine if 9 is a factor of 18, divide 18 by 9. 18÷9=218÷9=2 Since 2 is a whole number, 9 is a factor of 18. The factor pair here is (9, 2).
Example 5: Verifying 1 as a Factor
To verify if 1 is a factor of 18, divide 18 by 1. 18÷1=1818÷1=18 Since 18 is a whole number, 1 is a factor of 18. The factor pair here is (1, 18).
Factors of 18 : Tips
Calculating the factors of 18 can be made easier with a few helpful tips. These tips can streamline the process and ensure accuracy when identifying all the factors.
- Always begin by noting that 1 and the number itself (18) are factors. These are universal factors for any number.
- Start by testing the divisibility of 18 by smaller numbers like 2, 3, 4, and so on. This helps in quickly identifying common factors.
- For each potential factor, divide 18 by that number. If the result is a whole number, both the divisor and the quotient are factors of 18.
- To quickly check if 18 is divisible by 3, add the digits (1 + 8 = 9). Since 9 is divisible by 3, 18 is also divisible by 3.
- You only need to check divisibility up to the square root of 18 (approximately 4.24). Beyond this point, factor pairs will start to repeat in reverse order.
What are the prime factors of 18?
The prime factors of 18 are the prime numbers that multiply together to give 18. The prime factorization of 18 is 2 x 3 x 3 or 2×32.
Is 18 a composite number?
Yes, 18 is a composite number because it has more than two factors.
How do factors of 18 relate to multiples of 18?
Factors of 18 are the numbers that can divide 18 without leaving a remainder, whereas multiples of 18 are the numbers that result from multiplying 18 by any integer (e.g., 18, 36, 54, etc.).
What is the greatest common factor (GCF) of 18 and another number?
The greatest common factor of 18 and another number is the largest factor that both numbers share. For example, the GCF of 18 and 24 is 6.
What is the sum of the factors of 18?
The sum of the factors of 18 (1, 2, 3, 6, 9, 18) is 1 + 2 + 3 + 6 + 9 + 18 = 39.
What are the positive and negative pair factors of 18?
The positive pair factors of 18 are (1, 18), (2, 9), and (3, 6). The negative pair factors are (-1, -18), (-2, -9), and (-3, -6). Both positive and negative pairs, when multiplied together, yield the product 18, showcasing how factors can include both positive and negative integers.



